Chapter: 11th Physics : UNIT 5 : Motion of System of Particles and Rigid Bodies
Work done by Torque
Work done by Torque
Let us consider a rigid body rotating about a fixed axis. Figure 5.29 shows a point P on the body rotating about an axis perpendicular to the plane of the page. A tangential force F is applied on the body.
It produces a small displacement ds on the body. The work done (dw) by the force is,

dw = Fds
As the distance ds, the angle of rotation dθ and radius r are related by the expression,
ds = rdθ
The expression for work done now becomes,
dw = F ds; dw = F r dθ
The term (Fr) is the torque τ produced by the force on the body.
dw = τdθ (5.51)

This expression gives the work done by the external torque τ, which acts on the body rotating about a fixed axis through an angle dθ.
The corresponding expression for work done in translational motion is,
dw = Fds
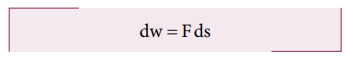
Related Topics