Solved Example Problems for Center of Mass

Solved Example Problems for Center of Mass of Two Point Masses

Example 5.1

Two point masses 3 kg and 5 kg are at 4 m and 8 m from the origin on X-axis. Locate the position of center of mass of the two point masses (i) from the origin and (ii) from 3 kg mass.

Solution

Let us take, m1 = 3 kg and m2= 5 kg

(i) To find center of mass from the origin:

The point masses are at positions, x1 = 4 m, x2 = 8 m from the origin along X axis.

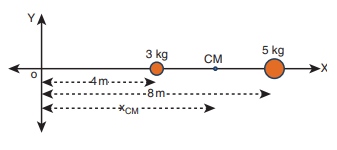
The center of mass xCM can be obtained using equation 5.4.

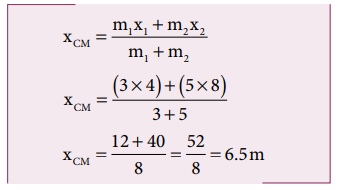
The center of mass is located 6.5 m from the origin on X-axis.

(ii) To find the center of mass from 3 kg mass:

The origin is shifted to 3 kg mass along X-axis. The position of 3 kg point mass is zero (x1 = 0) and the position of 5 kg point mass is 4 m from the shifted origin (x2 = 4 m).

The center of mass is located 2.5 m from 3 kg point mass, (and 1.5 m from the 5 kg point mass) on X-axis.

This result shows that the center of mass is located closer to larger mass. 

If the origin is shifted to the center of mass, then the principle of moments holds good. 

m1x1=m2x2; 3x2.5=5x1.5;7.5=7.5

When we compare case (i) with case (ii), the xCM = 2.5m from 3 kg mass could also be obtained by subtracting 4 m (the position of 3 kg mass) from 6.5 m, where the center of mass was located in case (i)

Example 5.2

From a uniform disc of radius R, a small disc of radius R/2 is cut and removed as shown in the diagram. Find the center of mass of the remaining portion of the disc.

Solution

Let us consider the mass of the uncut full disc be M. Its center of mass would be at the geometric center of the disc on which the origin coincides.

Let the mass of the small disc cut and removed be m and its center of mass is at a position R/2 to the right of the origin as shown in the figure.

Hence, the remaining portion of the disc should have its center of mass to the left of the origin; say, at a distance x. We can write from the principle of moments,

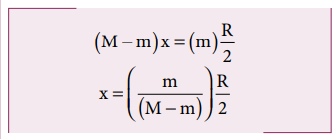
If σ is the surface mass density (i.e. mass per unit surface area), σ=M/πR2; then, the mass m of small disc is,

The center of mass of the remaining portion is at a distance R/6 to the left from the center of the disc.

If, the small disc is removed concentrically from the large disc, what will be the position of the center of mass of the remaining portion of disc? 

Example 5.3

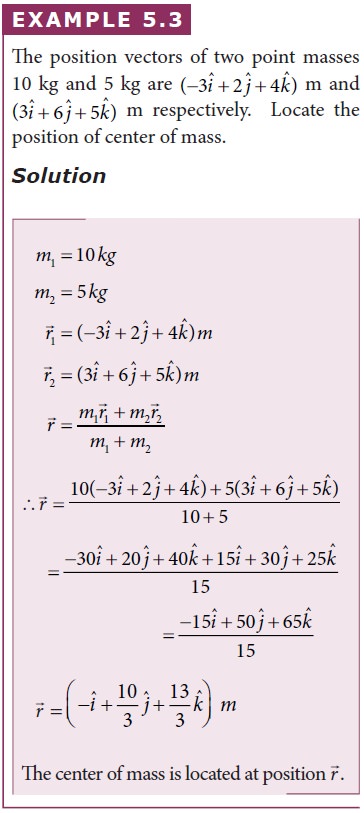
Solved Example Problems for Center of mass for uniform distribution of mass

Example 5.4

Locate the center of mass of a uniform rod of mass M and length l.

Solution

Consider a uniform rod of mass M and length whose one end coincides with the origin as shown in Figure. The rod is kept along the x axis. To find the center of mass

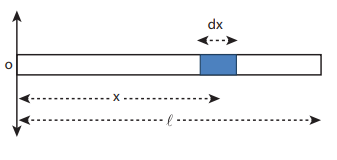
of this rod, we choose an infinitesimally small mass dm of elemental length dx at a distance x from the origin.

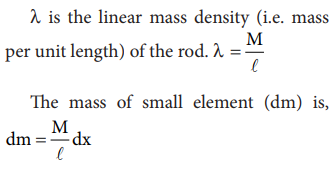
Now, we can write the center of mass equation for this mass distribution as,

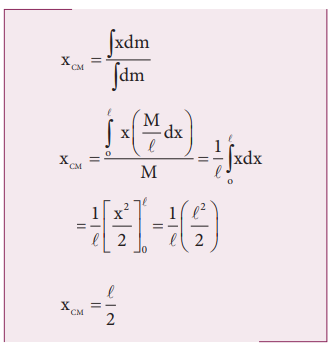
As the position l/2 is the geometric center of the rod, it is concluded that the center of mass of the uniform rod is located at its geometric center itself.

Solved Example Problems for Motion of Center of Mass

Example 5.5

A man of mass 50 kg is standing at one end of a boat of mass 300 kg floating on still water. He walks towards the other end of the boat with a constant velocity of 2 ms-1  with respect to a stationary observer on land. What will be the velocity of the boat, (a) with respect to the stationary observer on land? (b) with respect to the man walking in the boat?

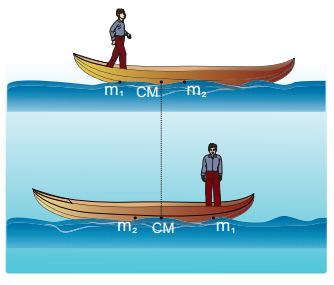
[Given: There is friction between the man and the boat and no friction between the boat and water.]

Solution

Mass of the man (m1) is, m1= 50 kg

Mass of the boat (m2) is, m2 = 300 kg

With respect to a stationary observer:

The man moves with a velocity, v1 = 2 m s-1 and the boat moves with a velocity v2 (which is to be found)

(i) To determine the velocity of the boat with respect to a stationary observer on land:

As there is no external force acting on the system, the man and boat move due to the friction, which is an internal force in the boat-man system. Hence, the velocity of the center of mass is zero (vCM = 0).

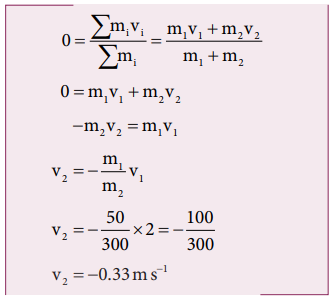
The negative sign in the answer implies that the boat moves in a direction opposite to that of the walking man on the boat to a stationary observer on land.

(ii) To determine the velocity of the boat with respect to the walking man:

We can find the relative velocity as,

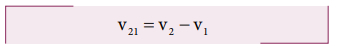
where, v21 is the relative velocity of the boat with respect to the walking man.

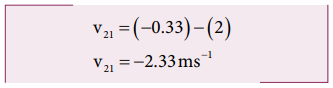
The negative sign in the answer implies that the boat appears to move in the opposite direction to the man walking in the boat.

The magnitude of the relative velocity of the boat with respect to the walking man is greater than the magnitude of the relative velocity of the boat with respect to the stationary observer. 

The negative signs in the two answers indicate the opposite direction of the boat with respect to the stationary observer and the walking man on the boat.

Example 5.6

A projectile of mass 5 kg, in its course of motion explodes on its own into two fragments. One fragment of mass 3 kg falls at three fourth of the range R of the projectile. Where will the other fragment fall?

Solution

It is an explosion of its own without any external influence. After the explosion, the center of mass of the projectile will continue to complete the parabolic path even though the fragments are not following the same parabolic path. After the fragments have fallen on the ground, the center of mass rests at a distance R (the range) from the point of projection as shown in the diagram.

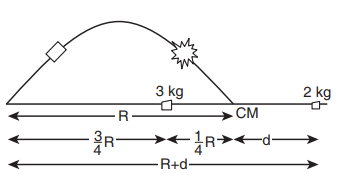
If the origin is fixed to the final position of the center of mass, the principle of moments holds good.

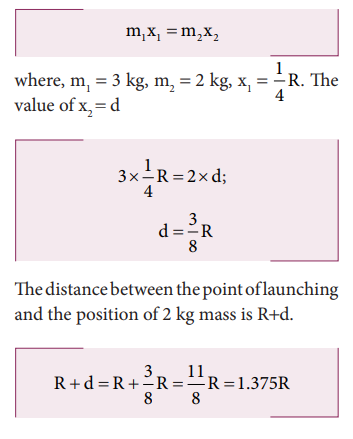
The other fragment falls at a distance of 1.375R from the point of launching. (Here R is the range of the projectile.)