Motion of System of Particles and Rigid Bodies: Important Questions

Short Answer Questions

1. Define center of mass.

2. Find out the center of mass for the given geometrical structures.

a) Equilateral triangle b) Cylinder c) Square

3. Define torque and mention its unit.

4. What are the conditions in which force can not produce torque?

5. Give any two examples of torque in day-to-day life.

6. What is the relation between torque and angular momentum?

7. What is equilibrium?

8. How do you distinguish between stable and unstable equilibrium?

9. Define couple.

10. State principle of moments.

11. Define center of gravity.

12. Mention any two physical significance of moment of inertia

13. What is radius of gyration?

14. State conservation of angular momentum.

15. What are the rotational equivalents for the physical quantities, (i) mass and (ii) force?

16. What is the condition for pure rolling?

17. What is the difference between sliding and slipping?

Long Answer Questions

1. Explain the types of equilibrium with suitable examples.

2. Explain the method to find the center of gravity of a irregularly shaped lamina.

3. Explain why a cyclist bends while negotiating a curve road? Arrive at the expression for angle of bending for a given velocity.

4. Derive the expression for moment of inertia of a rod about its center and perpendicular to the rod.

5. Derive the expression for moment of inertia of a uniform ring about an axis passing through the center and perpendicular to the plane.

6. Derive the expression for moment of inertia of a uniform disc about an axis passing through the center and perpendicular to the plane.

7. Discuss conservation of angular momentum with example.

8. State and prove parallel axis theorem.

9. State and prove perpendicular axis theorem.

10. Discuss rolling on inclined plane and arrive at the expression for the acceleration.

Numerical Problems

1. A uniform disc of mass 100g has a diameter of 10 cm. Calculate the total energy of the disc when rolling along a horizontal table with a velocity of 20 cms-1. (take the surface of table as reference)

Answer

Mass of the disc m = 100 g = 0.1 kg.

Diameter of the disc d = 10 cm

Radius of the disc r = 5 cm = 0.05m

Rolling with a velocity v = 20 cms-1 = 0.20 ms-1

Total energy of the disc ETot = ?

ETot = Translational K.E. + rotational K.E.

Moment of inertia (M.I) of the disc about its own axis

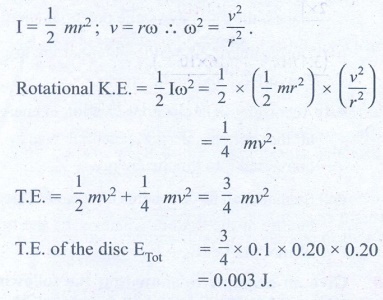
Ans: 0.1028 J

2. A particle of mass 5 units is moving with a uniform speed of v = 3√2 units in the XOY plane along the line y = x + 4. Find the magnitude of angular momentum.

Answer

Equation of line x - y + 4 = 0

Mass of particle = 5 units

Speed v - 3 √2 units

Distance of line from origin r = 

Angel momentum L = mvr = 5 × 3 √2 × 4/√2 = 60 units

Ans: 60 units

3. A fly wheel rotates with a uniform angular acceleration. If its angular velocity increases from 20π rad/s to 40π rad/s in 10 seconds. Find the number of rotations in that period.

Answer:

Initial Angular Velocity ω0 = 20π rad s-1

Final Angular velocity ω = 40 π rad s-1

time taken, t  = 10 s

No. of rotations / s = ?

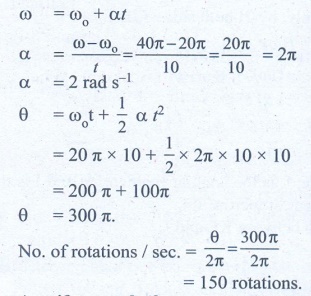
= 150 rotations.

Ans: 150 rotations

4. A uniform rod of mass m and length l makes a constant angle θ with an axis of rotation which passes through one end of the rod. Find the moment of inertia about this axis.

Answer

θ - angle that rod makes with the axis (vertical)

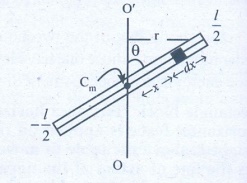
Linear mass density λ = m/l

Consider dx. the part of rod

Mass dx, the part of rod dm=λ.dx

dm= m/l dx. The M.I of the rod about OO'

I = ∫ r2 dm

Where is the perpendicular distance from the elementary mass dm.

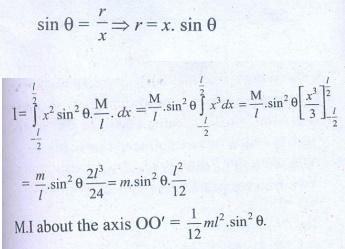
M.I about the axis OO' = 1/12 ml2.sin2 θ.

Ans: 1/12  Ml 2 sin2 θ

5. Two particles P and Q of mass 1kg and 3 kg respectively start moving towards each other from rest under mutual attraction. What is the velocity of their center of mass?

Answer

Mass of particle P m1 = 1 kg.

Mass of particle Q m2 = 3 kg

Velocity of particle P = velocity of particle Q

but in opposite direction.

velocity of center of mass vcm = ?

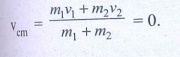
Ans: Zero

6. Find the moment of inertia of a hydrogen molecule about an axis passing through its center of mass and perpendicular to the inter-atomic axis. Given: mass of hydrogen atom 1.7 × 10-27 kg and inter atomic distance is equal to 4 × 10-10 m.

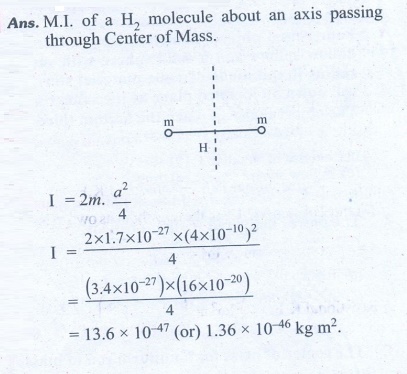
Ans: 1.36 × 10-46 kg m2

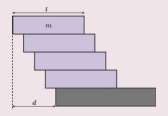
7. On the edge of a wall, we build a brick tower that only holds because of the bricks’ own weight. Our goal is to build a stable tower whose overhang d is greater than the length l of a single brick. What is the minimum number of bricks you need?

(Hint: Find the center of mass for each brick and add.)

8. The 747 boing plane is landing at a speed of 70m s-1. Before touching the ground, the wheels are not rotating. How long a skid mark do the wing wheels leave (assume their mass is 100 kg which is distributed uniformly, radius is 0.7 m, and the coefficient of friction with the ground is 0.5)?

Ans: 2.1 m

Conceptual Questions

1. When a tree is cut, the cut is made on the side facing the direction in which the tree is required to fall. Why?

Answer:

The weight of tree exerts a torque about the point where the cut is made. This causes rotation of the tree about the cut.

2. Why does a porter bend forward while carrying a sack of rice on his back?

Answer:

A porter bends forward while carrying a sack of rice on his back because to change the position of centre of gravity such that he gets the stability.

3. Why is it much easier to balance a meter scale on your finger tip than balancing on a match stick?

Answer

Meter scale is longer and larger than a match stick. Meter scale’s Centre of gravity is higher but match stick has Centre of gravity much lower as compared to scale. Higher the centre of gravity easier it is to balance.

4. Two identical water bottles one empty and the other filled with water are allowed to roll down an inclined plane. Which one of them reaches the bottom first? Explain your answer.

Answer

Water filled bottle. Because the moment of inertia of the empty bottle is higher than the moment of inertia of the water filled bottle.

 

5. Write the relation between angular momentum and rotational kinetic energy. Draw a graph for the same. For two objects of same angular momentum, compare the moment of inertia using the graph.

Answer:

Angular momentum, L = Iω.

Rotational K.E., EK = 1/2 Iω2

Multiply by 21 both sides (21) EK = —— 2IEk = (Ico)2

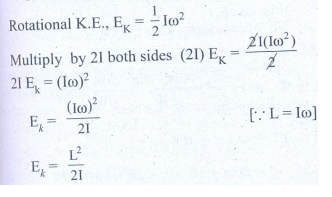
Where, L is the angular momentum and I is the moment of inertia.

Graph between KE and L :

The graph shows that of the two bodies of same angular momentum, those one have less rotational kinetic energy has higher moment of inertia.

6. A rectangle block rests on a horizontal table. A horizontal force is applied on the block at a height h above the table to move the block. Does the line of action of the normal force N exerted by the table on the block depend on h?

Answer

(i) Yes. The line of action of the normal force N exerted by the table on the block depend on h.

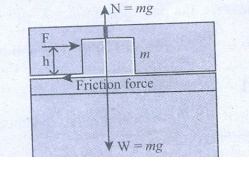
(ii) When height of the applied force ‘h’ increases, a torque is produced by the applied force and frictional force such that block start to tilt.

(iii) To balance this effect, line of action of normal force shift away’from applied force and make a opposite torque, joining with gravitational force ‘W’.

7. Three identical solid spheres move down through three inclined planes A, B and C all same dimensions. A is without friction, B is undergoing pure rolling and C is rolling with slipping. Compare the kinetic energies EA, EB and EC at the bottom.

Answer

(i) In this case, when three identical solid spheres starts to move on the inclined planes, they all have same potential energy.

(ii) During the motion, the potential energy is converted into kinetic energy.

(iii) According to law of conservation of energy, at the bottom all the potential energy is converted into kinetic energy.

(iv) Such that all three spheres have same kinetic energy at the bottom whatever be the type of motion, i.e. EA = EB = Ec.

8. Give an example to show that the following statement is false. ‘any two forces acting on a body can be combined into single force that would have same effect’.

Answer

(i) Consider two equal and opposite forces acting on a wheel.

(ii) If two forces combined and acting on single point on the wheel, there will be no effect. However, if they act separately on the edges of the wheel, there will be a rotating effect.

(iii) This example falsifies the given statement.