Electrostatics - Electric potential due to a point charge | 12th Physics : Electrostatics
Chapter: 12th Physics : Electrostatics
Electric potential due to a point charge
Electric potential due to a point charge
Consider a positive
charge q kept fixed at the origin. Let P be a point at distance r from the
charge q. This is shown in Figure 1.23.

The electric potential
at the point P is
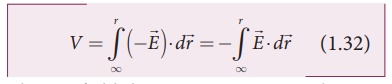
Electric field due to
positive point charge q is
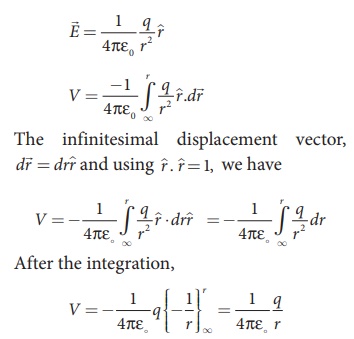
Hence the electric potential due to
a point charge q at a distance r is
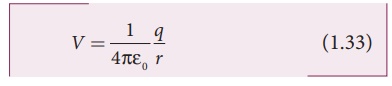
Important points
(i) If the source charge
q is positive, V > 0. If q is negative, then V is negative and equal to

(ii) The description of motion of objects using the concept of potential or potential energy is simpler than that using the concept of field.
(iii) From expression
(1.33), it is clear that the potential due to positive charge decreases as the
distance increases, but for a negative charge the potential increases as the
distance is increased. At infinity (r = ∞) electrostatic potential is zero (V = 0).
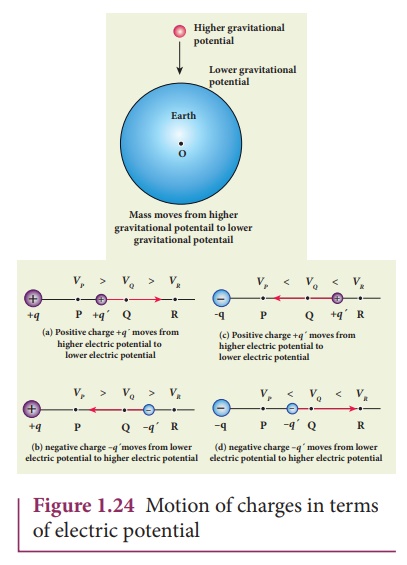
In the case of
gravitational force, mass moves from a point of higher gravitational potential
to a point of lower gravitational potential. Similarly a positive charge moves
from a point of higher electrostatic potential to lower electrostatic
potential. However a negative charge moves from lower electrostatic potential
to higher electrostatic potential. This comparison is shown in Figure 1.24.
(iv) The electric
potential at a point P due to a collection of charges q1,q2,q3…..qn
is equal to sum of the electric potentials due to individual charges.
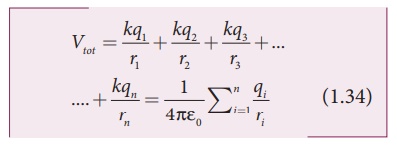
where r1,r2,r3…….rn
are the distances of q1, q2, q3 ….. qn
respectively from P (Figure 1.25).
EXAMPLE 1.12
(a) Calculate the
electric potential at points P and Q as shown in the figure below.
(b) Suppose the charge
+9µC is replaced by -9µC find the electrostatic potentials at points P and Q
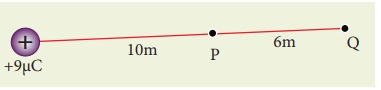
( c ) Calculate the work
done to bring a test charge +2µC from infinity to the point P. Assume the
charge +9µC is held fixed at origin and +2µC is brought from infinity to P.
Solution
(a) Electric potential
at point P is given by
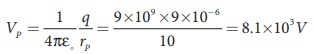
Electric potential at
point Q is given by
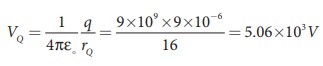
Note that the electric
potential at point Q is less than the electric potential at point P. If we put
a positive charge at P, it moves from P to Q. However if we place a negative
charge at P it will move towards the charge +9µC.
The potential difference
between the points P and Q is given by
∆V = VP −VQ
= +3 .04 ×103V
(b) Suppose we replace
the charge +9 µC by -9 µC, then the corresponding potentials at the points P
and Q are,
VP = −8 .
1×103 V , VQ =− 5.06 ×103V
Note that in this case
electric potential at the point Q is higher than at point P.
The potential difference
or voltage between the points P and Q is given by
∆V = VP −VQ
= −3 .04 ×103V
(c) The electric
potential V at a point P due to some charge is defined as the work done by an
external force to bring a unit positive charge from infinity to P. So to bring
the q amount of charge from infinity to the point P, work done is given as
follows.
W = qV
WQ = 2 ×10−
6 × 5. 06 ×103 J = 10 .12 ×10−3 J.
EXAMPLE 1.13
Consider a point charge
+q placed at the origin and another point charge -2q placed at a distance of 9
m from the charge +q. Determine the point between the two charges at which
electric potential is zero.
Solution
According to the
superposition principle, the total electric potential at a point is equal to
the sum of the potentials due to each charge at that point.
Consider the point at
which the total potential zero is located at a distance x from the charge +q as
shown in the figure.
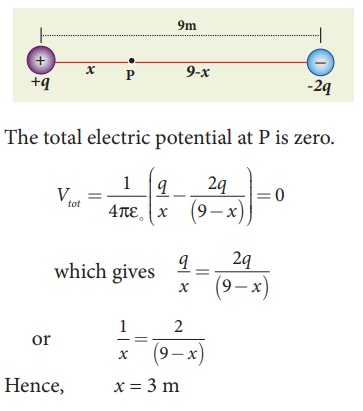
Related Topics