Chapter: 12th Physics : Electrostatics
Distribution of charges in a conductor
Distribution of charges in a conductor
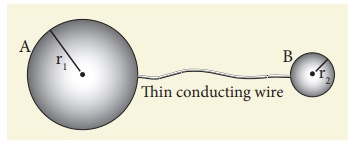
Consider two conducting
spheres A and B of radii r1 and r2 respectively connected
to each other by a thin conducting wire as shown in the Figure 1.62. The
distance between the spheres is much greater than the radii of either spheres.
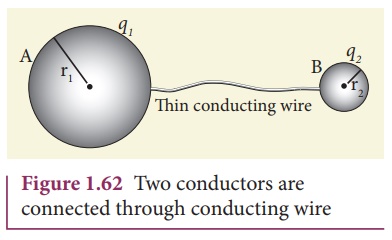
If a charge Q is
introduced into any one of the spheres, this charge Q is redistributed into
both the spheres such that the electrostatic potential is same in both the
spheres. They are now uniformly charged and attain electrostatic equilibrium.
Let q1 be the charge residing on the surface of sphere A and q2
is the charge residing on the surface of sphere B such that Q = q1 +
q2. The charges are distributed only on the surface and there is no
net charge inside the conductor.
The electrostatic
potential at the surface of the sphere A is given by
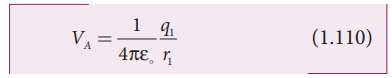
The electrostatic
potential at the surface of the sphere B is given by
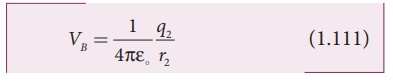
The surface of the
conductor is an equipotential. Since the spheres are connected by the
conducting wire, the surfaces of both the spheres together form an
equipotential surface. This implies that
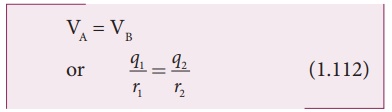
Let us take the charge
density on the surface of sphere A is σ1 and charge density on the
surface of sphere B is σ2. This implies that q1 = 4πr12σ1
and q2 = 4πr22σ2. Substituting
these values into equation (1.112), we get

Thus the surface charge
density σ is inversely proportional to the radius of the sphere. For a smaller
radius, the charge density will be larger and vice versa.
EXAMPLE 1.23
Two conducting spheres
of radius r1 = 8 cm and r2 = 2 cm are separated by a
distance much larger than 8 cm and are connected by a thin conducting wire as
shown in the figure. A total charge of Q = +100 nC is placed on one of the
spheres. After a fraction of a second, the charge Q is redistributed and both
the spheres attain electrostatic equilibrium.
(a) Calculate the charge
and surface charge density on each sphere.
(b) Calculate the potential
at the surface of each sphere.
Solution
(a) The electrostatic
potential on the surface of the sphere A is 
The electrostatic potential on the surface of the sphere A is 
Since VA = VB. We have
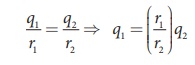
But from the
conservation of total charge, Q = q1
+ q2, we get q1 = Q – q2. By substituting this
in the above equation,

Note that the surface
charge density is greater on the smaller sphere compared to the larger sphere
(σ2 ≈ 4σ1) which confirms the result σ1 / σ1=
r2 / r2.
The potential on both
spheres is the same. So we can calculate the potential on any one of the
spheres.
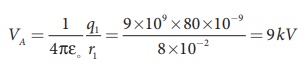
Related Topics