Solved Example Problems on Alternating Current (AC) and Circuit

EXAMPLE 4.18

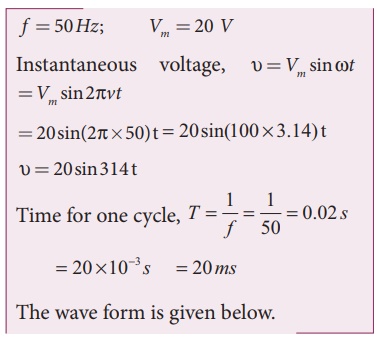
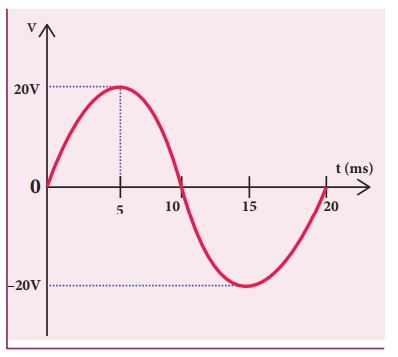
Write down the equation for a sinusoidal voltage of 50 Hz and its peak value is 20 V. Draw the corresponding voltage versus time graph.

Solution

EXAMPLE 4.19

The equation for an alternating current is given by i = 77 sin 314t. Find the peak value, frequency, time period and instantaneous value at t = 2 ms.

Solution

i = 77 sin 314t ; t = 2 ms = 2×10-3 s

The general equation of an alternating current is i = Im sin ωt . On comparsion,

(i) Peak value, Im = 77 A

(ii) Frequency, f = ω/2Ï€ = 314 / 2 ×3.14 = 50 Hz

Time period, T = 1/f = 150 = 0 .02 s

(iv) At t = 2 m s,

Instantaneous value,

i = 77sin(314×2×10−3 )

 i = 45.24 A

AC circuit containing only an inductor: Solved Example Problems

EXAMPLE 4.20

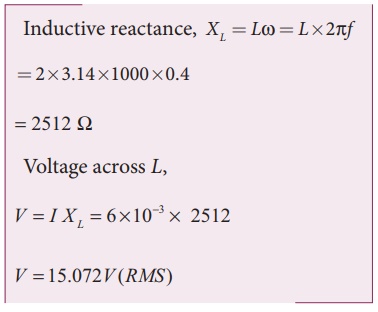
A 400 mH coil of negligible resistance is connected to an AC circuit in which an effective current of 6 mA is flowing. Find out the voltage across the coil if the frequency is 1000 Hz.

Solution

L = 400 x 10-3 H; Ieff = 6 x 10-3A

f = 1000 Hz

AC circuit containing only a capacitor: Solved Example Problems

EXAMPLE 4.21

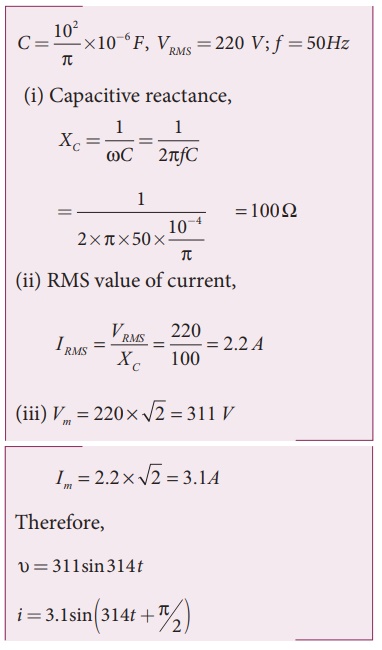
A capacitor of capacitance 102/π µF is connected across a 220 V, 50 Hz A.C. mains. Calculate the capacitive reactance, RMS value of current and write down the equations of voltage and current.

Solution

Quality factor or Q–factor: Solved Example Problems

EXAMPLE 4.22

Find the impedance of a series RLC circuit if the inductive reactance, capacitive reactance and resistance are 184 Ω, 144 Ω and 30 Ω respectively. Also calculate the phase angle between voltage and current.

Solution

XL = 184 Ω; XC = 144 Ω

R = 30 Ω

(i ) The impedance is

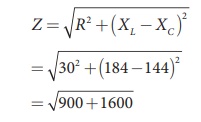
Impedance, Z = 50 Ω

(ii) Phase angle is

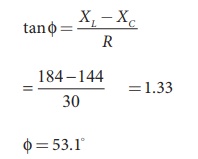
φ = 53.1

EXAMPLE 4.23

A 500 μH inductor, 80/Ï€2 pF capacitor and a 628 Ω resistor are connected to form a series RLC circuit. Calculate the resonant frequency and Q-factor of this circuit at resonance.

Solution

L=500×10-6H; C = 80/Ï€2 Ã—10−12 F; R = 628Ω

(i) Resonant frequency is

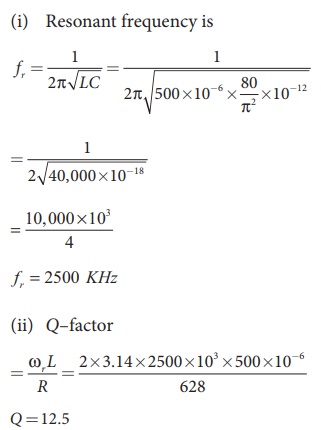
 Q =12.5

EXAMPLE 4.24

Find the instantaneous value of alternating voltage Ï… = 10 sin(3 π×104 t) volt at i) 0 s ii) 50 μs iii) 75 μs.

Solution

The given equation is Ï… = 10sin (3 π×104 t)

EXAMPLE 4.25

The current in an inductive circuit is given by 0.3 sin (200t – 40°) A. Write the equation for the voltage across it if the inductance is 40 mH.

Solution

L = 40 × 10-3 H; i = 0.1 sin (200t – 40º)

XL = ωL = 200 × 40 × 10-3 = 8 Ω

Vm = Im XL = 0.3 × 8 = 2.4 V

In an inductive circuit, the voltage leads the current by 90o Therefore,

v = Vm sin ( ωt +90º)

 v = 2 . 4 sin(200t −40 + 90 º)

v = 2 . 4 sin(200t +50 º)volt