Definition, Explanation, Formulas, Solved Example Problems | Electromagnetic Induction - Mutual inductance between two long co-axial solenoids | 12th Physics : Electromagnetic Induction and Alternating Current
Chapter: 12th Physics : Electromagnetic Induction and Alternating Current
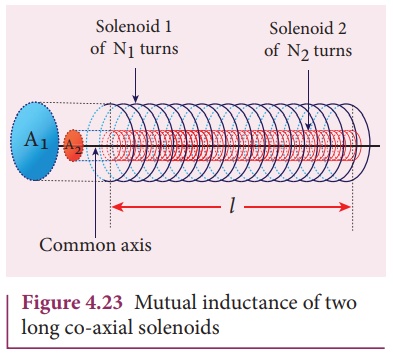
Mutual inductance between two long co-axial solenoids
Mutual inductance between two long
co-axial solenoids
Consider two long
co-axial solenoids of same length l. The length of these solenoids is
large when compared to their radii so that the magnetic field produced inside
the solenoids is uniform and the fringing effect at the ends may be ignored.
Let A1 and A2 be the area of cross section
of the solenoids with A1 being greater than A2
as shown in Figure 4.23. The turn density of these solenoids are n1
and n2 respectively.
Let i1
be the current flowing through solenoid 1, then the magnetic field produced
inside it is

As the field lines of ![]() 1
are passing through the area bounded by solenoid 2, the magnetic flux is linked
with each turn of solenoid 2 due to solenoid 1 and is given by
1
are passing through the area bounded by solenoid 2, the magnetic flux is linked
with each turn of solenoid 2 due to solenoid 1 and is given by

The flux linkage of
solenoid 2 with total turns N2 is

Comparing the equations
(4.20) and (4.21),

This gives the
expression for mutual inductance M21 of the solenoid 2 with
respect to solenoid 1. Similarly, we can find mutual inductance M12
of solenoid 1 with respect to solenoid 2 as given below.
The magnetic field
produced by the solenoid 2 when carrying a current i2 is
B2 = ┬Ą n2i2
This magnetic field B2
is uniform inside the solenoid 2 but outside the solenoid 2, it is almost zero.
Therefore for solenoid 1, the area A2 is the effective area
over which the magnetic field B2 is present; not area A1.
Then the magnetic flux ╬”12 linked with each
turn of solenoid 1 due to solenoid 2 is
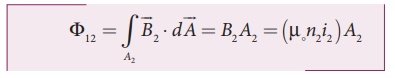
The flux linkage of
solenoid 1 with total turns N1 is

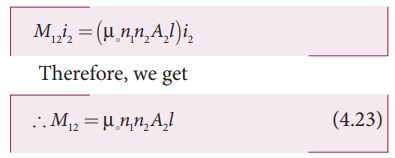
From equation (4.22) and
(4.23), we can write

In general, the mutual
inductance between two long co-axial solenoids is given by

If a dielectric medium
of relative permeability ┬Ąr is present inside the solenoids, then
M = ┬Ą n1 n2 A2
l
(or) M = ┬Ą ┬Ąr n1 n2 A2 l
EXAMPLE 4.12
The current flowing in
the first coil changes from 2 A to 10 A in 0.4 sec. Find the mutual inductance
between two coils if an emf of 60 mV is induced in the second coil. Also
determine the induced emf in the second coil if the current in the first coil
is changed from 4 A to 16 A in 0.03 sec. Consider only the magnitude of induced
emf.
Solution
Case (i):
di1 = 10 ŌĆō 2 = 8 A; dt =
0.4 s;
╬Ą2 = 60 ├Ś10ŌłÆ3V
Case (ii):
di1 = 16 ŌĆō 4
= 12 A;
dt = 0.03 s
(i) Mutual inductance of
the second coil with respect to the first coil
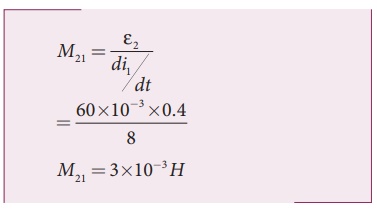
(ii) Induced emf in the
second coil due to the rate of change of current in the first coil is
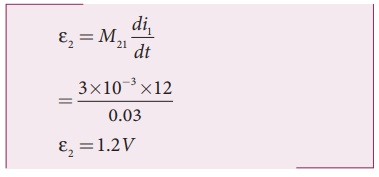
EXAMPLE 4.13
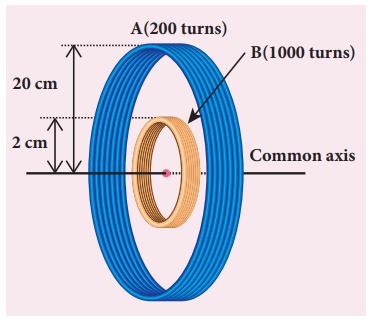
Consider two coplanar,
co-axial circular coils A and B as shown in figure. The radius of coil A is 20
cm while that of coil B is 2 cm. The number of turns is 200 and 1000 for coils
A and B respectively. Calculate the mutual inductance of coil B with respect to
coil A. If the current in coil A changes from 2 A to 6 A in 0.04 sec, determine
the induced emf in coil B and the rate of change of flux through the coil B at
that instant.
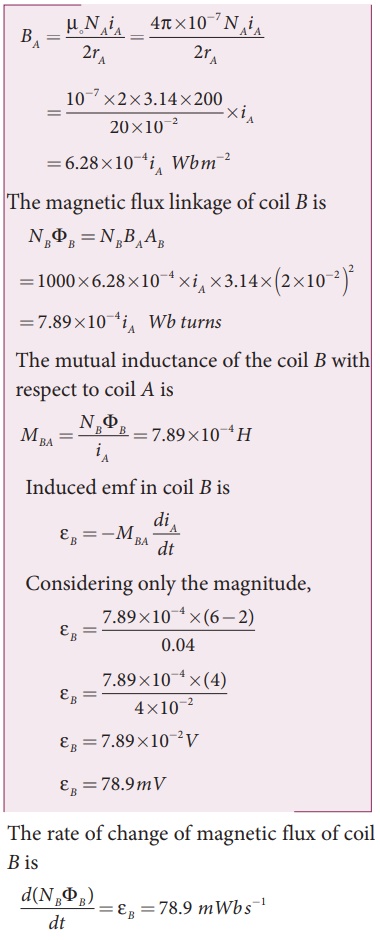
Solution
NA = 200
turns; NB = 1000 turns;
rA = 20 ├Ś 10-2
m; rB = 2 ├Ś 10-2 m;
dt = 0.04 s; diA
= 6ŌłÆ2 = 4A
Let iA be the
current flowing in coil A, then the magnetic field BA at the centre
of the circular coil A is
The rate of change of magnetic flux of coil is
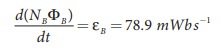
Related Topics