Chapter: 11th Physics : UNIT 11 : Waves
Standing Waves or stationary waves
STANDING
WAVES
Explanation of stationary waves
When
the wave hits the rigid boundary it bounces back to the original medium and can
interfere with the original waves. A pattern is formed, which are known as
standing waves or stationary waves. Consider two harmonic progressive waves
(formed by strings) that have the same amplitude and same velocity but move in
opposite directions. Then the displacement of the first wave (incident wave) is
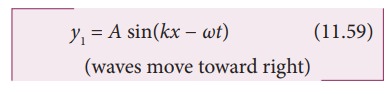
and
the displacement of the second wave (reflected wave) is

both
will interfere with each other by the principle of superposition, the net
displacement is

Substituting
equation (11.59) and equation (11.60) in equation (11.61), we get
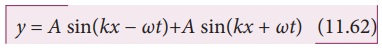
Using
trigonometric identity, we rewrite equation (11.62) as
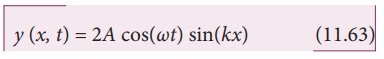
This represents a stationary wave or standing wave, which
means that this wave does not move either forward or backward, whereas
progressive or travelling waves will move forward or backward. Further, the
displacement of the particle in equation (11.63) can be written in more compact
form,
y(x,t) =
Aʹ cos(ωt)
where,
Aʹ = 2Asin(kx), implying that the particular element of the string executes
simple harmonic motion with amplitude equals to Aʹ. The maximum of this amplitude occurs at positions for which
sin(kx) =1
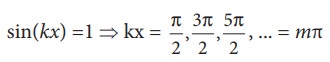
where
m takes half integer or half integral
values. The position of maximum amplitude is known as antinode. Expressing wave number in terms of wavelength, we can
represent the anti-nodal positions as
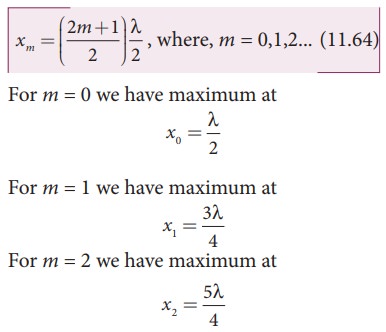
and
so on.
The
distance between two successive anti-nodes can be computed by
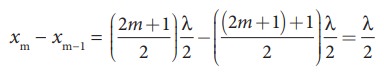
Similarly,
the minimum of the amplitude A' also occurs at some points in the space, and
these points can be determined by setting
sin(kx)= 0 ⇒
k x = 0,π,2π,3π,… = n π
where
n takes integer or integral values.
Note that the elements at these points do not vibrate (not move), and the
points are called nodes. The nthnodal positions is given by,

For
n = 0 we have minimum at
x0 = 0
For
n = 1 we have minimum at
x1 = λ /2
For
n = 2 we have maximum at
x2 = λ
and
so on.
The
distance between any two successive nodes can be calculated as
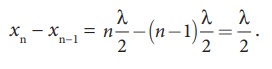
EXAMPLE 11.20
Compute
the distance between anti-node and neighbouring node.
Solution
For
nth mode, the distance between
anti-node and neighbouring node is
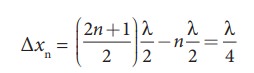
Characteristics of stationary waves
(1) Stationary waves are characterised by the confinement of a wave disturbance between two rigid boundaries. This means, the wave does not move forward or backward in a medium (does not advance), it remains steady at its place. Therefore, they are called “stationary waves or standing waves”.
(2)
Certain points in the region in which the wave exists have maximum amplitude, called as
anti-nodes and at certain points the
amplitude is minimum or zero, called as nodes.
(3)
The distance between two consecutive nodes (or) anti-nodes is λ/2 .
(4)
The distance between a node and its neighbouring anti-node is λ/4.
(5)
The transfer of energy along the standing wave is zero.
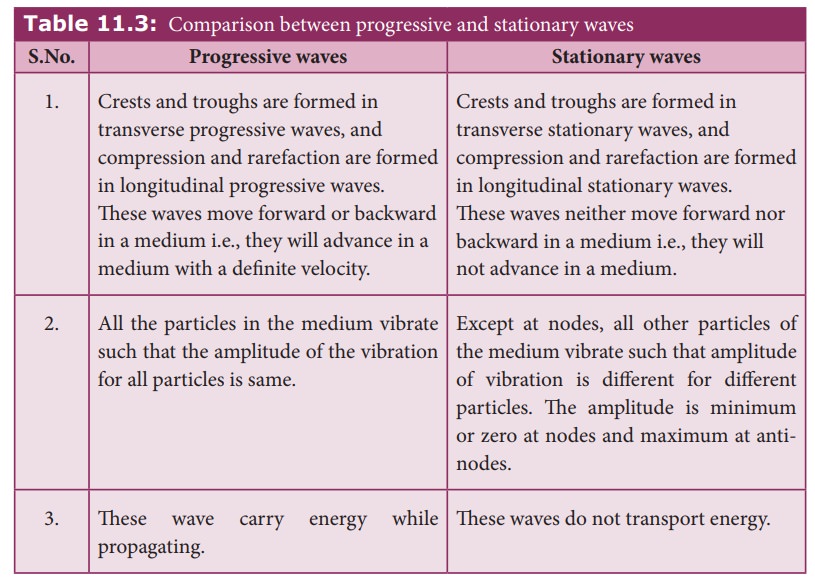
Stationary waves in sonometer
Sono means sound related, and sonometer implies sound-related measurements. It is a device for demonstrating the relationship between the frequency of the sound produced in the transverse standing wave in a string, and the tension, length and mass per unit length of the string. Therefore, using this device, we can determine the following quantities:
(a) the frequency
of the tuning fork or frequency of alternating current
(b)
the tension in the string
(c)
the unknown hanging mass
Construction:
The
sonometer is made up of a hollow box which is one meter long with a uniform
metallic thin string attached to it. One end of the string is connected to a
hook and the other end is connected to a weight hanger through a pulley as
shown in Figure 11.34. Since only one string is used, it is also known as monochord.
The weights are added to the free end of the wire to increase the tension of
the wire. Two adjustable wooden knives are put over the board, and their
positions are adjusted to change the vibrating length of the stretched wire.
Working :
A
transverse stationary or standing wave is produced and hence, at the knife
edges P and Q, nodes are formed. In between the knife edges, anti-nodes are
formed.
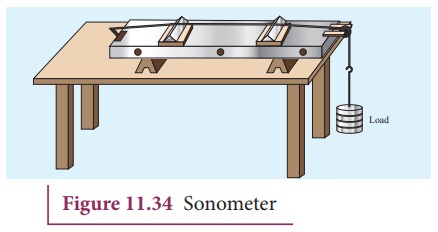
If the length of the vibrating element is l then
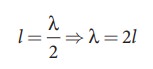
Let f be the frequency of the vibrating element, T the tension of in the string and μ the mass per unit length of the string. Then using equation (11.13), we get
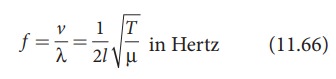
Let
ρ be the density of the material of
the string and d be the diameter of
the string. Then the mass per unit length μ,
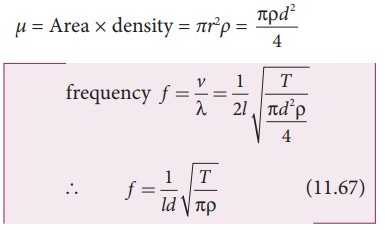
EXAMPLE 11.21
Let
f be the fundamental frequency of the
string. If the string is divided into three segments l1, l2
and l3 such that the
fundamental frequencies of each segments be f1,
f2 and f3, respectively. Show that

Solution
For
a fixed tension T and mass density µ,
frequency is inversely proportional to the string length i.e.

Fundamental frequency and overtones
Let
us now keep the rigid boundaries at x =
0 and x = L and produce a standing waves by wiggling the string (as in
plucking strings in a guitar). Standing waves with a specific wavelength are
produced. Since, the amplitude must vanish at the boundaries, therefore, the
displacement at the boundary must satisfy the following conditions
y(x = 0, t) = 0 and y(x = L, t) = 0 (11.68)
Since
the nodes formed are at a distance λn/2 apart, we have n(λn/2)
= L, where n is an integer, L is the length between the two boundaries and λn
is the specific wavelength that satisfy the specified boundary conditions.
Hence,

Therefore,
not all wavelengths are allowed. The (allowed) wavelengths should fit with the
specified boundary conditions, i.e., for n = 1, the first mode of vibration has
specific wavelength λl =
2L. Similarly for n = 2, the second mode of vibration has specific wavelength

For
n = 3, the third mode of vibration has specific wavelength
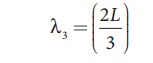
and
so on.
The
frequency of each mode of vibration (called natural frequency) can be
calculated.
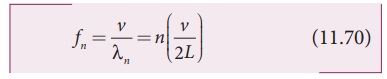
The
lowest natural frequency is called the fundamental
frequency.
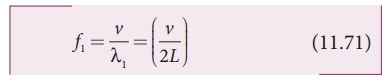
The
second natural frequency is called the first
over tone.
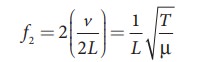
The
third natural frequency is called the second
over tone.
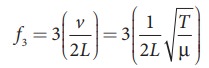
Therefore,
the nth natural frequency can be computed as integral (or integer )
multiple of fundamental frequency, i.e.,
fn = nf1, where n
is an integer (11.72)
If natural frequencies are written
as integral multiple of fundamental frequencies, then the frequencies are
called harmonics.
Thus, the first harmonic is f1 = f1 (the fundamental frequency is called first harmonic),
the second harmonic is f2
= 2f1, the third harmonic
is f3 = 3f1 etc.
EXAMPLE 11.22
Consider
a string in a guitar whose length is 80 cm and a mass of 0.32 g with tension 80
N is plucked. Compute the first four lowest frequencies produced when it is
plucked.
Solution
The
velocity of the wave
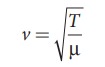
The length of the string, L = 80 cm=0.8 m The mass of the string, m = 0.32 g = 0.32 × 10-3kg
Therefore,
the linear mass density,
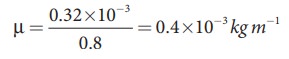
The
tension in the string, T = 80 N

The
wavelength corresponding to the fundamental frequency f1 is λ1
= 2L = 2 × 0.8 = 1.6 m
The
fundamental frequency f1
corresponding to the wavelength λ1
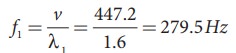
Similarly,
the frequency corresponding to the second harmonics, third harmonics and fourth
harmonics are
f2 = 2f1 = 559 Hz
f3 = 3f1 = 838.5 Hz
f4 = 4f1 = 1118 Hz
Laws of transverse vibrations in stretched strings
There
are three laws of transverse vibrations of stretched strings which are given as
follows:
(i) The law of length :
For
a given wire with tension T (which is
fixed) and mass per unit length μ
(fixed) the frequency varies inversely with the vibrating length. Therefore,

⇒l×f = C, where C is a constant
(ii) The law of tension:
![]()
![]() For a given vibrating length l (fixed) and mass per unit length μ (fixed) the frequency varies directly with the square root of the
tension T,
For a given vibrating length l (fixed) and mass per unit length μ (fixed) the frequency varies directly with the square root of the
tension T,

(iii) The law of mass:
For
a given vibrating length l (fixed)
and tension T (fixed) the frequency
varies inversely with the square root of the mass per unit length μ,
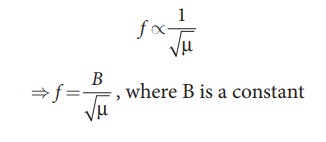
Related Topics