Chapter: 11th Physics : UNIT 7 : Properties of Matter
Solved Example Problems for Buoyancy
Physics : Properties of Matter - Solved Example Problems for Buoyancy
EXAMPLE 7.8
A cube of wood floating in water supports a 300 g mass at the centre of its top face. When the mass is removed, the cube rises by 3 cm. Determine the volume of the cube.
Solution
Let each side of the cube be l. The volume occupied by 3 cm depth of cube,
V=(3cm) ├Ś l2 = 3l2cm
According to the principle of floatation, we have
VŽüg = mg ŌćÆ VŽü = m
Žü is density of water = 1000 kg m-3
(3l2 ├Ś 10-2m) ├Ś (1000 kgm-3)=300 ├Ś 10-3ŌĆåkg
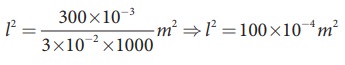
l = 10 ├Ś 10-2m = 10 cm
Therefore, volume of cube V = l3 = 1000 cm3
Study Material, Lecturing Notes, Assignment, Reference, Wiki description explanation, brief detail
11th Physics : UNIT 7 : Properties of Matter : Solved Example Problems for Buoyancy |
Related Topics
11th Physics : UNIT 7 : Properties of Matter