Bernoulli’s Theorem

BERNOULLI’S THEOREM

Equation of continuity

In order to discuss the mass flow rate through a pipe, it is necessary to assume that the flow of fluid is steady, the flow of the fluid is said to be steady if at any given point, the velocity of each passing fluid particle remains constant with respect to time. Under this condition, the path taken by the fluid particle is a streamline.

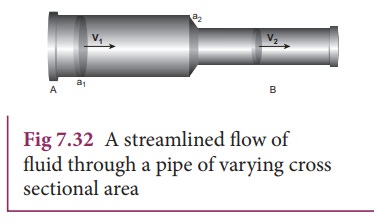
Consider a pipe AB of varying cross sectional area a₁ and a₂ such that a₁ > a₂. A non-viscous and incompressible liquid flows steadily through the pipe, with velocities v₁ and v₂ in area a₁ and a₂, respectively as shown in Figure 7.32.

Let m₁ be the mass of fluid flowing through section A in time Δt, m₁ = (a₁v₁ t) ρ

Let m₂ be the mass of fluid flowing through section B in time t, m₂ = (a₂v₂ t) ρ

For an incompressible liquid, mass is conserved m₁ = m₂

a₁ v₁ Δt ρ = a₂ v₂ Δt ρ

which is called the equation of continuity and it is a statement of conservation of mass in the flow of fluids.

In general, a v = constant, which means that the volume flux or flow rate remains constant throughout the pipe. In other words, the smaller the cross section, greater will be the velocity of the fluid.

EXAMPLE 7.14

In a normal adult, the average speed of the blood through the aorta (radius r = 0.8 cm) is 0.33 ms^-1. From the aorta, the blood goes into major arteries, which are 30 in number, each of radius 0.4 cm. Calculate the speed of the blood through the arteries.

Solution:

a₁v₁ = 30 a₂ v₂ ⇒ π r₁²v₁  = 30 π r₂²v₂

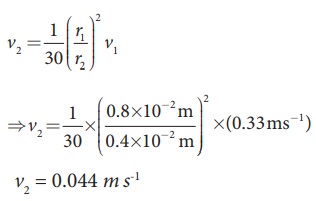
Pressure, kinetic and potential energy of liquids

A liquid in a steady flow can possess three kinds of energy. They are (1) Kinetic energy, (2) Potential energy, and (3) Pressure energy, respectively.

i) Kinetic energy:  The kinetic energy of a liquid of mass m moving with a velocity v is given by

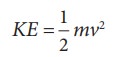
The kinetic energy per unit mass =

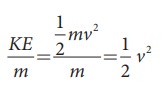
Similarly, the kinetic energy per unit volume

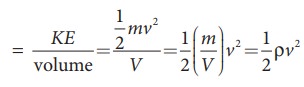
ii) Potential energy: The potential energy of a liquid of mass m at a height h above the ground level is given by

PE = mgh

The potential energy per unit mass

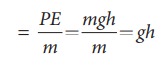
Similarly, the potential energy per unit volume =

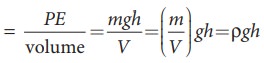
ii) Pressure energy: The energy acquired by a fluid by applying pressure on the fluid. We know that

Pressure = Force/Area ⇒ Force = Pressure × Area

F × d = (P A) × d = P (A × d)

F × d = W = P V = pressure energy

Therefore, pressure energy, E_P = PV The pressure energy per unit mass =

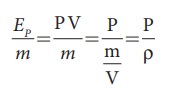
Similarly,  the  potential  energy  per  unit volume =

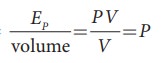
Bernoulli’s theorem and its applications

In 1738, the Swiss scientist Daniel Bernoulli developed a relationship for the flow of fluid through a pipe of varying cross section. He proposed a theorem for the streamline flow of a liquid based on the law of conservation of energy.

Bernoulli’s theorem

According to Bernoulli’s theorem, the sum of pressure energy, kinetic energy, and potential energy per unit mass of an incompressible, non-viscous fluid in a streamlined flow remains a constant. Mathematically,

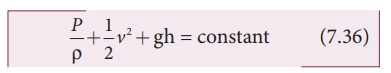
This is known as Bernoulli’s equation.

Proof:

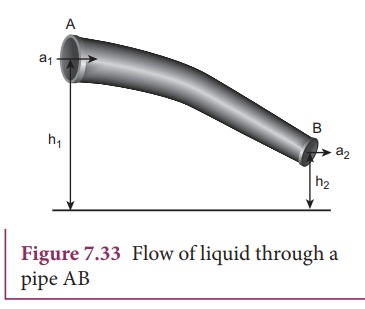
Let us consider a flow of liquid through a pipe AB as shown in Figure 7.33. Let V be the volume of the liquid when it enters A in a time t which is equal to the volume of the liquid leaving B in the same time. Let a_A, v_A and P_A be the area of cross section of the tube, velocity of the liquid and pressure exerted by the liquid at A respectively.

Let the force exerted by the liquid at A is

F_A = P_Aa_A

Distance travelled by the liquid in time t is

d = v_A t

Therefore, the work done is

W = F_Ad = P_Aa_Av_A t

But a_Av_At = a_Ad =V, volume of the liquid entering at A.

Thus, the work done is the pressure energy (at A), 

W = F_Ad = P_AV

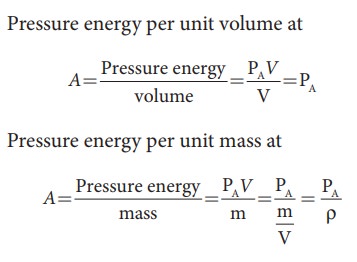
Since m is the mass of the liquid entering at A in a given time, therefore, pressure energy of the liquid at A is

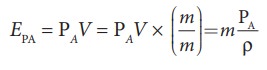
Potential energy of the liquid at A,

PE_A = mg h_A,

Due to the flow of liquid, the kinetic energy of the liquid at A,

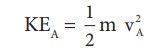
Therefore, the total energy due to the flow of liquid at A, E_A = EP_A + KE_A + PE_A

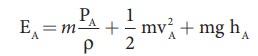
Similarly, let a_B, v_B, and P_B be the area of cross section of the tube, velocity of the liquid, and pressure exerted by the liquid at B. Calculating the total energy at EB, we get

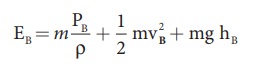
From the law of conservation of energy,

EA = EB

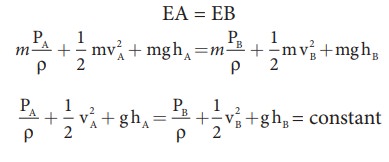
Thus, the above equation can be written as

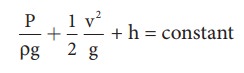
The above equation is the consequence of the conservation of energy which is true until there is no loss of energy due to friction. But in practice, some energy is lost due to friction. This arises due to the fact that in a fluid flow, the layers flowing with different velocities exert frictional forces on each other. This loss of energy is generally converted into heat energy. Therefore, Bernoulli’s relation is strictly valid for fluids with zero viscosity or non-viscous liquids. Notice that when the liquid flows through a horizontal pipe, then h = 0

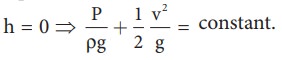
Applications of Bernoulli’s Theorem

(a) Blowing off roofs during wind storm In olden days, the roofs of the huts or houses were designed with a slope as shown in Figure.7.34. One important scientific reason is that as per the Bernoulli’s principle, it will be safeguarded except roof during storm or cyclone.

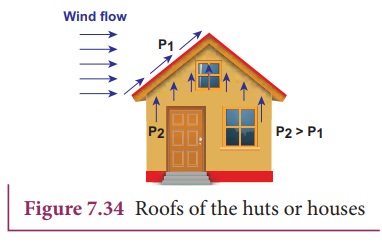
During cyclonic condition, the roof is blown off without damaging the other parts of the house. In accordance with the Bernoulli’s principle, the high wind blowing over the roof creates a low-pressure P₁. The pressure under the roof P₂ is greater. Therefore, this pressure difference (P₂–P₁) creates an up thrust and the roof is blown off.

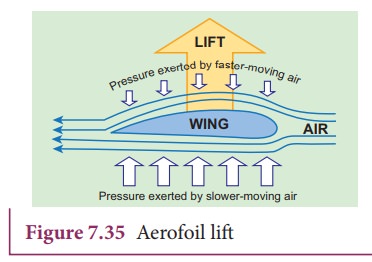
(b) Aerofoil lift

The wings of an airplane (aerofoil) are so designed that its upper surface is more curved than the lower surface and the front edge is broader than the real edge. As the aircraft moves, the air moves faster above the aerofoil than at the bottom as shown in Figure 7.35.

According to Bernoulli’s Principle, the pressure of air below is greater than above, which creates an upthrust called the dynamic lift to the aircraft.

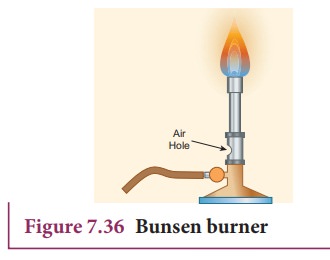
(c) Bunsen burner

In this, the gas comes out of the nozzle with high velocity, hence the pressure in the stem decreases. So outside air reaches into the burner through an air vent and the mixture of air and gas gives a blue flame as shown in Figure 7.36.

(d) Venturimeter

This device is used to measure the rate of flow (or say flow speed) of the incompressible fluid flowing through a pipe. It works on the principle of Bernoulli’s theorem. It consists of two wider tubes A and A' (with cross sectional area A) connected by a narrow tube B (with cross sectional area a). A manometer in the form of U-tube is also attached between the wide and narrow tubes as shown in Figure7.37. The manometer contains a liquid of density ‘ρ_m’.

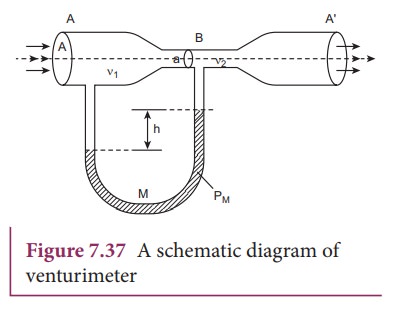
Let P₁ be the pressure of the fluid at the wider region of the tube A. Let us assume that the fluid of density ‘ρ’ flows from the pipe with speed ‘v₁’ and into the narrow region, its speed increases to ‘v₂’. According to the Bernoulli’s equation, this increase in speed is accompanied by a decrease in the fluid pressure P₂ at the narrow region of the tube B. Therefore, the pressure difference between the tubes A and B is noted by measuring the height difference (ΔP = P₁−P₂) between the surfaces of the manometer liquid.

From the equation of continuity, we can say that Av₁ = a v₂ which means that

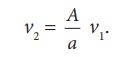
Using Bernoulli’s equation,

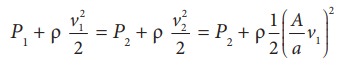
From the above equation, the pressure difference

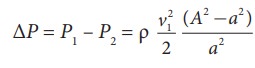
Thus, the speed of flow of fluid at the wide end of the tube A

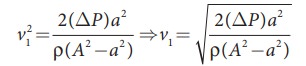
The volume of the liquid flowing out per second is

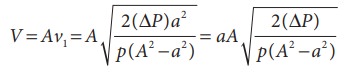
(e) Other applications

This Bernoulli’s concept is mainly used in the design of carburetor of automobiles, filter pumps, atomizers, and sprayers. 

For example, the carburetor has a very fine channel called nozzle through which the air is allowed to flow in larger speed. In this case, the pressure is lowered at the narrow neck and in turn, the required fuel or petrol is sucked into the chamber so as to provide the correct mixture of air and fuel necessary for ignition process.