Equation of a plane perpendicular to a vector and passing through a given point

Equation of a plane perpendicular to a vector and passing through a given point

(a) Vector form of equation

Consider a plane passing through a point A with position vector  and  is a normal vector to the given plane.

Let  be the position vector of an arbitrary point P on the plane.

Then  is perpendicular to .

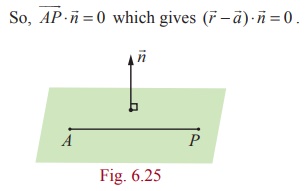
which is the vector form of the equation of a plane passing through a point with position vector  and perpendicular to .

Note

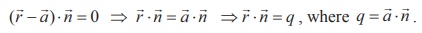
(b) Cartesian form of equation

If a, b, c are the direction ratios of , then we have  = aiˆ + bˆj + ckˆ.

Suppose, A is (x₁ , y₁ , z₁) then equation (1) becomes ((x − x₁ )iˆ + ( y − y₁ ) ˆj + (z − z₁ )kˆ) â‹… (aiˆ + bˆj + ckˆ) = 0 . That is,

a(x − x₁) + b( y − y₁) + c(z − z₁) = 0

which is the Cartesian equation of a plane, normal to a vector with direction ratios a, b, c and passing through a given point (x₁ , y₁ , z₁) .