A point on the straight line and the direction of the straight line

A point on the straight line and the direction of the straight line  are given

(A) Parametric form of vector equation

Theorem 6.11

The vector equation of a straight line passing through a fixed point with position vector  and parallel to a given vector  is  =  + t, where t∈ R.

Proof

If  is the position vector of a given point A and  is the position vector of an arbitrary point  P on the straight line, then

=  - .

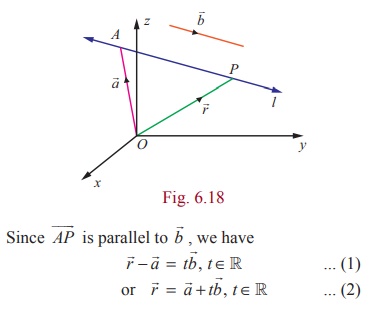
This is the vector equation of the straight line in parametric form.

Remark

The position vector of any point on the line is taken as  + t.

(b) Non-parametric form of vector equation

Since  is parallel to  , we have   Ã—  = 

That is, ( âˆ’ ) ×  = 0 .

This is known as the vector equation of the straight line in non-parametric form.

(c) Cartesian equation

Suppose P is (x, y, z) , A is (x1 , y1 , z1 ) and  = b1 Ë†i + b2 Ë†j + b3 Ë†k . Then, substituting  = x Ë†i + y Ë†j + z Ë†k ,  = x1ˆi + y1ˆ j + z1 Ë†k  in (1) and comparing the coefficients of ˆi , ˆj, ˆk , we get

x âˆ’ x1 = tb1 , y âˆ’ y1 = tb2 , z âˆ’ z1 = tb3                     â€¦â€¦â€¦â€¦.(4)

Conventionally (4) can be written as

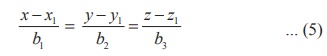
which are called the Cartesian equations or symmetric equations of a straight line passing through the point (x1, y1 , z1) and parallel to a vector with direction ratios b1, b2, b3.

Remark

(i) Every point on the line (5) is of the form (x1 + tb1 , y1 + tb2 , z1 + tb3) , where t âˆˆ R.

(ii) Since the direction cosines of a line are proportional to direction ratios of the line, if l, m, n are the direction cosines of the line, then the Cartesian equations of the line are

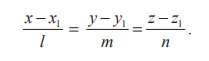
(iii) In (5), if any one or two of b1, b2 , b3 are zero, it does not mean that we are dividing by zero. But it means that the corresponding numerator is zero. For instance, If b1 â‰  0, b2 â‰  0 and b3 = 0 , then 

(iv) We know that the direction cosines of x - axis are 1, 0,0 . Therefore, the equations of x -axis are 

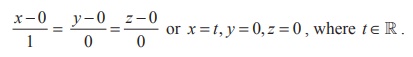
Similarly the equations of y -axis and z -axis are given by 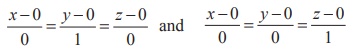 respectively.