Definition, Theorem, Proof, Solved Example Problems, Solution - Distance between two parallel planes | 12th Mathematics : UNIT 6 : Applications of Vector Algebra
Chapter: 12th Mathematics : UNIT 6 : Applications of Vector Algebra
Distance between two parallel planes
Distance between two parallel planes
Theorem 6.21
The distance between two parallel planes ax + by + cz
+ d1 = 0 and ax + by + cz + d2
= 0 is given by
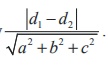
Proof
Let A( x1 , y1 , z1
) be any point on the plane ax + by + cz + d2
= 0 , then we have
ax1 + by1 + cz1 + d2
= 0 ⇒ ax1 + by1 + cz1
= −d2
The distance of the plane ax + by + cz + d1
= 0 from the point A( x1 , y1 , z1
) is given by
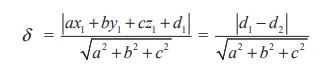
Hence, the distance between two parallel planes ax + by + cz + d1 = 0 and ax + by + cz + d2 = 0 given
by δ = 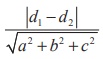 .
.
Example 6.51
Find the distance between the parallel planes x + 2 y −
2z +1 = 0 and 2x + 4 y − 4z + 5 = 0
Solution
We know that the formula for the distance between two parallel
planes ax + by + cz + d1 = 0 and ax +
by + cz + d2 = 0 is  Rewrite the second
equation as x + 2y – 2z + 5/2 = 0.
Comparing the given equations with the general equations, we get a = 1, b
= 2, c = −2, d1=1, d2 = 5/2.
Rewrite the second
equation as x + 2y – 2z + 5/2 = 0.
Comparing the given equations with the general equations, we get a = 1, b
= 2, c = −2, d1=1, d2 = 5/2.
Substituting these values in the formula, we get the distance

Example 6.52
Find the distance between the planes ⋅

Solution
Let ![]() be the position vector of an arbitrary
point on the plane
be the position vector of an arbitrary
point on the plane ![]() ⋅(2 ˆi − ˆj −
2 ˆk ) = 6 .
Then, we have
⋅(2 ˆi − ˆj −
2 ˆk ) = 6 .
Then, we have
![]() ⋅(2 ˆi − ˆj − 2 ˆk ) = 6 .................(1)
⋅(2 ˆi − ˆj − 2 ˆk ) = 6 .................(1)
If δ is the distance between the given planes, then δ is
the perpendicular distance from ![]() to the plane
to the plane
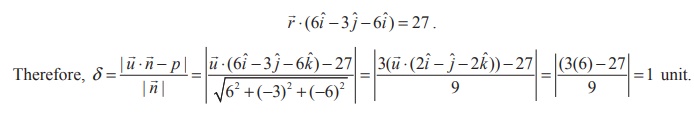
Related Topics