Definition, Theorem, Proof, Solved Example Problems, Solution - Application of Vectors to 3-Dimensional Geometry | 12th Mathematics : UNIT 6 : Applications of Vector Algebra
Chapter: 12th Mathematics : UNIT 6 : Applications of Vector Algebra
Application of Vectors to 3-Dimensional Geometry
Application
of Vectors to 3-Dimensional Geometry
Vectors provide an elegant approach to study straight lines and
planes in three dimension. All straight lines and planes are subsets of R3. For brevity, we shall
call a straight line simply as line. A plane is a surface which is understood
as a set P of points in R3
such that , if A, B, and C are any three non-collinear
points of P , then the line passing through any two of them is a subset
of P. Two planes are said to be intersecting if they have at least one
point in common and at least one point which lies on one plane but not on the
other. Two planes are said to be coincident if they have exactly the same
points. Two planes are said to be parallel but not coincident if they have no
point in common. Similarly, a straight line can be understood as the set of
points common to two intersecting planes. In this section, we obtain vector and
Cartesian equations of straight line and plane by applying vector methods. By a
vector form of equation of a geometrical object, we mean an equation which is
satisfied by the position vector of every point of the object. The equation may
be a vector equation or a scalar equation.
1. Different forms of equation of a straight line
A straight line can be uniquely fixed if
·
a point on the straight line and the direction of the straight
line are given
·
two points on the straight line are given
We find equations of a straight line in vector and Cartesian
form. To find the equation of a straight line in vector form, an arbitrary
point P with position vector ![]() on the straight line is
taken and a relation satisfied by
on the straight line is
taken and a relation satisfied by ![]() is obtained by using the
given conditions. This relation is called the vector equation of the straight
line. A vector equation of a straight line may or may not involve parameters.
If a vector equation involves parameters, then it is called a vector equation in
parametric form. If no parameter is involved, then the equation is called a vector equation in non
– parametric form.
is obtained by using the
given conditions. This relation is called the vector equation of the straight
line. A vector equation of a straight line may or may not involve parameters.
If a vector equation involves parameters, then it is called a vector equation in
parametric form. If no parameter is involved, then the equation is called a vector equation in non
– parametric form.
2. A point on the straight line and the direction of the straight line are given
(A) Parametric form of vector equation
Theorem 6.11
The vector equation of a straight line passing through a fixed
point with position vector ![]() and parallel to a given vector
and parallel to a given vector ![]() is
is ![]() =
= ![]() + t
+ t![]() , where t
∈ R.
, where t
∈ R.
Proof
If ![]() is the position vector of a given point A
and
is the position vector of a given point A
and ![]() is the position vector of an arbitrary point P
on the straight line, then
is the position vector of an arbitrary point P
on the straight line, then
![]() =
= ![]() -
- ![]() .
.
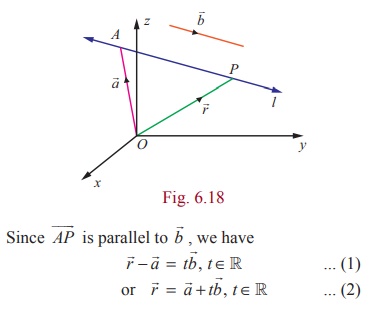
This is the vector equation of the straight line in parametric
form.
Remark
The position vector of any point on the line is taken as ![]() +
t
+
t![]() .
.
(b) Non-parametric form of vector equation
Since ![]() is parallel to
is parallel to ![]() , we have
, we have ![]() ×
× ![]() =
= ![]()
That is, (![]() −
− ![]() ) ×
) × ![]() = 0 .
= 0 .
This is known as the vector equation of the straight line
in non-parametric form.
(c) Cartesian equation
Suppose P is (x, y, z) , A is
(x1 , y1 , z1 ) and ![]() =
b1 ˆi
+ b2 ˆj + b3 ˆk . Then, substituting
=
b1 ˆi
+ b2 ˆj + b3 ˆk . Then, substituting ![]() = x ˆi + y ˆj + z ˆk ,
= x ˆi + y ˆj + z ˆk , ![]() = x1ˆi + y1ˆ j + z1 ˆk in (1) and comparing
the coefficients of ˆi ,
ˆj, ˆk , we get
= x1ˆi + y1ˆ j + z1 ˆk in (1) and comparing
the coefficients of ˆi ,
ˆj, ˆk , we get
x − x1 = tb1 , y − y1
= tb2 , z − z1 = tb3 ………….(4)
Conventionally (4) can be written as
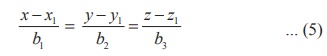
which are called the Cartesian equations or symmetric equations of a straight line
passing through the point (x1, y1 , z1)
and parallel to a vector with direction ratios b1, b2,
b3.
Remark
(i) Every point on the line (5) is of the form
(x1 + tb1 , y1 + tb2
, z1 + tb3) , where t ∈ R.
(ii) Since the direction cosines of a line are
proportional to direction ratios of the line, if l, m, n are the direction
cosines of the line, then the Cartesian equations of the line are
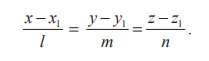
(iii) In (5), if any one or two of b1, b2
, b3 are zero, it does not mean that we are dividing by zero.
But it means that the corresponding numerator is zero. For instance, If b1
≠ 0, b2 ≠ 0 and b3 = 0 , then

(iv) We know that the direction cosines of x - axis are
1, 0,0 . Therefore, the equations of x -axis are
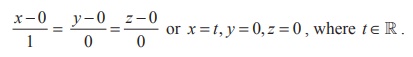
Similarly the equations of y
-axis and z -axis are given by 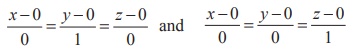 respectively.
respectively.
3. Straight Line passing through two given points
(a) Parametric form of vector equation
Theorem 6.12
The parametric form of vector equation of a line passing through
two given points whose position vectors are ![]() and
and ![]() respectively
is
respectively
is 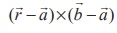 , t ∈ R.
, t ∈ R.
(b) Non-parametric form of vector equation
The above equation can be written equivalently in non-parametric form of vector equation as
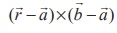 =
= ![]()
(c) Cartesian form of equation
Suppose P is (x, y, z) , A is
(x1, y1 , z1 ) and B is
(x2 , y2 , z2). Then
substituting ![]() = x ˆi + y ˆ j + z ˆk ,
= x ˆi + y ˆ j + z ˆk , ![]() = x1ˆi +
y1ˆ j + z1ˆk and
= x1ˆi +
y1ˆ j + z1ˆk and ![]() =
x2i + y2 ˆ j + z2ˆk
in theorem 6.12 and comparing the coefficients of ˆi , ˆ j,
ˆk , we get x − x1 = t(x2
− x1), y − y1 = t( y2
− y1), z − z1 = t(z2
− z1 ) and so the Cartesian equations of a line passing
through two given points (x1, y1, z1)
and (x2, y2, z2) are given by
=
x2i + y2 ˆ j + z2ˆk
in theorem 6.12 and comparing the coefficients of ˆi , ˆ j,
ˆk , we get x − x1 = t(x2
− x1), y − y1 = t( y2
− y1), z − z1 = t(z2
− z1 ) and so the Cartesian equations of a line passing
through two given points (x1, y1, z1)
and (x2, y2, z2) are given by

From the above equation, we observe that the direction ratios of a
line passing through two given points (x1 , y1
, z1) and (x2 , y2 , z2
) are given by x2 − x1 , y2
− y1 , z2 − z1, which are
also given by any three numbers proportional to them and in particular x1
− x2 , y1 − y2 , z1
− z2.
Example 6.24
A straight line passes through the point (1, 2, −3) and parallel to 4iˆ
+ 5 ˆj − 7kˆ . Find (i) vector equation in parametric form (ii)
vector equation in non-parametric form (iii) Cartesian equations of the
straight line.
Solution
The required line passes through (1, 2, −3) . So, the position
vector of the point is iˆ + 2 ˆj − 3kˆ.
Let ![]() = ˆi + 2 ˆj − 3ˆk and
= ˆi + 2 ˆj − 3ˆk and ![]() = 4ˆi + 5 ˆj − 7ˆk . Then, we have
= 4ˆi + 5 ˆj − 7ˆk . Then, we have
Let a = i + 2 j - 3k and b = 4i + 5 j - 7k . Then, we have
(i) vector equation of the required straight line in parametric
form is ![]() =
= ![]() + t
+ t![]() , t ∈ R.
, t ∈ R.
Therefore, ![]() = (ˆi +
2 ˆ j - 3 ˆk ) + t(4 ˆi + 5 ˆ j - 7 ˆ k ), t∈ R..
= (ˆi +
2 ˆ j - 3 ˆk ) + t(4 ˆi + 5 ˆ j - 7 ˆ k ), t∈ R..
(ii) vector equation of the
required straight line in non-parametric form is ( ![]() -
- ![]() ) ×
) × ![]() =
= ![]() .
.
Therefore, ( ![]() - (ˆi +
2 ˆj - 3 ˆk )) × (4 ˆi + 5 ˆj - 7 ˆk ) =
- (ˆi +
2 ˆj - 3 ˆk )) × (4 ˆi + 5 ˆj - 7 ˆk ) = ![]() .
.
(iii) Cartesian equations of
the required line are (x - x1)
/ b1 = y - y1 / b1 = (z - z1) / b1.
Here, (x1 , y1 , z1) = (1, 2, -3) and direction ratios of the required line are proportional to 4, 5, -7 . Therefore, Cartesian equations of the straight line are (x -1)/4 = (y – 2)/5 = (z + 3)/-7.
Example 6.25
The vector equation in parametric form of a line is ![]() = (3 ˆi − 2 ˆj + 6 ˆk ) + t(2 ˆi − ˆj
+ 3 ˆk ) . Find (i) the direction cosines of the straight line (ii)
vector equation in non-parametric form of the line (iii)Cartesian equations of
the line.
= (3 ˆi − 2 ˆj + 6 ˆk ) + t(2 ˆi − ˆj
+ 3 ˆk ) . Find (i) the direction cosines of the straight line (ii)
vector equation in non-parametric form of the line (iii)Cartesian equations of
the line.
Solution
Comparing the given equation with equation of a straight line ![]() =
= ![]() + t
+ t![]() , we have
, we have ![]() = 3 ˆi − 2 ˆj
+ 6 ˆk and
= 3 ˆi − 2 ˆj
+ 6 ˆk and ![]() = 2iˆ − ˆj + 3kˆ . Therefore,
= 2iˆ − ˆj + 3kˆ . Therefore,
(i) If ![]() = b1iˆ + b2ˆj + b3kˆ
, then
direction ratios of the straight
line are b1 , b2
, b3. Therefore, direction ratios of the given straight line are
proportional to 2, -1, 3 , and hence the direction cosines of the given
straight line are
= b1iˆ + b2ˆj + b3kˆ
, then
direction ratios of the straight
line are b1 , b2
, b3. Therefore, direction ratios of the given straight line are
proportional to 2, -1, 3 , and hence the direction cosines of the given
straight line are 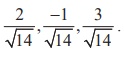 .
.
(ii)
vector equation of the straight line in non-parametric form is given by ( ![]() -
- ![]() ) ×
) × ![]() =
= ![]() . Therefore,
(
. Therefore,
( ![]() - (3 ˆi - 2 ˆj + 6 ˆk )) x(2 ˆi - ˆj + 3 ˆk ) = 0 .
- (3 ˆi - 2 ˆj + 6 ˆk )) x(2 ˆi - ˆj + 3 ˆk ) = 0 .
(iii) Here (x1 , y1 , z1
) = (3, -2, 6) and the direction ratios are proportional to 2, -1, 3 .
Therefore,
Cartesian equations of the straight line are (x – 3)/2 = (y + 2)/-1 = (z – 6)/3
Example 6.26
Find the vector equation in parametric form and Cartesian
equations of the line passing through (−4, 2, −3) and is parallel to the line 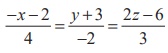
Solution
Rewriting the given equations as  and comparing with
and comparing with 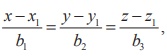
We have 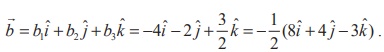
Clearly, ![]() is parallel to the vector 8iˆ + 4ˆj - 3kˆ . Therefore, a vector equation of the
required straight line passing through the given point (-4, 2, -3) and parallel
to the vector 8iˆ + 4ˆj - 3kˆ
in parametric form is
is parallel to the vector 8iˆ + 4ˆj - 3kˆ . Therefore, a vector equation of the
required straight line passing through the given point (-4, 2, -3) and parallel
to the vector 8iˆ + 4ˆj - 3kˆ
in parametric form is
![]() = (-4iˆ + 2ˆj - 3kˆ) + t(8iˆ + 4ˆj - 3kˆ),
t ∈ R.
= (-4iˆ + 2ˆj - 3kˆ) + t(8iˆ + 4ˆj - 3kˆ),
t ∈ R.
Therefore, Cartesian equations of the required straight line are
given by
(x + 4) / 8 = (y – 2) / 4 =
(z + 3) / -3 .
Example 6.27
Find the vector equation in parametric form and Cartesian
equations of a straight passing through the points (−5, 7, −4) and (13, −5, 2)
. Find the point where the straight line crosses the xy -plane.
Solution
The straight line passes through the points (−5, 7, −4) and (13,
−5, 2) , and therefore, direction ratios of the straight line joining these two
points are 18, −12, 6 . That is 3, −2,1.
So, the straight line is parallel to 3iˆ − 2 ˆj + kˆ
. Therefore,
Required vector equation of the straight line in parametric form
is ![]() = (−5ˆi + 7 ˆj − 4ˆk ) + t(3ˆi − 2ˆj + ˆk ) or
= (−5ˆi + 7 ˆj − 4ˆk ) + t(3ˆi − 2ˆj + ˆk ) or ![]() = (13ˆi − 5ˆj + 2ˆk ) + s(3ˆi − 2ˆj + ˆk ) where s, t ∈ R.
= (13ˆi − 5ˆj + 2ˆk ) + s(3ˆi − 2ˆj + ˆk ) where s, t ∈ R.
Required cartesian equations of the straight line are 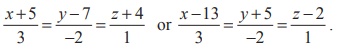
An arbitrary point on the straight line is of the form
Since the straight line crosses the xy -plane, the z -coordinate of the point of intersection is zero. Therefore, we have t − = 4 0 , that is, t = 4, and hence the straight line crosses the xy -plane at (7,−1,0).
Example 6.28
Find the angles between the straight line 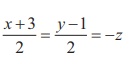 with
coordinate axes.
with
coordinate axes.
Solution
If bˆ is a unit vector
parallel to the given line, then bˆ = 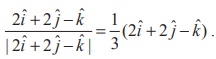 Therefore, from the definition of direction cosines of bˆ , we have
Therefore, from the definition of direction cosines of bˆ , we have

where α , β ,γ are the angles made by bˆ with the positive x -axis, positive y -axis, and positive z
-axis, respectively. As the angle between the given straight line with the
coordinate axes are same as the angles made by bˆ with the coordinate axes, we have α = cos-1 (2/3), β
= cos-1( 2/3), γ = cos-1(-1/3), respectively.
4. Angle between two straight lines
(a) Vector form
The acute angle between two given straight lines
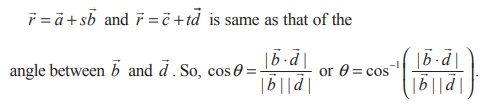
(b) Cartesian form
If two lines are given in Cartesian form as 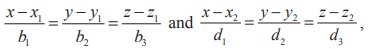 then the
acute angle θ between the two given lines is given by
then the
acute angle θ between the two given lines is given by
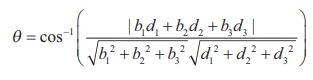
Remark
(i) The two given lines with direction ratios b1 , b2
, b3 and d1 , d2 , d3 are parallel
if, and only if 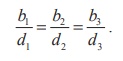 .
.
(ii) The two given lines
with direction ratios b1, b2, b3 and d1,
d2, d3 are perpendicular if and only if b1d1 + b2d2 + b3d3
= 0 .
(iii) If the direction cosines of two given straight lines are l1 , m1 , n1 and l2 , m2 , n2, then the angle between the two given straight lines is cos θ =| l1l2 + m1m2 + n1n2 | .
Example 6.29
Find the acute angle between the lines ![]() = (ˆi + 2ˆj + 4ˆk ) + t(2ˆi + 2ˆj + ˆk ) and the straight line
passing through the points (5,1, 4) and (9, 2,12) .
= (ˆi + 2ˆj + 4ˆk ) + t(2ˆi + 2ˆj + ˆk ) and the straight line
passing through the points (5,1, 4) and (9, 2,12) .
Solution
We know that the line ![]() = (ˆi + 2ˆj + 4ˆk ) + t(2ˆi + 2ˆj + ˆk ) is parallel to the
vector 2ˆi + 2ˆj + ˆk.
= (ˆi + 2ˆj + 4ˆk ) + t(2ˆi + 2ˆj + ˆk ) is parallel to the
vector 2ˆi + 2ˆj + ˆk.
Direction ratios of the straight line joining the two given points
(5,1, 4) and (9, 2,12) are 4,1,8 and hence this line is parallel to the vector
4iˆ + ˆj + 8kˆ .
Therefore, the acute angle between the given two straight lines
is

Example 6.30
Find the acute angle between the straight lines 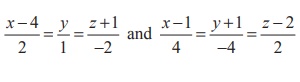 and state whether they are parallel or perpendicular.
and state whether they are parallel or perpendicular.
Solution
Comparing the given lines with the general Cartesian equations
of straight lines,
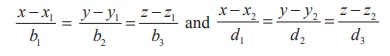
we find (b1 , b2 , b3
) = (2,1, −2) and (d1 , d2 , d3
) = (4, −4, 2) . Therefore, the acute angle between the two straight lines is
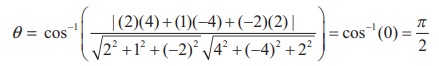
Thus the two straight lines are perpendicular.
Example 6.31
Show that the straight line passing through the points A(6,
7, 5) and B(8,10, 6) is perpendicular to the straight line passing
through the points C(10, 2, −5) and D(8, 3, −4) .
Solution
The straight line passing through the points A(6, 7, 5)
and B(8,10, 6) is parallel to the vector ![]() =
= ![]() =
= ![]() −
− ![]() = 2iˆ + 3 ˆj + kˆ and the straight
line passing through the points C(10, 2, −5) and D(8, 3, −4) is
parallel to the vector
= 2iˆ + 3 ˆj + kˆ and the straight
line passing through the points C(10, 2, −5) and D(8, 3, −4) is
parallel to the vector ![]() =
= ![]() = −2iˆ + ˆj
+ kˆ . Therefore, the angle between the two straight lines is the
angle between the two vectors
= −2iˆ + ˆj
+ kˆ . Therefore, the angle between the two straight lines is the
angle between the two vectors ![]() and
and ![]() .
Since
.
Since
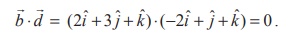
the two vectors are perpendicular, and hence the two straight
lines are perpendicular.
Aliter
We find that direction ratios of the straight line joining the
points A(6, 7, 5) and B(8,10, 6) are (b1 , b2
, b3 ) = (2, 3,1) and and direction ratios of the line joining the
points C(10, 2, −5) and D(8, 3, −4) are (d1 , d2
, d3 ) = (−2,1,1) . Since b1d1
+ b2d2 + b3d3
= (2)(−2) + (3)(1) + (1)(1) = 0 , the two straight lines are perpendicular.
Example 6.32
Show that the lines 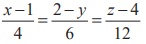 and
and 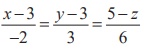 are parallel
are parallel
Solution
We observe that the straight line 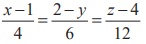 is parallel to
the vector 4iˆ - 6 ˆj +12kˆ
and the straight line
is parallel to
the vector 4iˆ - 6 ˆj +12kˆ
and the straight line 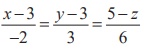 is parallel to the vector -2iˆ + 3ˆj - 6kˆ.
is parallel to the vector -2iˆ + 3ˆj - 6kˆ.
Since 4iˆ - 6ˆj +12kˆ = -2(-2iˆ + 3ˆj - 6kˆ) , the two vectors are parallel, and hence the two straight lines are parallel.
Related Topics