Solved Example Problems for Differential Calculus

Solved Example Problems for Differential Calculus

Example 2.18

Consider the function y = x 2 . Calculate the derivative dy/dx using the concept of limit.

Solution

Let us take two points given by

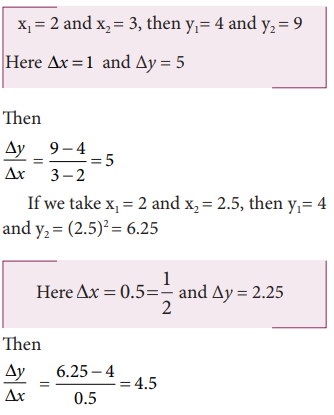
These results are tabulated as shown below:

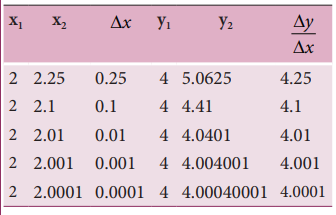
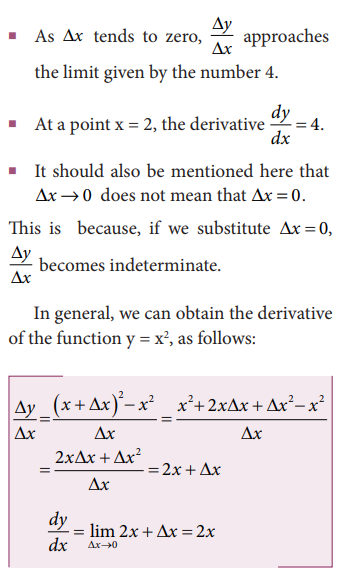
From the above table, the following inferences can be made.

Example 2.19

Find the derivative with respect to t, of the function x= A0 + A1t + A2 t2 where A0, A1 and A2 are constants.

Solution

Note that here the independent variable is ‘t’ and the dependent variable is ‘x’

The requived derivative is dx/dt = 0+ A1+2A2t

The second derivative is d2x/d2t = 2A2