Addition of Vectors (Triangular Law of addition method)

Addition of Vectors

Since vectors have both magnitude and direction they cannot be added by the method of ordinary algebra. Thus, vectors can be added geometrically or analytically using certain rules called ‘vector algebra’. In order to find the sum (resultant) of two vectors, which are inclined to each other, we use (i) Triangular law of addition method or (ii) Parallelogram law of vectors.

Triangular Law of a͢ddition method

Let us consider two vectors  and  as shown in Figure 2.16.

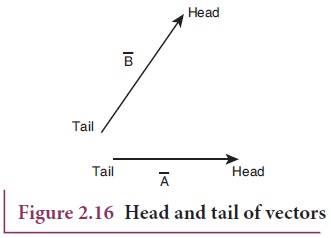
To find the resultant of the two vectors we apply the triangular law of addition as follows:

Represent the vectors  and  by the two adjacent sides of a triangle taken in the same order. Then the resultant is given by the third side of the triangle as shown in Figure 2.17.

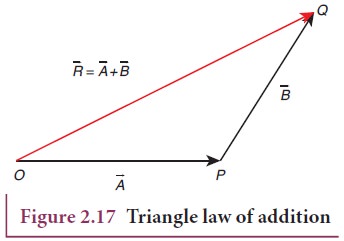
To explain further, the head of the first vector  is connected to the tail of the second vector . Let θ be the angle between  and . Then  is the resultant vector connecting the tail of the first vector  to the head of the second vector . The magnitude of  (resultant) is given geometrically by the length of  (OQ) and the direction of the resultant vector is the angle between  and . Thus we write = .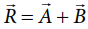

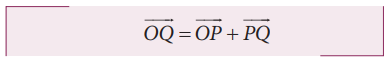
1. Magnitude of resultant vector

The magnitude and angle of the resultant vector are determined as follows.

From Figure 2.18, consider the triangle ABN, which is obtained by extending the side OA to ON. ABN is a right angled triangle.

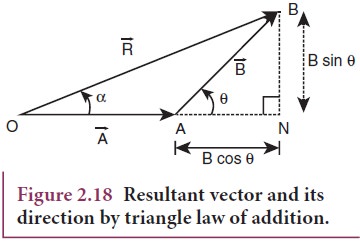
From Figure 2.18

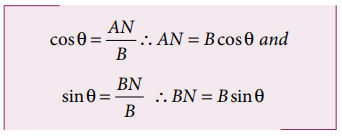
For ∆OBN, we have OB2 = ON 2 + BN 2

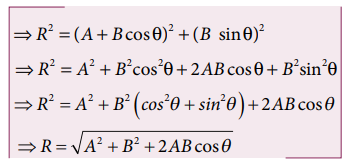
which is the magnitude of the resultant of  and 

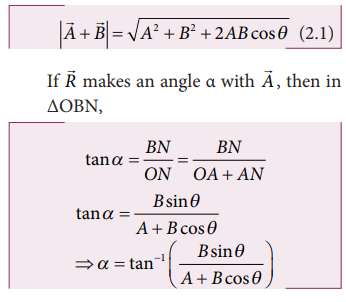
2. Direction of resultant vectors:

If θ is the angle between  and  , then

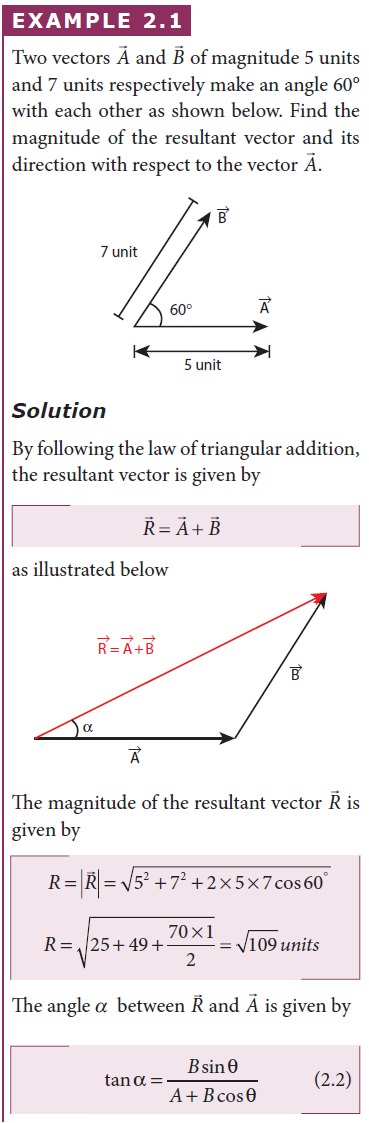
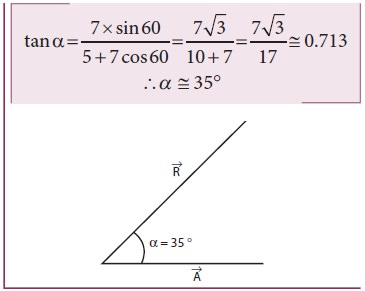
Solved Example Problem for Addition of Vectors