Projectile Motion

PROJECTILE MOTION

Introduction

When an object is thrown in the air with some initial velocity (NOT just upwards), and then allowed to move under the action of gravity alone, the object is known as a projectile. The path followed by the particle is called its trajectory.

Examples of projectile are

1. An object dropped from window of a moving train.

2. A bullet fired from a rifle.

3. A ball thrown in any direction.

4. A javelin or shot put thrown by an athlete.

5. A jet of water issuing from a hole near the bottom of a water tank.

It is found that a projectile moves under the combined effect of two velocities.

i. A uniform velocity in the horizontal direction, which will not change provided there is no air resistance.

ii. A uniformly changing velocity (i.e., increasing or decreasing) in the vertical direction.

There are two types of projectile motion:

i. Projectile given an initial velocity in the horizontal direction (horizontal projection)

ii. Projectile given an initial velocity at an angle to the horizontal (angular projection)

To study the motion of a projectile, let us assume that,

i. Air resistance is neglected.

ii. The effect due to rotation of Earth and curvature of Earth is negligible.

iii. The acceleration due to gravity is constant in magnitude and direction at all points of the motion of the projectile.

Projectile in horizontal projection

Consider a projectile, say a ball, thrown horizontally with an initial velocity  from the top of a tower of height h (Figure 2.39).

As the ball moves, it covers a horizontal distance due to its uniform horizontal velocity u, and a vertical downward distance because of constant acceleration due to gravity g. 

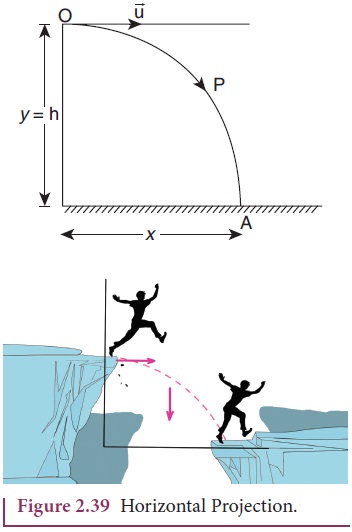
Thus, under the combined effect the ball moves along the path OPA. The motion is in a 2-dimensional plane. Let the ball take time t to reach the ground at point A, Then the horizontal distance travelled by the ball is x (t) = x, and the vertical distance travelled is y (t) = y

We can apply the kinematic equations along the x direction and y direction separately. Since this is two-dimensional motion, the velocity will have both horizontal component u_x and vertical component u_y.

Motion along horizontal direction

The particle has zero acceleration along x direction. So, the initial velocity u_x remains constant throughout the motion

The distance traveled by the projectile at a time t is given by the equation x = u_xt + 1/2 at². Since a = 0 along x direction, we have

Motion along downward direction

Here u_y = 0 (initial velocity has no downward component), a = g (we choose the +ve y-axis in downward direction), and distance y at time t

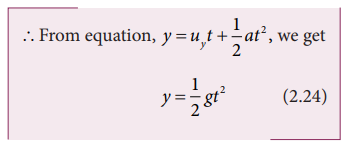
Substituting the value of t from equation (2.23) in equation (2.24) we have

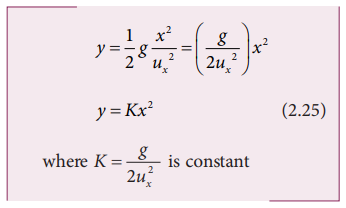
Equation (2.25) is the equation of a parabola. Thus, the path followed by the projectile is a parabola (curve OPA in the Figure 2.39).

(1) Time of Flight: The time taken for the projectile to complete its trajectory or time taken by the projectile to hit the ground is called time of flight.

Consider the example of a tower and projectile. Let h be the height of a tower. Let T be the time taken by the projectile to hit the ground, after being thrown horizontally from the tower.

We know that s _y = u_yt + 1/2 at² for vertical motion. Here s_y = h, t = T, u_y = 0 (i.e., no initial vertical velocity). Then

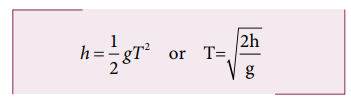
Thus, the time of flight for projectile motion depends on the height of the tower, but is independent of the horizontal velocity of projection. If one ball falls vertically and another ball is projected horizontally with some velocity, both the balls will reach the bottom at the same time. This is illustrated in the Figure 2.40.

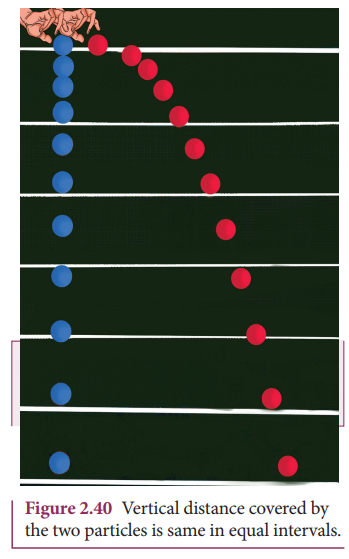
(2) Horizontal range: The horizontal distance covered by the projectile from the foot of the tower to the point where the projectile hits the ground is called horizontal range. For horizontal motion, we have

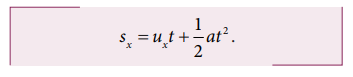
Here, s_x = R (range), u_x = u, a = 0 (no horizontal acceleration) T is time of flight. Then horizontal range = uT.

The above equation implies that the range R is directly proportional to the initial velocity u and inversely proportional to acceleration due to gravity g.

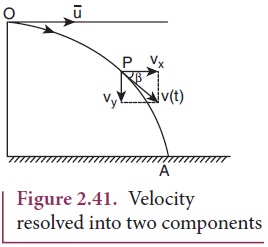
(3) Resultant Velocity (Velocity of projectile at any time): At any instant t, the projectile has velocity components along both x-axis and y-axis. The resultant of these two components gives the velocity of the projectile at that instant t, as shown in Figure 2.41.

The velocity component at any t along horizontal (x-axis) is v_x = u_x + a _x t

Since, u_x = u, a_x = 0 , we get

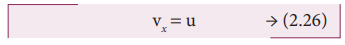
The component of velocity along vertical direction (y-axis) is v _y = u_y + a _y t

Since, u_y = 0, a_y = g, we get

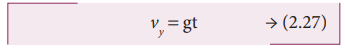
Hence the velocity of the particle at any instant is

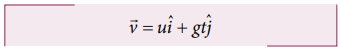
The speed of the particle at any instant t is given by

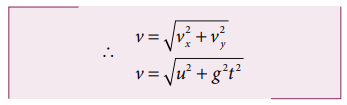
(4) Speed of the projectile when it hits the ground: When the projectile hits the ground after initially thrown horizontally from the top of tower of height h, the time of flight is

The horizontal component velocity of the projectile remains the same i.e v_x= u

The vertical component velocity of the projectile at time T is

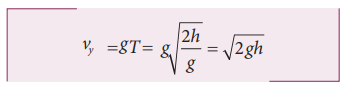
The speed of the particle when it reaches the ground is

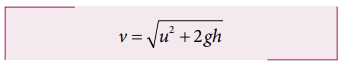
Projectile under an angular projection

This projectile motion takes place when the initial velocity is not horizontal, but at some angle with the vertical, as shown in Figure 2.42.

(Oblique projectile)

Examples :

·           Water ejected out of a hose pipe held obliquely.

·           Cannon fired in a battle ground.

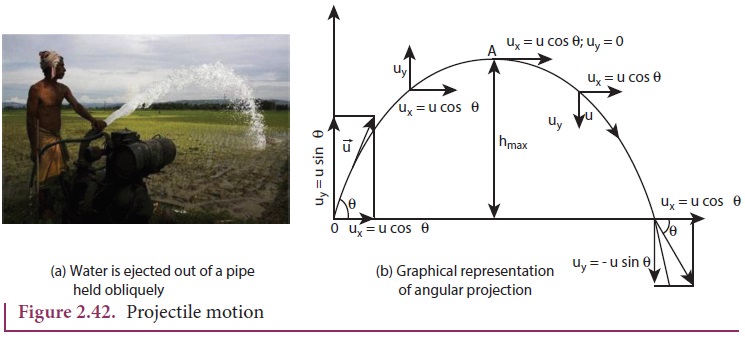
Consider an object thrown with initial velocity  at an angle θ  with the horizontal. Refer Figures 2.42 and 2.43.

Then

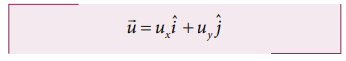
where u_x = u cosθ is the horizontal component and u_y = usinθ the vertical component of velocity.

Since the acceleration due to gravity is in the direction opposite to the direction of vertical component u_y, this component will gradually reduce to zero at the maximum height of the projectile. At this maximum height, the same gravitational force will push the projectile to move downward and fall to the ground. There is no acceleration along the x direction throughout the motion. So, the horizontal component of the velocity (u_x = u cosθ) remains the same till the object reaches the ground.

Hence after the time t, the velocity along horizontal motion v_x = u_x+ a_xt = u_x = u cos θ

The horizontal distance travelled by projectile in time t is s_x = u_xt + 1/2 a_xt²

Here, s_x = x, u_x = u cosθ, a_x = 0

Next, for the vertical motion v_y = u_y+ a_yt

Here u_y= u sinθ, a_y = - g (acceleration due to gravity acts opposite to the motion). Thus

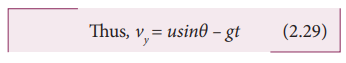
The vertical distance travelled by the projectile in the same time t is s_y = u_yt + 1/2 a_yt²

Here, s_y = y, u_y = u sinθ, a_x =-g. Then

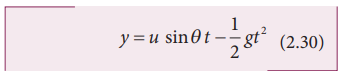
Substitute the value of t from equation (2.28) in equation (2.30), we have

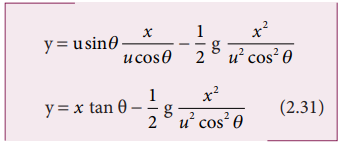
Thus the path followed by the projectile is an inverted parabola .

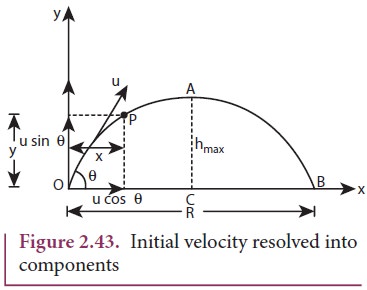
Maximum height (h_max)

The maximum vertical distance travelled by the projectile during its journey is called maximum height. This is determined as follows:

For the vertical part of the motion,

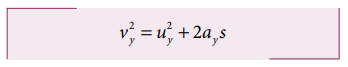
Here, u_y = u sinθ, a =-g, s = h_max, and at the maximum height v_y = 0

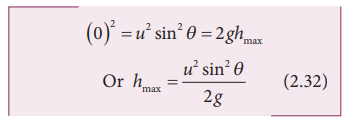
Time of flight (T_f)

The total time taken by the projectile from the point of projection till it hits the horizontal plane is called time of flight.

This time of flight is the time taken by the projectile to go from point O to B via point A (Figure 2.43)

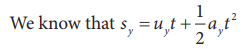
Here, s_y = y = 0 (net displacement in y-direction is zero), u_y = u sinθ, a_y = -g, t = T_f  Then

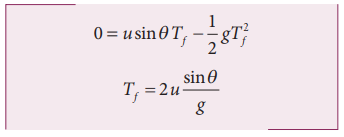
Horizontal range (R)

The maximum horizontal distance between the point of projection and the point on the horizontal plane where the projectile hits the ground is called horizontal range (R). This is found easily since the horizontal component of initial velocity remains the same. We can write

Range R = Horizontal component of velocity x time of flight = u cosθ ×T_f

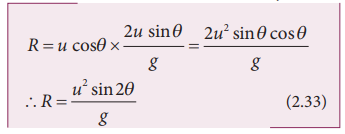
The horizontal range directly depends on the initial speed (u) and the sine of angle of projection (θ ). It inversely depends on acceleration due to gravity ‘g’

For a given initial speed u, the maximum possible range is reached when sin2θ is maximum, sin2θ = 1. This implies 2θ = π /2

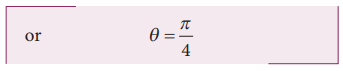
This means that if the particle is projected at 45 degrees with respect to horizontal, it attains maximum range, given by.

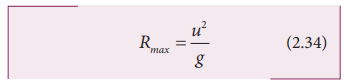
Solved Example Problems for Projectile Motion

Example 2.37

Suppose an object is thrown with initial speed 10 m s-1 at an angle π/4 with the horizontal, what is the range covered? Suppose the same object is thrown similarly in the Moon, will there be any change in the range? If yes, what is the change? (The acceleration due to gravity in the Moon gmoon = 1/6 g)

Solution

In projectile motion, the range of particle is given by,

If the same object is thrown in the Moon, the range will increase because in the Moon, the acceleration due to gravity is smaller than g on Earth,

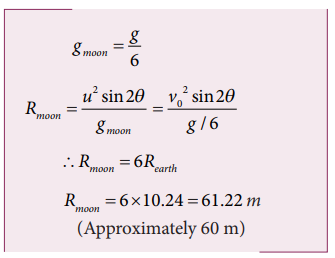
The range attained on the Moon is approximately six times that on Earth.

Example 2.38

In the cricket game, a batsman strikes the ball such that it moves with the speed 30 m s-1  at an angle 30o with the horizontal as shown in the figure. The boundary line of the cricket ground is located at a distance of 75 m from the batsman? Will the ball go for a six? (Neglect the air resistance and take acceleration due to gravity g = 10 m s-2).

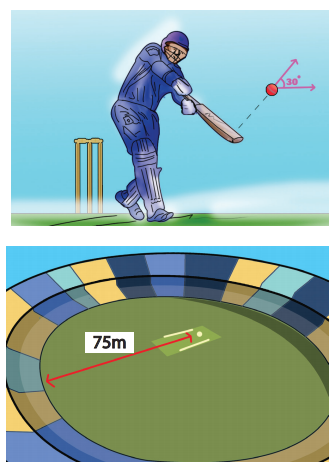
Solution

The motion of the cricket ball in air is essentially a projectile motion. As we have already seen, the range (horizontal distance) of the projectile motion is given by

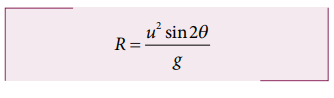
The initial speed u  30 m s-1

The projection angle θ = 30o

The horizontal distance travelled by the cricket ball

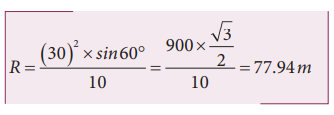
This distance is greater than the distance of the boundary line. Hence the ball will cross this line and go for a six.