Size and density of the nucleus

Size and density of the nucleus

The alpha particle scattering experiment and many other measurements using different methods have been carried out on the nuclei of various atoms. The nuclei are found to be approximately spherical in shape. It is experimentally found that radius of nuclei for Z > 10, satisfies the following empirical formula

Here A is the mass number of the nucleus and the constant R₀ = 1.2 F, where 1 F = 1 × 10^–15 m. The unit fermi (F) is named after Enrico Fermi.

EXAMPLE 8.7

Calculate the radius of ¹⁹⁷₇₉Au nucleus.

Solution

According to the equation (8.19),

R = 1.2 × 10^-15 × (197)^1/3 = 6.97 × 10^-15m

Or R = 6.97 F

EXAMPLE 8.8

Calculate the density of the nucleus with mass number A.

Solution

From equation (8.19), the radius of the nuclei satisfy the equation = R₀A^1/3. Then the volume of the nucleus

V = 4/3 Ï€R³ = 4/3 Ï€R³A

By ignoring the mass difference between the proton and neutron, the total mass of the nucleus having mass number A is equal to A.m where m is mass of the proton and is equal to 1.6726 x 10^-27 kg.

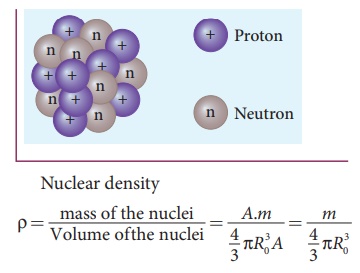
The above expression shows that the nuclear density is independent of the mass number A. In other words, all the nuclei (Z > 10) have the same density and it is an important characteristics of the nuclei.

We can calculate the numerical value of this density by substituting the corresponding values.

It implies that nucleons are extremely tightly packed in the nucleus and compare this density with the density of water which is 103 kg m^-3.

A single teaspoon of nuclear matter would weigh about trillion tons.