Exercise 3.10

Exercise 3.10

Miscellaneous Practice Problems

1. The sum of three numbers is 58. The second number is three times of two-fifth of the first number and the third number is 6 less than the first number. Find the three numbers.

Solution:

Here what we know

a + b + c = 58 (sum of three numbers is 58)

Let the first number be ‘x’

b = a + 3 (the second number is three times of 2/5 of the first number)

b = 3 × (2/5) x = (6/5) x

Third number = x − 6

Sum of the numbers is given as 58.

∴ x + 6/5 x + (x − 6) = 58

Multiplying by 5 throughout, we get

5 × x + 6x + 5 × (x – 6) = 58 × 5

5x + 6x + 5x – 30 = 290

∴ 16x = 290 + 30

∴ 16x = 320

∴ x = (320/16) x = 20

Answer:

1^st number = 20

2^nd number = 3 × 2/5 × 20 = 24

3^rd number = 24 – 6 = 14

2. In triangle ABC, the measure of ∠B is two-third of the measure of ∠A. The measure of ∠C is 20° more than the measure of ∠A. Find the measures of the three angles.

Solution:

Let angle ∠A be a°

Given that ∠B = 2/3 × ∠A = 2/3 a

& given ∠C = ∠A + 20 = a + 20

Since A, B & C are angles of a triangle, they add up to 180° (Δ property)

∴ ∠A +∠B + ∠C = 180°

⇒ a + 2/3 a + a + 20 = 180°

{[3a + 2a + 3a] / 3} + 20 =  180°

8a / 3 =  180 – 20 = 160

∴ a = [160 × 3] / 8 = 60°

∠B = 2/3 × ∠A = 2/3 × 60 = 40°

∠C = 80°

3. Two equal sides of an isosceles triangle are 5y−2 and 4y+9 units. The third side is 2y+5 units. Find ῾y᾿ and the perimeter of the triangle.

Solution:

Given that 5y − 2 & 4y + 9 are the equal sides of an isosceles triangle.

∴  The 2 sides are equal

=> 5y − 2 = 4y + 9  

∴  5y − 4y = 9 + 2 (by transposing)

∴ y = 11

∴  1^st side = 5y − 2 = 5 × 11 − 2 = 55 − 2 = 53

2^nd side = 53

3^rd side = 2y + 5 = 2 × 11 + 5 = 22 + 5 = 27

Perimeter is the sum of all 3 sides

∴  P = 53 + 53 + 27 = 133 units

4. In the given figure, angle XOZ and angle ZOY form a linear pair. Find the value of x.

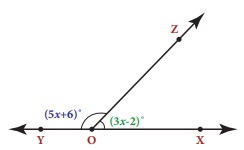
Solution:

Since ∠XOZ & ∠ZOY form a linear pair,

by property, we have their sum to be 180°

∴  ∠XOZ + ∠ZOY = 180°

∴ 3x − 2 + 5x + 6 = 180°

8x + 4 = 180 = 8x = 180 − 4

∴  8x = 176  ⇒ x = 176 / 8 ⇒ x = 22°

XOZ = 3x − 2 = 3 × 22 − 2 = 66 − 2 = 64°

YOZ = 5x + 6 = 5 × 22 + 6

= 110 + 6 = 116

5. Draw a graph for the following data:

Does the graph represent a linear relation?

Solution:

Graph between side of square & area

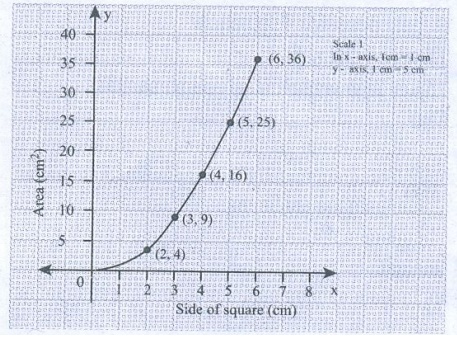
 When we plot the graph, we observe that it is not a linear relation.

Challenging Problems

6. Three consecutive integers, when taken in increasing order and multiplied by 2, 3 and 4 respectively, total up to 74. Find the three numbers.

Solution:

Let the 3 consecutive integers be ‘x’, ‘x + 1’ & ‘x + 2’

Given that when multiplied by 2, 3 & 4 respectively & added up, we get 74

i.e      [ 2 × x ] + [ 3 × (x + 1) ] + [ 4 (x + 2) ] = 74 

Simplifying the equation, we get

2x + 3x + 3 + 4x + 8 = 74

9x + 11 = 74

9x = 63  ⇒  x = 63 / 9 = 7

First number = 7

Second numbers = x + 1  ⇒ 7 + 1 = 8

Third numbers = x + 2  ⇒ 7 + 2 = 9

∴ The numbers are 7, 8 & 9

7. 331 students went on a field trip. Six buses were filled to capacity and 7 students had to travel in a van. How many students were there in each bus?

Solution:

Let the number of students in each bus be ‘x’

∴ number of students in 6 buses = 6 × x = 6x

Apart from 6 buses, 7 students went in van

A total number of students is 331

∴ 6x + 7 = 331

∴ 6x = 331 − 7 = 324

∴ x = 324 / 6 = 54

∴ There are 54 students in each bus.

8. A mobile vendor has 22 items, some which are pencils and others are ball pens. On a particular day, he is able to sell the pencils and ball pens. Pencils are sold for ₹15 each and ball pens are sold at ₹20 each. If the total sale amount with the vendor is ₹380, how many pencils did he sell?

Solution:

Let vendor have ‘p’ number of pencils & ‘b’ number of ball pens

Given that total number of items is 22

∴ p + b = 22 ……..(I)

Pencils are sold for  ₹ 15 each & ball pens for  ₹ 20 each

total sale amount = 15 × p + 20 × b

= 15p + 20b which is given to be 380.

∴ 15p + 20b  = 380

Dividing by 5 throughout,

15p/5 + 20b/5 = 380 / 5  ⇒  3p + 4b  = 76 ………..(2)

Multiplying equation (1) by 3 we get

3 × p + 3 × b = 22 × 3

⇒  3p + 3b = 66 ………..(3)

Equation (2) − (3) gives

      3p + 4b = 76

 (−) 3p + 3b = 66

       0 + b  = 10

∴ b = 10

∴ p = 12

He sold 12 pencils

9. Draw the graph of the lines y = x, y = 2x, y = 3x and y = 5x on the same graph sheet. Is there anything special that you find in these graphs?

Solution:

(i) y = x, (ii) y = 2x, (iii) y = 3x  (iv) y = 5x

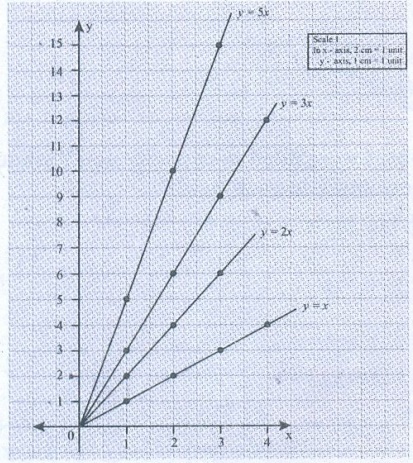
(i) y = x

 When x = 1, y = 1

x = 2, y = 2

x = 3, y = 2

(ii) y = 2x

 When x = 1, y = 2

 x = 2, y = 4

 x = 3, y = 6

(iii) y = 3x

 when x = 1, y = 3

 x = 2, y = 6

 x = 3, y = 9

(iv) y = 5x

 When x = 1, y = 5

 x = 2, y = 10

 x = 3, y = 15

When we plot the above points & join the points to form line, we notice that the lines become progressively steeper. In other words, the slope keeps increasing.

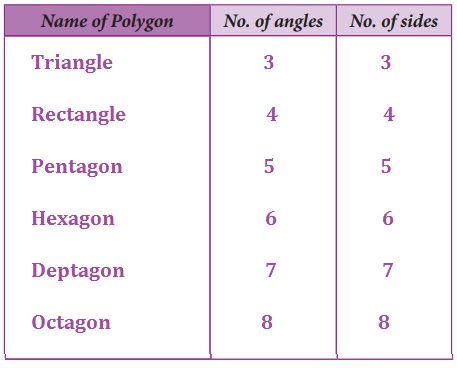
10. Consider the number of angles of a convex polygon and the number of sides of that polygon. Tabulate as follows:

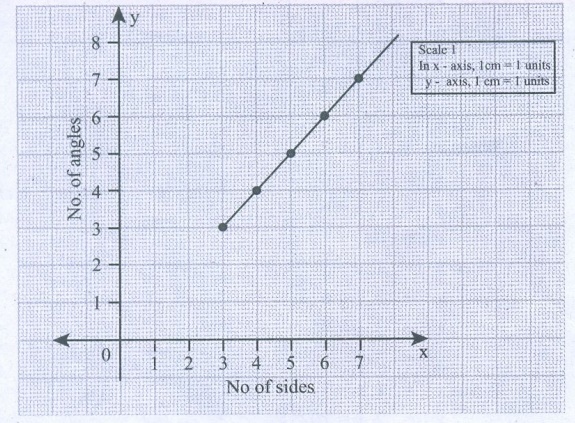
Use this to draw a graph illustrating the relationship between the number of angles and the number of sides of a polygon.

Solution:

Shapes: Trianlge, Rectangle, Pentagon, Hexagon

Angles :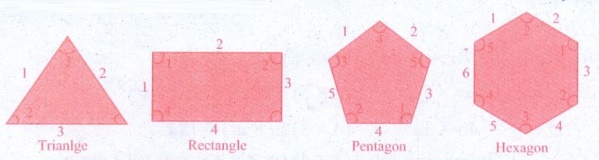 

Answer:

Exercise 3.10

Miscellaneous Practice Problems

1. x = 20

2. 60°, 40°, 80°

3. y = 11 units p=133 units

4. 116°,64°

Challenging Problems

6. 7,8,9

7. 54

8. 12 pencils