Cubic Identities

Cubic Identities

I. (a + b)³ = a³ + 3a²b + 3ab² + b³

We shall prove it now,

LHS = (a + b)³

= [(a + b)(a + b)](a + b) (expanded form)

= (a + b)² (a + b)

= (a ² + 2ab + b² )(a + b) (using identity)

= a(a² + 2ab + b² ) + b(a ² + 2ab + b² ) (using distributive law)

= a ³ + 2a²b + ab² + ba² + 2ab² + b³

= a³ + (2a²b + ba² ) + (ab² + 2ab² ) + b³ (grouping ‘like’ terms)

= a ³ + 3a²b + 3ab ² + b³

= RHS

(a + b)³ = a³ + 3a²b + 3ab² + b³

Hence, we proved the cubic identity by direct multiplication.

Activity

You can visualize the geometrical proof of (a+b)³ with the help of your teacher.

II. (a – b)³ = a³ – 3a²b + 3ab² – b³

We can prove this identity by direct multiplication

We have (a − b)³ = (a − b)(a − b)(a − b)

= (a − b)² × (a − b)

= (a ² − 2ab + b² )(a − b)

= a(a² − 2ab + b ² ) − b(a ² − 2ab + b² )

= a ³ − 2a²b + ab² − ba² + 2ab² − b³

= a³ - 2a²b - ba² + ab² + 2ab² - b³

= a³ − 3a²b + 3ab ² − b³

= RHS

(a – b)³ = a³ – 3a²b + 3ab² – b³

Hence, we proved.

a²b = ba²

Multiplication is commutative

III. (x + a)(x + b)(x + c) = x³ + (a + b + c)x² + (ab + bc + ca)x + abc

We know that the identity (x + a)(x + b) = x² + (a + b)x + ab . Let us multiply this by a binomial ( x + c) . Then we get.

(x + a)(x + b)(x + c ) = [( x + a)(x + b)](x + c)

= ( x² + (a + b)x + ab) × ( x + c)

= x [x² + (a + b)x + ab] + c[ x² + (a + b)x + ab ] (distributive law)

= x³ + (a + b )x² + abx + cx ² + (a + b)xc + abc

= x³ + ax ² + bx ² + abx + cx ² + acx + bcx + abc

= x³ + (a + b + c)x² + (ab + bc + ca )x + abc (Combine x² , x terms)

(x + a)(x + b)(x + c) = x³ + (a + b + c)x² + (ab + bc + ca)x + abc

Thus, we summarise the cubic identities as :

• (a + b)³ = a ³ + 3a²b + 3ab² + b³

• (a − b)³ = a ³ − 3a²b + 3ab² − b³

• (x + a)(x + b)(x + c) = x ³ + (a + b + c)x² + (ab + bc + ca )x + abc

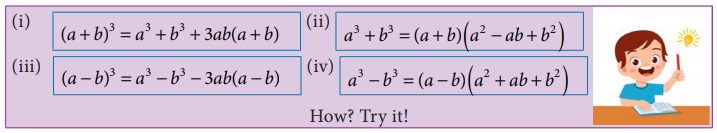
Deductions:

The above identities give the following deductions: