Exercise 1.7: Matrix: Homogeneous system of linear equations

EXERCISE 1.7

1. Solve the following system of homogenous equations.

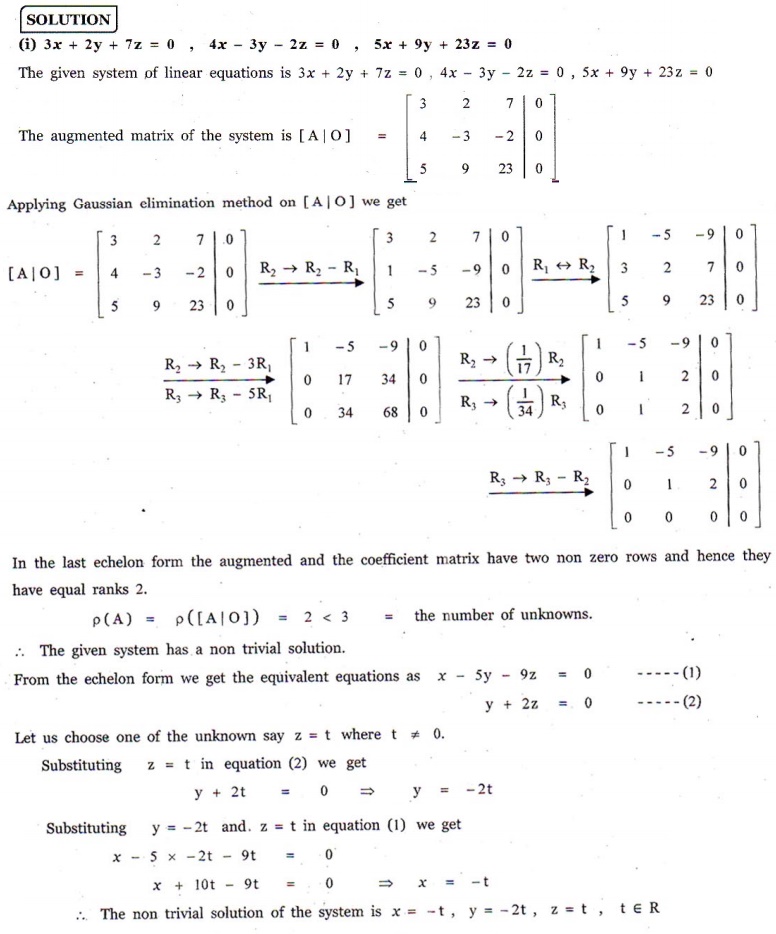
(i) 3x + 2 y + 7z = 0, 4x - 3y - 2z = 0, 5x + 9 y + 23z = 0

(ii) 2x + 3y - z = 0, x - y - 2z = 0, 3x + y + 3z = 0

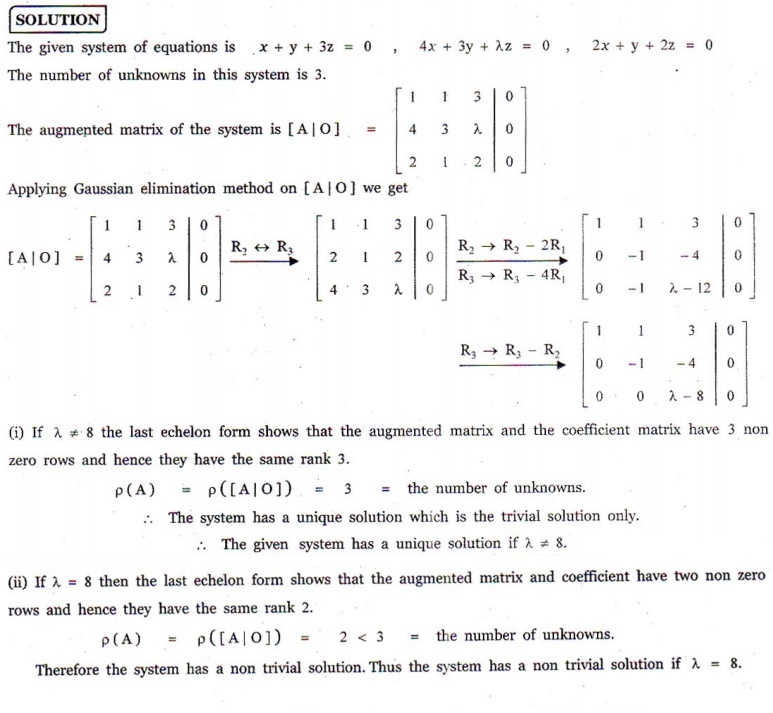
2. Determine the values of λ for which the following system of equations

x + y + 3z = 0, 4x + 3y + λz = 0, 2x + y + 2z = 0 has

(i) a unique solution

(ii) a non-trivial solution.

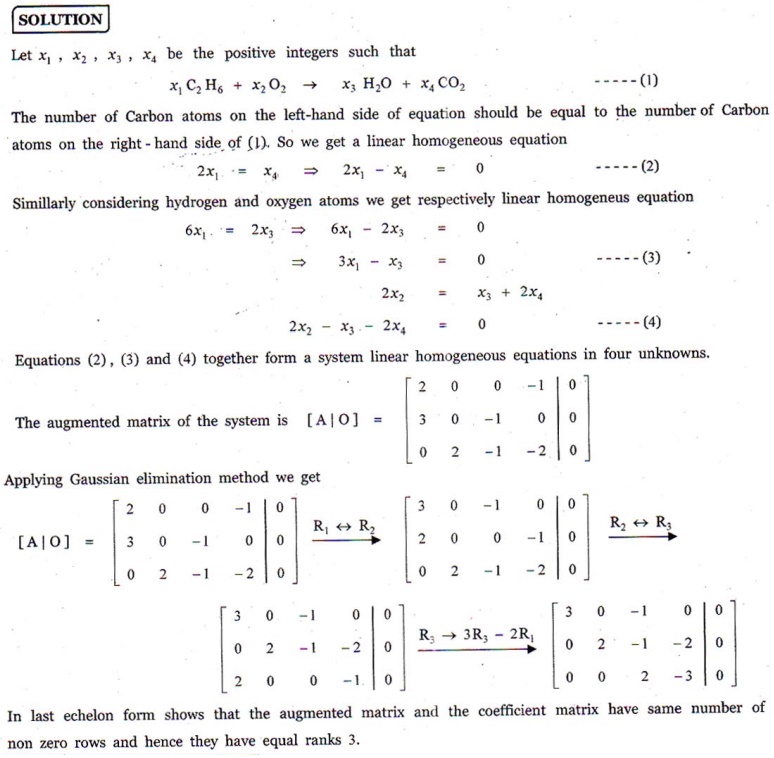
3. By using Gaussian elimination method, balance the chemical reaction equation:

C2H6 + O2 â†’ H2O + CO2

Answers for Exercise 1.7

1. (i) x = -t, y = -2t, z = t, t âˆˆ R

(ii) Trivial solutions only

2. (i) λ ≠ 8 (ii) λ = 8

3. 2C2 H6 + 7O2 â†’ 6H2O + 4CO2