Solving a Quadratic Equation by Completing the Square Method

Solving a Quadratic Equation by Completing the Square Method

In deriving the formula for the roots of a quadratic equation we used completing the squares method. The same technique can be applied in solving any given quadratic equation through the following steps

Step 1 Write the quadratic equation in general form ax² + bx +c = 0 .

Step 2 Divide both sides of the equation by the coefficient of x² if it is not 1.

Step 3 Shift the constant term to the right hand side.

Step 4 Add the square of one-half of the coefficient of x to both sides.

Step 5 Write the left hand side as a square and simplify the right hand side.

Step 6 Take the square root on both sides and solve for x.

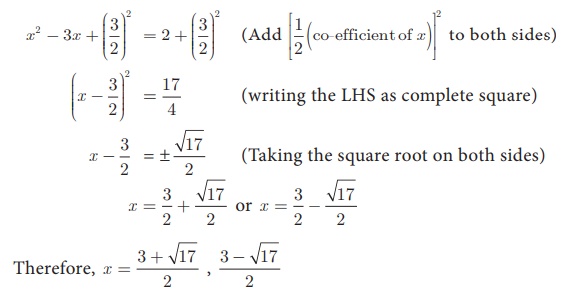
Example 3.31 

Solve x² − 3x − 2 = 0

Solution

x² − 3x − 2 = 0

x² - 3x = 2 (Shifting the Constant to RHS)

Example 3.32

Solve 2x ² − x −1 = 0

Solution 2x² − x −1 = 0