Chapter: 10th Mathematics : UNIT 3 : Algebra
Order of a Matrix
Order of a Matrix
If a matrix A has m number of rows and n
number of columns, then the order of the matrix A is (Number of rows)×(Number
of columns) that is, m×n .We read m×n as m
cross n or m by n. It may be noted that m×n is
not a product of m and n.
General form of a matrix A with m rows and n columns
(order m×n ) can be written in the form
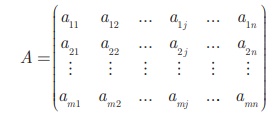
where, a11 , a12,... denote
entries of the matrix. a11 is the element in first
row, first column, a12 is the element in the
first row, second column, and so on.
In general, aij is the element in the ith
row and j th column and is referred as (i,j)th
element.
With this notation, we can express the matrix A as A
= (aij )m ×n where i
= 1, 2,....m and j = 1, 2,...n .
The total number of entries in the matrix A = (aij
)m ×n is mn.
Note
When giving the order of a matrix, you should always mention the
number of rows first, followed by the number of columns.
For example,
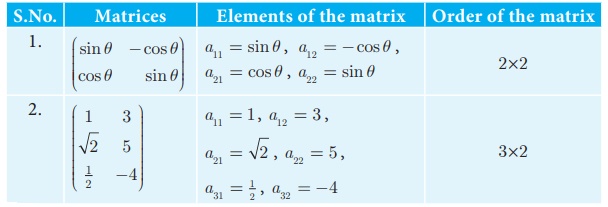
Related Topics