Remainder Theorem

Remainder Theorem

In the previous section , we have learnt the division of a polynomial by another non – zero polynomial. 

In this section , we shall study a simple and an elegant method of finding the remainder.

In the case of divisibility of a polynomial by a linear polynomial we use a well known theorem called Remainder Theorem.

If a polynomial p(x) of degree greater than or equal to one is divided by a linear polynomial (x–a) then the remainder is p(a), where a is any real number.

Significance of Remainder theorem : It enables us to find the remainder without actually following the cumbersome process of long division. 

It leads to another well known theorem called ‘Factor theorem’.

Example: 3.15

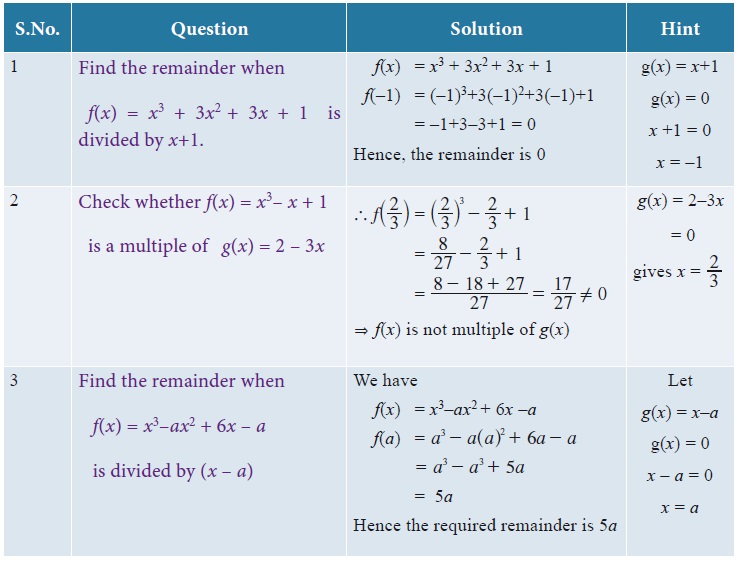
Example 3.16

If the polynomials f(x) = ax3 + 4x 2 + 3x –4 and g(x) = x3– 4x + a leave the same remainder when divided by x–3, find the value of a. Also find the remainder.

Solution

Let f(x) = ax3 + 4x 2 + 3x –4 and g(x) = x3– 4x + a, When f(x) is divided by (x–3), the remainder is f(3).

Now f(x) = a(3)3 + 4(3) 2 + 3(3) –4

 = 27a + 36 + 9 – 4

 f(3) = 27a + 41 (1)

When g(x) is divided by (x–3), the remainder is g(3).

Now g(3) = 33 – 4(3) + a

 = 27 – 12+ a

 = 15 + a (2)

Since the remainders are same, (1) = (2)

Given that, f(3) = g(3)

That is 27a + 41 = 15 + a

 27a – a = 15 – 41

 26a = –26

a = - 26/26 = –1

 Substituting a = –1,in f(3), we get

 f(3) = 27( ) - + 1 14

 = – 27 + 41

 f(3) = 14

so The remainder is 14.

Example 3.17 or 3.12

Without actual division , prove that f(x) = 2 x4 - 6 x3 + 3 x2 + 3x - 2 is exactly divisible by x2 –3x + 2

Solution :

Let f(x) = 2 x4 - 6 x3 + 3 x2 + 3x - 2

g(x) = x2 –3x + 2

= x2-2x-x+2

=x(x-2)-1(x-2)

=(x-2)(x-1)

we show that f(x) is exactly divisible by (x–1) and (x–2) using remainder theorem

f(1)= 2 (1)4 - 6 (1)3 + 3 (1)2 + 3(1) - 2

f(1)=2-6+3+3-2=0

f(2)= 2 (2)4 - 6 (2)3 + 3 (2)2 + 3(2) - 2

f(2)=32-48+12+6=0

f(x) is exactly divisible by (x – 1) (x – 2)

i.e., f(x) is exactly divisible by x2 –3x + 2

If p(x) is divided by (x -a) with the remainder p(a ) = 0 , then (x -a) is a factor of p(x). Remainder Theorem leads to Factor Theorem.

1. Factor Theorem

If p(x) is a polynomial of degree n ≥ 1 and ‘a’ is any real number then

(i) p(a ) = 0 implies (x -a) is a factor of p(x).

(ii) (x -a) is a factor of p(x) implies p(a ) = 0 .

Proof

If p(x) is the dividend and (x -a) is a divisor, then by division algorithm we write, p(x) = (x −a)q (x) + p(a) where q (x) is the quotient and p(a) is the remainder.

(i) If p(a) = 0, we get p(x) = (x −a)q (x) which shows that (x -a) is a factor of p(x).

(ii) Since (x -a) is a factor of p(x) , p(x) = (x – a)g (x) for some polynomial g (x) .

In this case

p(a ) = (a −a)g (a )

= 0 ×g (a )

= 0

Hence, p(a) = 0, when (x -a) is a factor of p(x).

Thinking Corner

For any two integers a(a ≠ 0) and b, a divides b if b = ax, for some integer x.

Note

• (x -a)  is a factor of p(x) , if p(a) = 0 ( x–a = 0, x = a)

• (x + a) is a factor of p(x) , if p(–a) = 0 ( x+a = 0, x = –a)

• (ax+b) is a factor of p(x) , if p(–b/a) = 0    ( ax + b = 0, ax = −b, x = − b/a) 

• (ax–b) is a factor of p(x) , if p(b/a) = 0  { (ax – b) = 0, ax = b, x =  b/a )

• (x–a) (x–b) is a factor of p(x) , if p(a)=0 and p(b) = 0 (  x − a = 0 or x − b = 0 , x = a or  x = b )

Example 3.13

Show that (x + 2) is a factor of x ³ − 4x² −2x + 20

Solution

Let p(x) = x ³ − 4x² − 2x + 20

To find the zero of x+2;

put x + 2 = 0

we get x = –2

By factor theorem, (x + 2) is factor of p(x), if p(− 2) = 0

 p(− 2) = (− 2)³ − 4(− 2)² −2(− 2) + 20

= −8−4(4)+4+20

p(− 2) = 0

Therefore, (x + 2) is a factor of x³ − 4x² −2x + 20

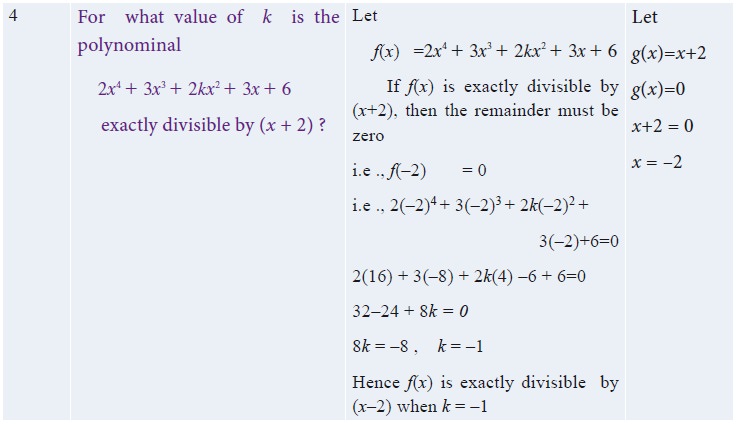
Example 3.14

Is (3x -2) a factor of 3x³ + x² −20x +12 ?

Solution

Let p(x) = 3x³ + x² −20x +12

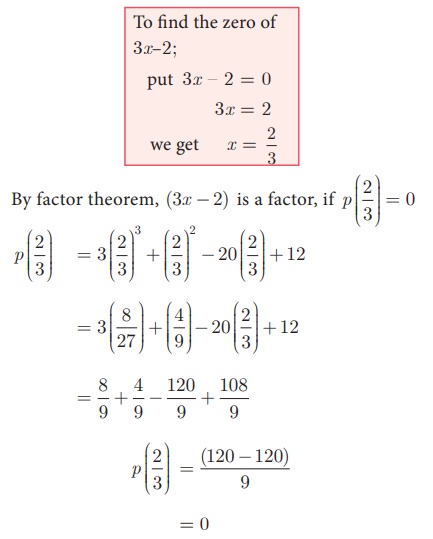
Therefore,(3x -2) is a factor of

3x ³ + x² −20x +12

Progress Check

1. (x+3) is a factor of p(x), if p(__) = 0

2. (3–x) is a factor of p(x), if p(__) = 0

3. (y–3) is a factor of p(y), if p(__) = 0

4. (–x–b) is a factor of p(x), if p(__) = 0

5. (–x+b) is a factor of p(x), if p(__) = 0

Example 3.15

Find the value of m, if (x -2) is a factor of the polynomial 2x³ − 6x² +mx + 4 .

Solution

Let p(x) = 2x³ −6x² + mx + 4

To find the zero of x–2;

put x – 2 = 0