Factorisation using Identity

Factorisation using Identity

(i) a ² + 2ab + b ² ≡ (a +b)²

(ii) a ² − 2ab +b² ≡ (a −b)²

(iii) a² − b² ≡ (a + b )(a −b)

(iv) a ² + b ² +c ² + 2ab + 2bc + 2ca ≡ (a + b +c)²

(v) a ³ + b ³ ≡ (a + b )(a ² −ab +b² )

(vi) a ³ − b ³ ≡ (a − b )(a ² +ab +b² )

(vii) a ³ + b ³ +c ³ − 3abc ≡ (a + b +c )(a ² + b ² +c² − ab −bc −ca)

Note

 (a + b )² +(a − b)² = 2(a² + b² ); a⁴ −b⁴ = (a² +b² )(a + b )(a −b)

 (a + b )² −(a −b)² = 4ab ; a⁶ − b⁶ = (a +b)(a − b )(a² −ab + b² )(a² +ab +b² )

Progress Check

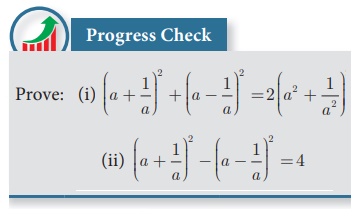
Example 3.25

Factorise the following:

(i) 9x² + 12xy + 4y² (ii) 25a² − 10a +1 (iii) 36m² − 49n² (iv) x³ –x (v) x⁴ − 16 (vi) x² + 4y² + 9z² − 4xy +12yz −6xz

Solution

(i) 9x² + 12xy + 4y² = (3x )² + 2(3x )(2y ) +(2y)² [ a ² + 2ab + b² = (a +b)² ]

 = (3x + 2y)²

(ii) 25a² − 10a +1 = (5a )² −2(5a )(1) +1²

= (5a −1)²    [ a ² − 2ab + b² = (a − b)² ]

(iii) 36m² − 49n². = (6m)² -(7n)²

= (6m + 7n)(6m −7n)               [a ² − b² = (a + b)(a −b)]

(iv) x³ − x = x (x² −1)

= x(x ² −1² )

= x (x +1)(x −1)

(v) x⁴ -16 = x⁴ -2⁴         [a⁴ − b⁴ =(a² + b²)(a + b)(a − b)]

 = (x ² + 2² )(x² −2² )

 = (x ² + 4)(x + 2)(x −2)

(vi) x ² + 4y ² + 9z ² − 4xy +12yz −6xz

 = (−x )² + (2y )² + (3z )² + 2(−x )(2y ) + 2(2y)(3z ) + 2(3z)(−x)

 = (−x + 2y + 3z)² or (x − 2y − 3z)²

Example 3.26

Factorise the following:

(i) 27x ³ +125y³

(ii) 216m ³ − 343n³

(iii) 2x⁴ -16xy³

(iv) 8x³ + 27y³ + 64z³ − 72xyz

Solution

(i) 27x³ +125y³

 = (3x)³ +(5y)²     [ (a³ + b³ ) = (a + b)(a² − ab + b² ) ]

= (3x + 5y)((3x)² − (3x)(5y ) + (5y)² )

= (3x + 5y)(9x² − 15xy + 25y² )

(ii) 216m ³ − 343n³ = (6m )³ −(7n)³         [a³− b³ ) = (a − b)(a ²+ ab + b² )]

= (6m − 7n) ((6m )² + (6m)(7n ) + (7n)² )

= (6m −7n)(36m ² + 42mn + 49n² )

(iii) 2x⁴ − 16xy³ = 2x (x ³ − 8y³ )

= 2x (x³ −(2y)³ )      [(a³ − b³ ) = (a − b)(a² + ab + b²)]

= 2x ((x − 2y )(x² + (x)(2y) + (2y)² ))

= 2x (x −2y )(x² + 2xy +4y² )

(iv) 8x³ + 27y³ + 64z³ −72xyz

= (2x)³ + (3y)³ + (4z)³ − 3(2x)(3y)(4z )

= (2x + 3y + 4z )(4x ² + 9y ² + 16z ² −6xy − 12yz − 8xz)

Thinking Corner

Check 15 divides the following

(i) 2017³ + 2018³

(ii) 2018³ – 1973³