Kinetic Theory of Gases | Physics - Solved Example Problems: Expression for pressure exerted by a gas | 11th Physics : UNIT 9 : Kinetic Theory of Gases
Chapter: 11th Physics : UNIT 9 : Kinetic Theory of Gases
Solved Example Problems: Expression for pressure exerted by a gas
EXAMPLE 9.1
A football at 27°C has 0.5 mole of air molecules. Calculate the internal energy of air in the ball.
Solution
The internal energy of ideal gas = 3/2 NkT. The number of air molecules is given in terms of number of moles so, rewrite the expression as follows
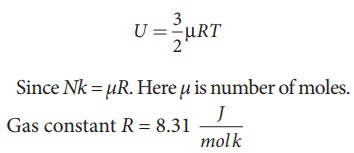
Temperature T =273+27=300K
U = 3/2 × 0.5 × 8.31 × 300 = 1869.75J
This is approximately equivalent to the kinetic energy of a man of 57 kg running with a speed of 8 m s-1.
EXAMPLE 9.2(Root mean square speed)
A room contains oxygen and hydrogen molecules in the ratio 3:1. The temperature of the room is 27°C. The molar mass of 02 is 32 g mol-1 and for H2 2 g mol-1. The value of gas constant R is 8.32 J mol-1K-1
Calculate
(a) rms speed of oxygen and hydrogen molecule
(b) Average kinetic energy per oxygen molecule and per hydrogen molecule
(c) Ratio of average kinetic energy of oxygen molecules and hydrogen molecules
Solution
(a) Absolute Temperature
T=27°C =27+273=300 K.
Gas constant R=8.32 J mol-1k-1
For Oxygen molecule: Molar mass
M=32 gm=32 x 10-3 kg mol-1

Note that the rms speed is inversely proportional to √M and the molar mass of oxygen is 16 times higher than molar mass of hydrogen. It implies that the rms speed of hydrogen is 4 times greater than rms speed of oxygen at the same temperature.
1934/484 ≈ 4 .
(b) The average kinetic energy per molecule is 3/2 kT. It depends only on absolute temperature of the gas and is independent of the nature of molecules. Since both the gas molecules are at the same temperature, they have the same average kinetic energy per molecule. k is Boltzmaan constant.

(c) Average kinetic energy of total oxygen molecules = 3/2 N0kT where N0 - number of oxygen molecules in the room
Average kinetic energy of total hydrogen molecules = 3/2 NHkT where NH - number of hydrogen molecules in the room.
It is given that the number of oxygen molecules is 3 times more than number of hydrogen molecules in the room. So the ratio of average kinetic energy of oxygen molecules with average kinetic energy of hydrogen molecules is 3:1
EXAMPLE 9.3
Ten particles are moving at the speed of 2, 3, 4, 5, 5, 5, 6, 6, 7 and 9 m s-1. Calculate rms speed, average speed and most probable speed.
Solution
The average speed
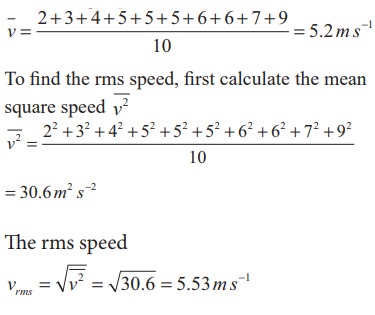
The most probable speed is 5 ms-1 because three of the particles have that speed.
EXAMPLE 9.4
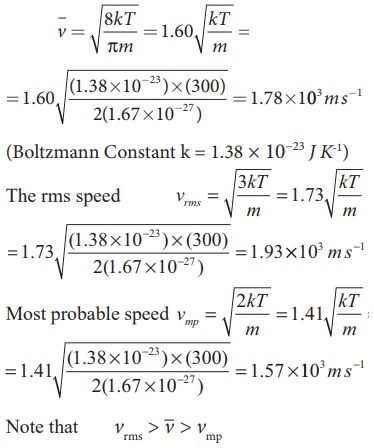
Calculate the rms speed, average speed and the most probable speed of 1 mole of hydrogen molecules at 300 K. Neglect the mass of electron.
Solution
The hydrogen atom has one proton and one electron. The mass of electron is negligible compared to the mass of proton.
Mass of one proton = 1.67 × 10−27kg.
One hydrogen molecule = 2 hydrogen atoms = 2 × 1.67 × 10−27kg.
The average speed
Related Topics