Chapter: Modern Analytical Chemistry: Spectroscopic Methods of Analysis
Characterization Applications - Ultraviolet-Visible and Infrared Spectrophotometry
Characterization Applications
Molecular absorption, particularly in the UV/Vis
range, has been used for a variety of different characterization studies, including determining the
stoichiometry of metal–ligand complexes and determining equilibrium constants. Both of these
ex- amples are examined
in this section.
Stoichiometry of a Metal–Ligand Complex
The stoichiometry for a metal–ligand complexation reaction of the following general form
M+ yL < == > MLy
can be determined by one of three methods: the method of
continuous variations, the mole-ratio method, and the slope-ratio method.
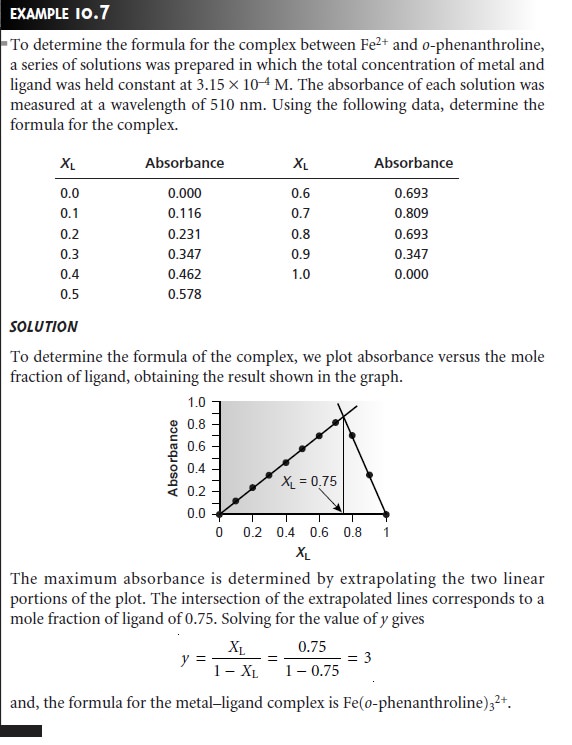
Of the three methods, the method of continuous variations, also called Job’s
method, is the most popular.
In this method
a series of solutions is prepared such that the total moles
of metal and
ligand, ntot, in each
solution is the
same. Thus, if (nM)i and (nL)i are, respectively, the moles of metal and ligand in the i-th solution, then
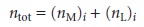
The relative amount
of ligand and metal in each solution
is expressed as the mole fraction of ligand, (XL)i, and the mole
fraction of metal,
(XM)i,
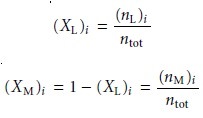
The
concentration of the metal–ligand complex is determined by the limiting
reagent, with the greatest concentration occurring when the metal and ligand are mixed stoichiometrically. If the
reaction is monitored at a wavelength where only the metal–ligand complex absorbs, a plot of absorbance versus the mole fraction of ligand
will show two
linear branches: one
when the ligand
is the limiting reagent and a second
when the metal
is the limiting reagent. The intersection of these two branches occurs when a stoichiometric mixing
of metal and ligand is reached. The mole
fraction of ligand at this intersection is used to determine the value of y for the metal–ligand complex, MLy.
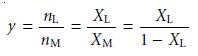
If there is no wavelength where only the metal–ligand complex
absorbs, then the measured absorbances must be corrected for the absorbance that would be exhib-
ited if the metal and
ligand did not
react to form
MLy.
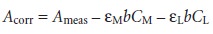
In essence, the corrected absorbance gives the change
in absorbance due to the for-
mation of the metal–ligand complex. An example of the application of the method of continuous variations is shown in Example 10.7.
Several precautions are necessary when using the method of continuous varia- tions. First, the method
of continuous variations requires that a single metal–ligand complex be formed. To determine if this condition is true, plots
of absorbance ver- sus
XL should
be constructed for
several different wavelengths and for several
differ- ent values of ntot. If the maximum
absorbance does not
occur at the
same value of XL for each set of conditions, then more than one metal–ligand complex must be present. A second precaution is that the metal–ligand complex
must obey Beer’s
law for the range of concentrations used in constructing the plot of absorbance versus XL. Third,
if the metal–ligand complex’s formation constant is relatively small, the plot of absorbance versus
XL may show significant curvature. In this case it is often difficult to determine the stoichiometry by extrapolation. Finally,
since the stability of the metal–ligand complex
may be influenced by solution
conditions, the compo- sition of the solutions must be carefully controlled. When the ligand is a weak base,
for example, the solutions must be buffered
to the same pH.
In the mole-ratio method
the moles of one reactant, usually the metal,
are held constant, while the moles of the other reactant
are varied. The absorbance is moni-
tored at a wavelength at which the metal–ligand complex
absorbs. A plot of ab- sorbance as a function of the ligand-to-metal mole ratio (nL/nM) has two linear branches that
intersect at a mole ratio
corresponding to the
formula of the
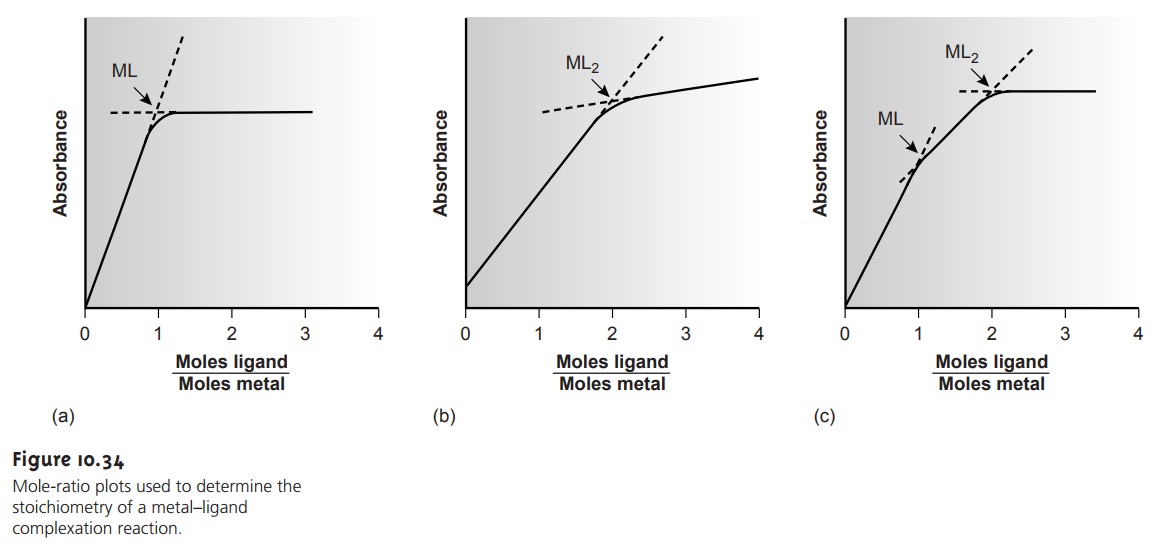
complex. Figure 10.34a shows
a mole-ratio plot for the formation of a 1:1 complex in which
the absorbance is monitored at a wavelength at which only the complex
absorbs. Figure 10.34b shows
a mole-ratio plot
for a 1:2 complex in which the
metal, the ligand, and the complex
absorb at the selected wavelength. Unlike the method
of continuous variations, the mole-ratio method can be used for
complexation reac- tions that occur in a stepwise
fashion, provided that the molar
absorptivities of the metal–ligand complexes differ and the
formation constants are sufficiently differ- ent. A typical mole-ratio plot for the
stepwise formation of ML and
ML2 is shown
in Figure 10.34c.

Both the method
of continuous variations and the mole-ratio method rely on an
extrapolation of absorbance data collected under
conditions in which
a linear re- lationship exists between absorbance and the relative amounts of metal
and ligand. When a metal–ligand complex
is very weak,
a plot of absorbance versus
XL or nL/nM may be curved,
making it impossible to determine the stoichiometry by extrapola-
tion. In this case the slope ratio
may be used.
In the slope-ratio method
two sets of solutions are prepared. The first set con-
sists of a constant amount
of metal and a variable
amount of ligand,
chosen such that the total concentration of metal, CM, is much greater
than the total
concentra- tion of ligand,
CL. Under
these conditions we may assume
that essentially all the
ligand is complexed. The concentration of a metal–ligand complex of the
general form MxLy is
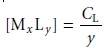
If absorbance is monitored at a wavelength where only MxLy absorbs, then
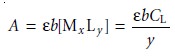
and a plot of absorbance versus CL will be linear with a slope, sL, of
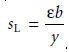
A second set
of solutions is prepared with
a fixed concentration of ligand that
is much greater than
the variable concentration of metal; thus
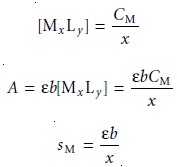
The mole ratio of ligand-to-metal is determined from the ratio
of the two slopes.
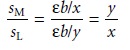
An important assumption in the slope-ratio method is that the complexation reac- tion continues to completion in the presence of a sufficiently large excess of metal
or ligand. The slope-ratio method
also is limited
to systems that
obey Beer’s law
and in which only a single
complex is formed.
Determination of Equilibrium Constants
Another important application of molec- ular absorption is the determination of equilibrium constants. Let’s consider, as a
simple example, an acid–base reaction of the general
form
HIn+
H2O < = = > H3O+ + In–
where HIn and In– are the conjugate weak acid and weak base forms of a visual acid–base indicator. The equilibrium constant for this
reaction is
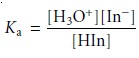
To determine the equilibrium constant’s value, we prepare
a solution in which the reaction exists in a state of equilibrium and determine the equilibrium concentration of H3O+, HIn, and In–. The concentration of H3O+ is easily determined by measuring
the solution’s pH, whereas the concentration of HIn and In– may be determined by measuring the solution’s absorbance.
If both HIn and In– absorb at the selected
wavelength, then, from equation
10.6, we know that
A = εHInb[HIn] + εInb[In–] ……….10.20
where εHIn and εIn are the molar
absorptivities for HIn and In–. The total
concentra- tion of indicator, C, is given by a mass balance equation
C = [HIn] + [In–] ……….10.21
Solving equation 10.21 for [HIn] and substituting into equation
10.20 gives
A = εHInb(C – [In–]) + εInb[In–]
which simplifies to
A = εHInbC – εHInb[In–]+
εInb[In–]
A =
AHIn + b[In–](εIn – εHIn)
……….10.22
where
AHIn, which is equal to εHInbC, is the
absorbance when the
pH is acidic enough that essentially all the indicator is present as HIn. Solving
equation 10.22 for the
concentration of In– gives
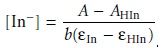 10.23
10.23
Proceeding in the same fashion,
we can derive a similar
equation for the concentra-
tion of HIn; thus
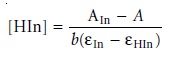 10.24
10.24
where AIn, which is equal
to εInbC, is the absorbance when the pH is basic
enough that only In– contributes to the absorbance. Substituting equations 10.23
and 10.24 into the equilibrium constant
expression for HIn gives
 10.25
10.25
Using equation 10.25,
the value of Ka can be determined in one of two ways.
The simplest approach is to prepare
three solutions, each of which
contains the same amount, C, of indicator. The pH of one solution
is made acidic
enough that [HIn] >> [In–].
The absorbance of this solution
gives AHIn. The value
of AIn is determined by adjusting the pH of the second solution
such that [In–] >> [HIn].
Finally, the pH of
the third solution
is adjusted to an intermediate value, and the pH and ab- sorbance, A, are
recorded. The value
of Ka can then be calculated by making appro- priate substitutions into equation
10.25.
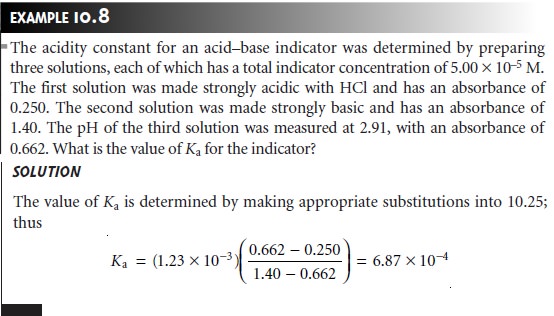
A second approach is to prepare
a series of solutions, each
of which contains the same amount of indicator. Two solutions are used to determine values
for AHIn and AIn. Rewriting equation 10.25
in logarithmic form
and rearranging
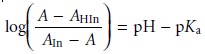
shows that a plot of log [(A –
AHIn)/(AIn – A)]
versus pH is linear, with
a slope of +1
and a y-intercept of –pKa.
In developing this treatment for determining equilibrium
constants, we have considered a relatively simple system in which the absorbance of HIn and In– were easily measured, and for which it is easy to determine
the concentration of H3O+. In addition to acid–base reactions, the same approach
can be applied to any reaction
of the general form
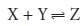
including metal–ligand complexation and redox reactions,
provided that the con- centration of the product, Z, and one of the reactants can be determined spec- trophotometrically and the concentration of the other
reactant can be determined
by another method. With appropriate modifications, more-complicated systems,
in which one or more of these parameters cannot be measured, also can be treated.
Related Topics