Absorbance of Electromagnetic Radiation - Spectroscopy Based on Absorption

Absorbance of Electromagnetic Radiation

In absorption spectroscopy a beam of electromagnetic radiation passes through a sam- ple. Much of the radiation is transmitted without a loss in intensity. At selected fre- quencies, however, the radiation’s intensity is attenuated. This process of attenuation is called absorption. Two general requirements must be met if an analyte is to absorb electromagnetic radiation. The first requirement is that there must be a mechanism by which the radiation’s electric field or magnetic field interacts with the analyte. For ul- traviolet and visible radiation, this interaction involves the electronic energy of valence electrons. A chemical bond’s vibrational energy is altered by the absorbance of infrared radiation. A more detailed treatment of this interaction, and its importance in deter- mining the intensity of absorption.

The second requirement is that the energy of the electromagnetic radia- tion must exactly equal the difference in energy, ∆E, between two of the ana- lytes quantized energy states. Figure 10.4 shows a simplified view of the ab- sorption of a photon. The figure is useful because it emphasizes that the photon’s energy must match the difference in energy between a lower-energy state and a higher-energy state. What is missing, however, is information about the types of energetic states involved, which transitions between states are likely to occur, and the appearance of the resulting spectrum.

We can use the energy level diagram in Figure 10.14 to explain an ab- sorbance spectrum. The thick lines labeled E₀ and E₁ represent the analyte’s ground (lowest) electronic state and its first electronic excited state. Superim- posed on each electronic energy level is a series of lines representing vibra- tional energy levels.

Infrared Spectra for Molecules and Polyatomic Ions 

The energy of infrared radiation is sufficient to produce a change in the vibrational energy of a mole- cule or polyatomic ion (see Table 10.1). As shown in Figure 10.14, vibrational energy levels are quantized; that is, a molecule may have only certain, discrete vibrational energies. The energy for allowed vibrational modes, E_v, is

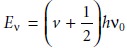
where v is the vibrational quantum number, which may take values of 0, 1, 2,

. . ., and v₀ is the bond’s fundamental vibrational frequency. Values for v₀ are determined by the bond’s strength and the mass at each end of the bond and are characteristic of the type of bond. For example, a carbon–carbon sin- gle bond (C—C) absorbs infrared radiation at a lower energy than a carbon–carbon double bond (C=C) because aC—C bond is weaker than a C=C bond.

At room temperature most molecules are in their ground vibrational state (v = 0). A transi- tion from the ground vibrational state to the first vibrational excited state (v = 1) requires the absorption of a photon with an energy of hv₀. Transitions in which ∆v is 1 give rise to the fundamental absorption lines. Weaker absorp- tion lines, called overtones, are due to transi- tions in which ∆v is ±2 or ±3. The number of possible normal vibrational modes for a linear molecule is 3N – 5, and for a nonlinear mole- cule is 3N – 6, where N is the number of atoms in the molecule. Not surprisingly, infrared spec- tra often show a considerable number of ab- sorption bands. 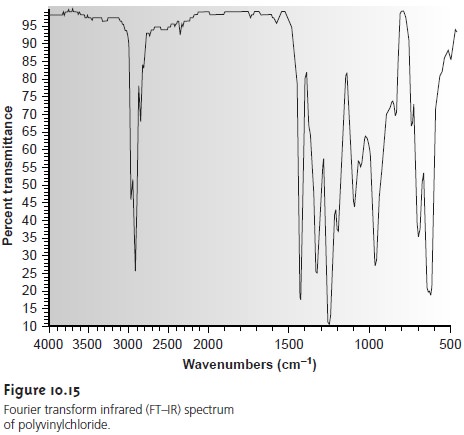Even a relatively simple mole- cule, such as benzene (C₆H₆), for example, has 30 possible normal modes of vibration, al- though not all of these vibrational modes give rise to an absorption. A typical IR spectrum is shown in Figure 10.15.

UV/Vis Spectra for Molecules and Ions 

When a molecule or ion absorbs ultravio- let or visible radiation it undergoes a change in its valence electron configuration. The valence electrons in organic molecules, and inorganic anions such as CO₃2–, oc- cupy quantized sigma bonding, σ, pi bonding, Ï€, and nonbonding, n, molecular or- bitals. Unoccupied sigma antibonding, σ*, and pi antibonding, Ï€*, molecular or- bitals often lie close enough in energy that the transition of an electron from an occupied to an unoccupied orbital is possible.

Four types of transitions between quantized energy levels account for molecu- lar UV/Vis spectra. The approximate wavelength ranges for these absorptions, as well as a partial list of bonds, functional groups, or molecules that give rise to these transitions is shown in Table 10.5. Of these transitions, the most important are the n → π* and s → π *, because they involve functional groups that are characteristic of the analyte and wavelengths that are easily accessible. The bonds and functional groups that give rise to the absorption of ultraviolet and visible radiation are called chromophores.

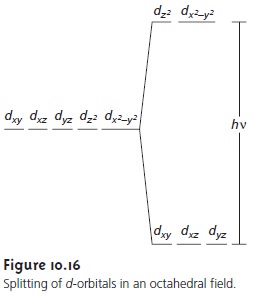
Many transition metal ions, such as Cu2+ and Co2+, form solutions that are colored because the metal ion absorbs visible light. The transitions giving rise to this absorption are due to valence electrons in the metal ion’s d-orbitals. For a free metal ion, the five d-orbitals are of equal energy. In the presence of a com- plexing ligand or solvent molecule, however, the d-orbitals split into two or more groups that differ in energy. For example, in the octahedral complex Cu(H₂O)₆2+ the six water molecules perturb the d-orbitals into two groups as shown in Figure 10.16. The resulting d–d transitions for transition metal ions are relatively weak.

A more important source of UV/Vis absorption for inorganic metal–ligand complexes is charge transfer, in which absorbing a photon produces an excited state species that can be described in terms of the transfer of an electron from the metal, M, to the ligand, L.

M—L + hv → M+—L–

Charge-transfer absorption is important because it produces very large absorbances, providing for a much more sensitive analytical method. One important example of a charge-transfer complex is that of o-phenanthroline with Fe2+, the UV/Vis spec- trum for which is shown in Figure 10.17. Charge-transfer absorption in which the electron moves from the ligand to the metal also is possible.

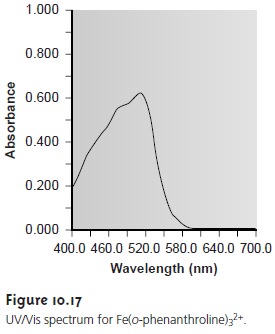
Comparing the IR spectrum in Figure 10.15 to the UV/Vis spectrum in Figure 10.17, we note that UV/Vis absorption bands are often significantly broader than those for IR absorption. Figure 10.14 shows why this is true. 

When a species absorbs UV/Vis radiation, the transition between electronic energy levels may also include a transition between vibrational energy levels. The result is a num- ber of closely spaced absorption bands that merge together to form a single broad absorption band.

UV/Vis Spectra for Atoms 

As noted in Table 10.1, the energy of ultra- violet and visible electromagnetic radiation is sufficient to cause a change in an atom’s valence electron configuration. Sodium, for exam- ple, with a valence shell electron configuration of [Ne] 3s1, has a single valence electron in its 3s atomic orbital. Unoccupied, higher energy atomic orbitals also exist. Figure 10.18 shows a partial energy level dia- gram for sodium’s occupied and unoccupied valence shell atomic or- bitals. This configuration of atomic orbitals, which shows a splitting of the p orbitals into two levels with slightly different energies, may differ from that encountered in earlier courses. The reasons for this splitting, however, are beyond the level of this text, and unimportant in this context.

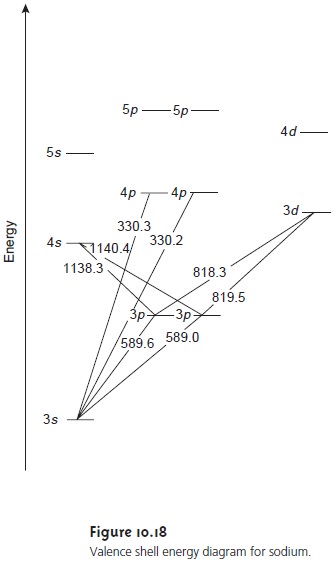
Absorption of a photon is accompanied by the excitation of an electron from a lower-energy atomic orbital to an orbital of higher energy. Not all possible transitions between atomic orbitals are al- lowed. For sodium the only allowed transitions are those in which there is a change of ±1 in the orbital quantum number (l); thus transitions from s→p orbitals are allowed, but transitions from s→d orbitals are forbidden. The wavelengths of electromagnetic ra- diation that must be absorbed to cause several allowed transitions are shown in Figure 10.18.

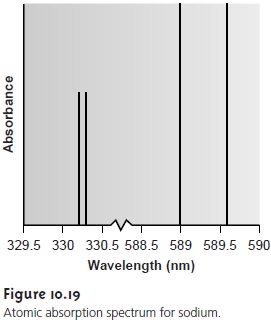
The atomic absorption spectrum for Na is shown in Figure 10.19 and is typical of that found for most atoms. The most obvi- ous feature of this spectrum is that it consists of a few, discrete ab- sorption lines corresponding to transitions between the ground state (the 3s atomic orbital) and the 3p and 4p atomic orbitals. Ab- sorption from excited states, such as that from the 3p atomic or bital to the 4s or 3d atomic orbital, which are included in the en- ergy level diagram in Figure 10.18, are too weak to detect. Since the lifetime of an excited state is short, typically 10–7–10–8 s, an atom in the ex- cited state is likely to return to the ground state before it has an opportu- nity to absorb a photon.

Another feature of the spectrum shown in Figure 10.19 is the narrow width of the absorption lines, which is a consequence of the fixed difference in energy between the ground and excited states. Natural line widths for atomic absorption, which are governed by the uncertainty principle, are ap- proximately 10–5 nm. Other contributions to broadening increase this line width to approximately 10–3 nm.