Chapter: Modern Analytical Chemistry: Equilibrium Chemistry
Systematic Approach to Solving Equilibrium Problems
Systematic
Approach to Solving Equilibrium Problems
Calculating the solubility
of Pb(IO3)2
in a solution of Pb(NO3)2 was more com- plicated
than calculating its solubility in
distilled water. The necessary
calcula- tions, however,
were still relatively easy to organize,
and the assumption used to simplify
the problem was fairly obvious. This problem was reasonably
straight- forward because it involved only a single
equilibrium reaction, the
solubility of
Pb(IO3)2. Calculating the equilibrium composition of a system with multiple equilibrium reactions can become quite complicated. In this section we will learn how to use a systematic
approach to setting
up and solving equilibrium
problems.
As its name implies, a systematic approach involves a series of
steps:
·
Write all relevant equilibrium reactions and their
equilibrium constant
expressions.
·
Count the number of species whose
concentrations appear in the equilibrium constant expressions; these are your unknowns. If the number
of unknowns equals the number of equilibrium constant
expressions, then you have enough information to solve the
problem. If not,
additional equations based
on the conservation of mass and
charge must be written. Continue to add equations until you have the same number
of equations as you have unknowns.
·
Decide how accurate your
final answer needs
to be. This
decision will influence your evaluation of any assumptions you use to simplify the problem.
·
Combine your equations to solve for one unknown
(usually the one you are most
interested in knowing). Whenever possible, simplify the algebra by making appropriate assumptions.
·
When you obtain your final answer,
be sure to check your assumptions. If any
of your assumptions prove invalid,
then return to the previous
step and continue solving.
The problem is complete when you have an answer
that does not violate
any of your assumptions.
Besides equilibrium constant
equations, two other
types of equations are used in the systematic approach
to solving equilibrium problems. The first
of these is a
mass
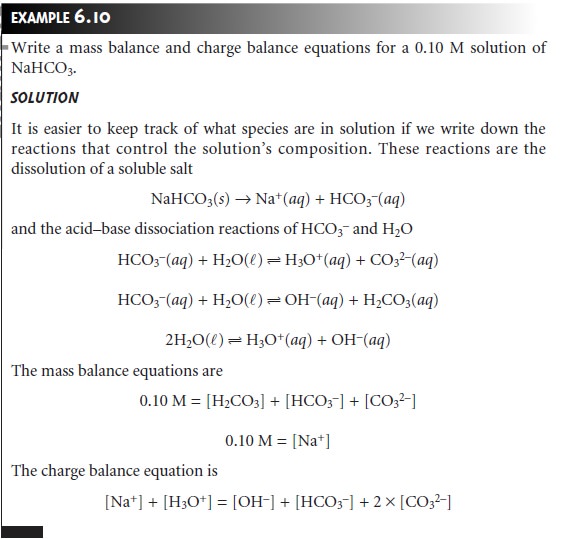
balance equation, which is simply a statement of the conservation of matter. In a solution of a monoprotic weak acid, for example, the combined concentrations of the conjugate weak acid, HA, and the conjugate weak base, A–, must equal
the weak acid’s initial
concentration, CHA.*
The second type of equation
is a charge balance
equation. A charge balance equation is a statement of solution electroneutrality.
Total positive charge
from cations = total negative
charge from anions Mathematically, the
charge balance expression is expressed as
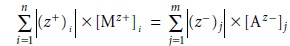
where
[Mz+]i and [Az–]j are,
respectively, the
concentrations of the ith cation
and the jth anion, and (z+)i and
(z–)j are the charges
of the ith cation and the jth anion. Note that the concentration terms are multiplied
by the absolute values of each ion’s charge, since electroneutrality is a conservation of charge,
not con- centration. Every ion in solution, even those not involved
in any equilibrium reactions,
must be included in the charge balance equation. The charge balance equation
for an aqueous solution of Ca(NO3)2 is
|
3 |
Note that the concentration of Ca2+ is
multiplied by 2, and that the concentrations of H3O+ and OH– are also included. Charge
balance equations must be written carefully since every ion
in solution must
be included. This
presents a problem when the concentration of one ion in solution
is held constant
by a reagent of un- specified composition. For example,
in many situations pH is held constant
using a buffer. If the composition of the buffer
is not specified, then a charge balance
equa- tion cannot be written.
Related Topics