Chapter: Modern Analytical Chemistry: Equilibrium Chemistry
Equilibrium Constants for OxidationŌĆōReduction Reactions
OxidationŌĆōReduction Reactions
In a complexation reaction, a Lewis base donates
a pair of electrons to a Lewis acid.
In an oxidationŌĆōreduction reaction, also
known as a redox reaction, electrons are not shared, but are transferred from one reactant
to another. As a result
of this elec- tron transfer, some of the elements
involved in the reaction undergo
a change in ox-
idation state. Those
species experiencing an increase in their oxidation state are oxi- dized, while those experiencing a decrease in their oxidation state are reduced.
For example, in the following redox
reaction between Fe3+ and oxalic
acid, H2C2O4,
iron is reduced since its
oxidation state changes
from +3 to +2.
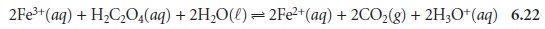
Oxalic acid, on the other
hand, is oxidized since the oxidation state for carbon
in- creases from +3 in H2C2O4 to +4 in CO2.
Redox reactions, such
as that shown
in equation 6.22,
can be divided
into sepa- rate half-reactions that individually describe the oxidation
and the reduction processes.
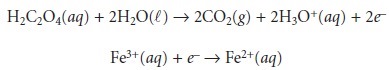
It is important to remember, however,
that oxidation and reduction reactions al- ways occur in pairs.* This relationship is formalized by the convention of calling the species being oxidized a reducing
agent, because it provides the electrons for the re- duction half-reaction. Conversely, the species being reduced is called an oxidizing agent. Thus, in reaction
6.22, Fe3+ is
the oxidizing agent
and H2C2O4 is the reducing agent.
The products of a redox
reaction also have redox properties. For example, the Fe2+ in reaction
6.22 can be oxidized to Fe3+, while
CO2 can be reduced to H2C2O4.
Borrowing some terminology from acidŌĆōbase
chemistry, we call Fe2+ the conjugate reducing agent of the oxidizing agent Fe3+ and CO2 the conjugate oxidizing agent of the
reducing agent H2C2O4.
Unlike the reactions that we have already considered, the equilibrium position of a redox reaction
is rarely expressed by an equilibrium constant. Since redox
reac- tions involve the
transfer of electrons from a reducing agent to an oxidizing agent, it is convenient to consider the thermodynamics of the reaction
in terms of the
electron.
The free energy, ŌłåG,
associated with moving a charge, Q, under
a potential, E, is given by
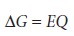
Charge is proportional to the number
of electrons that must be moved. For a reac-
tion in which
one mole of reactant is oxidized or reduced, the charge, in coulombs, is
Q = nF
where n is the number of moles of electrons per mole of reactant, and F is FaradayŌĆÖs constant (96,485 C . molŌĆō1). The
change in free
energy (in joules
per mole; J/mol) for a redox reaction, therefore, is
ŌłåG = ŌĆōnFE
6.23
where ŌłåG has units of
joules per mole. The appearance of a minus sign in equation 6.23 is due to a difference in the conventions for assigning the favored direction
for reactions. In thermodynamics, reactions are favored
when ŌłåG is
negative, and redox reactions are favored when
E
is positive.
The relationship between
electrochemical potential and the concentrations of reactants and products can be determined by substituting equation 6.23 into equation 6.3
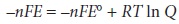
where E┬░ is the electrochemical potential under standard-state conditions. Dividing through by ŌĆōnF leads to the well-known Nernst equation.
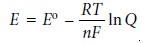
Substituting appropriate values for R and
F, assuming a temperature of 25 ┬░C (298 K),
and switching from
ln to log* gives the
potential in volts
as
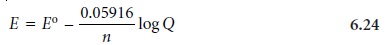
The standard-state electrochemical potential, E┬░, provides an alternative way of
expressing the equilibrium constant for a redox reaction. Since a reaction at equilib- rium has a ŌłåG of
zero, the electrochemical potential, E, also must be zero.
Substi- tuting into equation
6.24 and rearranging shows that

Standard-state potentials are
generally not tabulated for chemical reactions, but are calculated using
the standard-state potentials for the oxidation, E┬░ox, and reduction
half-reactions, E┬░red. By convention, standard-state potentials are only listed
for re- duction half-reactions, and E┬░ for a reaction is calculated as
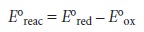
where both E┬░red
and E┬░ox are
standard-state reduction potentials.
Since the potential for a single
half-reaction cannot be measured, a reference half- reaction is arbitrarily assigned a standard-state potential of zero. All
other reduction potentials are
reported relative to this reference. The standard half-reaction is
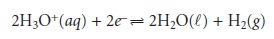
Appendix 3D contains
a listing of the standard-state reduction potentials for se-
lected species. The more positive
the standard-state reduction potential, the more favorable the reduction reaction
will be under
standard-state conditions. Thus, under standard-state conditions, the reduction of Cu2+ to Cu (E┬░ = +0.3419)
is more favorable than
the reduction of Zn2+ to Zn (E┬░ = ŌĆō0.7618).
Related Topics