Chapter: Modern Analytical Chemistry: Equilibrium Chemistry
Solving Equilibrium Problems: pH of a Monoprotic Weak Acid
pH of a Monoprotic Weak Acid
To illustrate the systematic approach,
let us calculate the pH of 1.0 M HF. Two
equilbria affect the pH of this system.
The first, and most obvious,
is the acid disso- ciation reaction
for HF
HF(aq)+ H2O(l) < = = > H3O+(aq)+ F–(aq)
for which the equilibrium constant expression is

The second equilibrium reaction is the
dissociation of water,
which is an obvious
yet easily disregarded reaction
2H2O(l)
<
== == > H3O+(aq)+ OH–(aq)
Kw = [H3O+][OH–] = 1.00 x 10–14 6.36
Counting unknowns, we find four
([HF], [F–], [H3O+], and [OH–]). To solve this problem, therefore, we need
to write two
additional equations involving these un- knowns. These
equations are a mass balance
equation
CHF =
[HF]+ [F–] 6.37
and a charge
balance equation
H3O+]= [F–] + [OH–] 6.38
We now have
four equations (6.35,
6.36, 6.37, and
6.38) and four
unknowns ([HF], [F–], [H3O+], and [OH–]) and are ready
to solve the problem. Before
doing so, however, we will simplify the algebra by making two reasonable assumptions. First, since
HF is a weak acid,
we expect the solution to be acidic;
thus it is reason-
able to assume that
[H3O+] >> [OH–]
simplifying the charge balance equation (6.38) to
[H3O+]=
[F–] 6.39
Second, since HF is a weak acid we expect that very little
dissociation occurs, and
[HF] >> [F–]
Thus, the mass balance equation (6.36) simplifies to
CHF = [HF] 6.40
For this exercise
we will accept our assumptions if the error introduced by each as- sumption is less than ±5%.
Substituting equations 6.39 and 6.40 into the equilibrium constant
expression for the dissociation of HF (equation 6.35) and solving
for the concentration of H3O+ gives us

Before accepting this answer, we must verify that our assumptions are acceptable.
The first assumption was that
the [OH–] is significantly smaller
than the [H3O+]. To calculate the concentration of OH– we use the Kw expression (6.36)
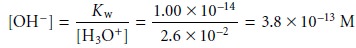
Clearly this assumption is reasonable. The second assumption was that the [F–] is significantly smaller than the
[HF]. From equation 6.39 we have
[F–] = 2.6 x 10–2 M
Since the [F–] is 2.6% of CHF, this assumption is also within
our limit that the error be
no more than ±5%. Accepting
our solution for the concentration of H3O+, we find
that the pH of 1.0 M HF is 1.59.
How does the result of this calculation change if we require our assumptions to have
an error of less than
±1%. In this
case we can
no longer assume
that [HF] >> [F–].
Solving the mass
balance equation (6.37)
for [HF]
[HF] = CHF
– [F–]
and substituting into the Ka expression along with equation 6.39 gives
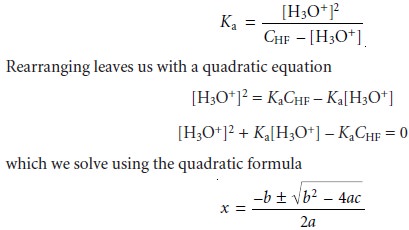
where a, b, and
c
are the coefficients in the quadratic equation ax2 + bx +
c
= 0. Solving the quadratic formula
gives two roots, only one of which has any chemical
significance. For our problem the quadratic formula
gives roots of
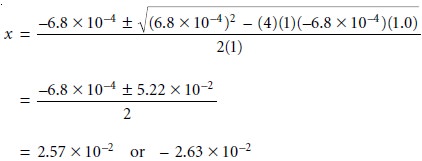
Only the positive
root has any chemical significance since the negative
root implies that the concentration of H3O+ is
negative. Thus, the [H3O+] is 2.6 x 10–2 M,
and the pH to two significant figures is still
1.59.
This same approach
can be extended to find the pH of a monoprotic weak base, replacing Ka with Kb, CHF with the weak base’s concentration, and solving for the
[OH–] in place of [H3O+]
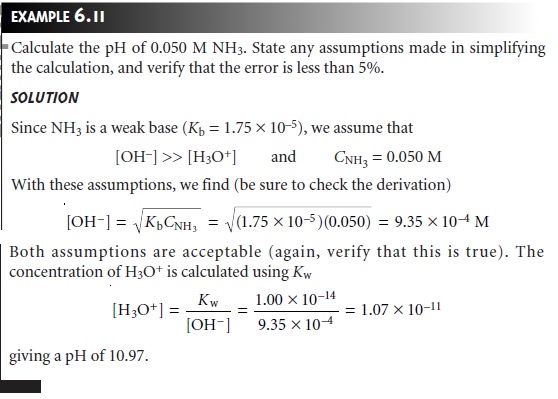
Related Topics