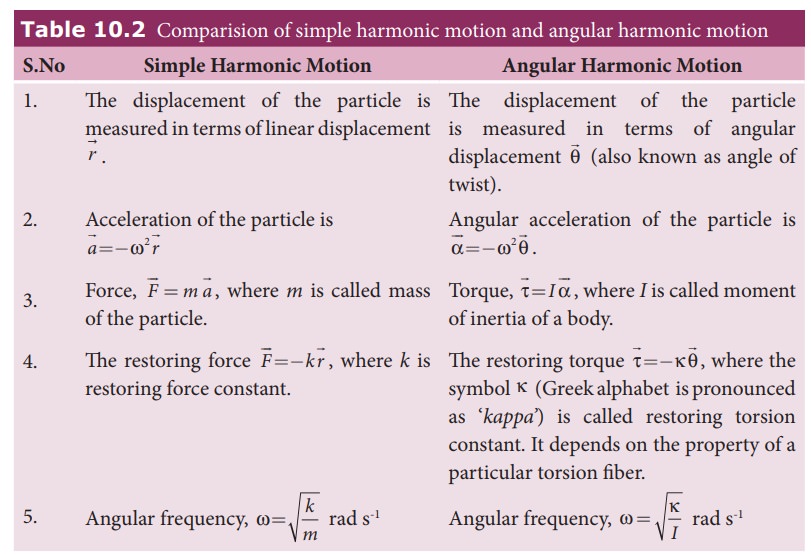
Comparison of Simple Harmonic Motion and Angular Simple Harmonic Motion

Comparison of Simple Harmonic Motion and Angular Simple Harmonic Motion

In linear simple harmonic motion, the displacement of the particle is measured in terms of linear displacement  The restoring force is  =− k  , where k is a spring constant or force constant which is force per unit displacement. In this case, the inertia factor is mass of the body executing simple harmonic motion.

In angular simple harmonic motion, the displacement of the particle is measured in terms of angular displacement . Here, the spring factor stands for torque constant i.e., the moment of the couple to produce unit angular displacement or the restoring torque per unit angular displacement. In this case, the inertia factor stands for moment of inertia of the body executing angular simple harmonic oscillation.