Chapter: 12th Mathematics : UNIT 2 : Complex Numbers
Solved Example Problems on de MoivreŌĆÖs Theorem
Example 2.28
If z = (cos╬Ė + i sin╬Ė ) , show that zn + 1/ zn = 2 cos n╬Ė and zn ŌĆō [1/ zn] = 2i sin n╬Ė .
Solution
Let z = (cos╬Ė + i sin╬Ė ) .
By de MoivreŌĆÖs theorem ,
zn = (cos╬Ė + i sin╬Ė )n = cos n╬Ė + i sin n╬Ė
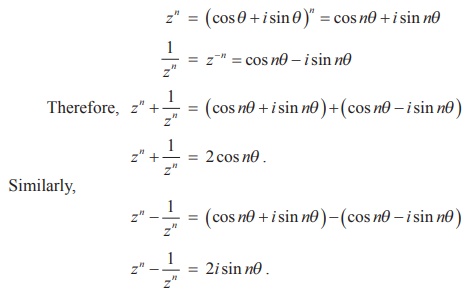
Example 2.29
Similarly, 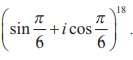
Solution
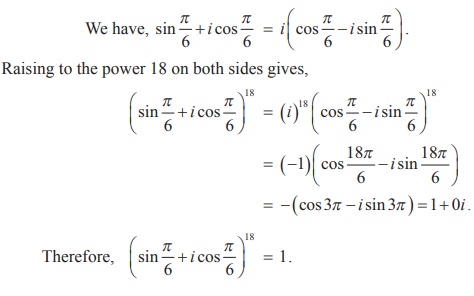
Example 2.30
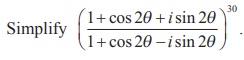
Solution
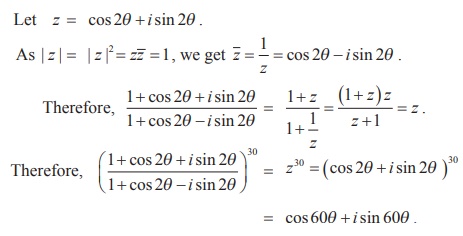
Example 2.31
Simplify
(i) (1+ i)18
(ii) (-ŌłÜ3 + 3i)31 .
Solution
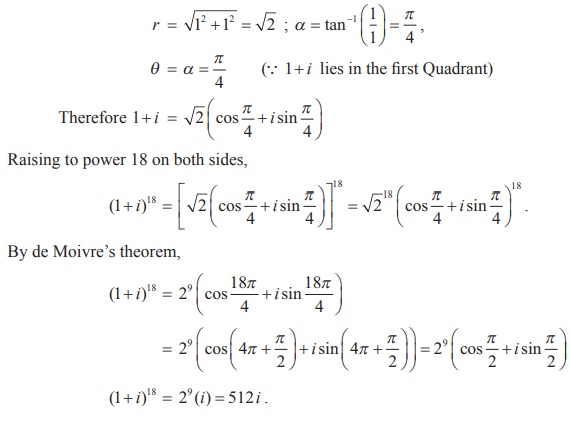
(i) (1+ i)18
Let 1+ i = r (cos╬Ė + i sin╬Ė ) . Then, we get
(ii) (-ŌłÜ3 + 3i)31 .
Let -ŌłÜ3 + 3i = r (cos╬Ė + i sin╬Ė ) . Then, we get
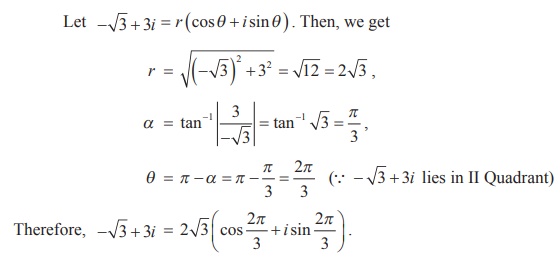
Raising power 31 on both sides,
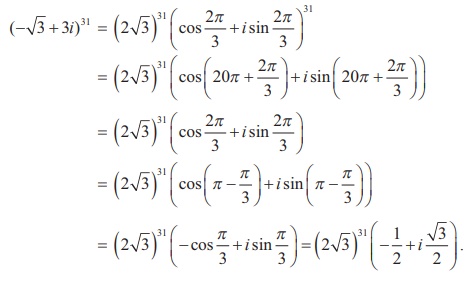
The nth roots of unity
Example 2.32
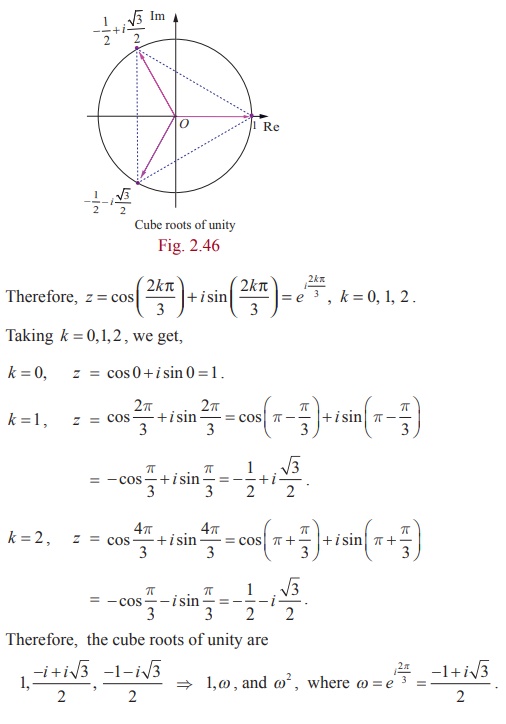
Find the cube roots of unity.
Solution
We have to find 11/3 . Let z = 11/3 then z3 = 1.
In polar form, the equation z3 = 1 can be written as
z3 = cos(0 + 2kŽĆ) + i sin(0 + 2kŽĆ) = ei2kŽĆ , k = 0, 1, 2,...
Example 2.33
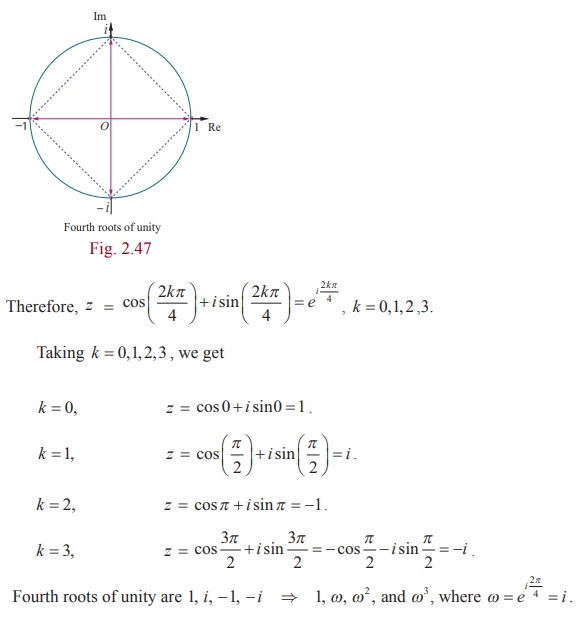
Find the fourth roots of unity.
Solution
We have to find 11/4. Let z =11/4 . Then z4 = 1 .
In polar form, the equation z4 = 1 can be written as
z4 = cos (0 + 2kŽĆ ) + i sin (0 + 2kŽĆ ) = ei2kŽĆ , k = 0, 1, 2,...
Note
(i) In this chapter the letter Žē is used for nth roots of unity. Therefore the value of Žē is depending on n as shown in following table.

(ii) The complex number zei╬Ė is a rotation of z by ╬Ė radians in the counter clockwise direction about the origin.
Example 2.34
Solve the equation z3 + 8i = 0 , where z Ōłł C.
Solution
Let z3 + 8i = 0 . Then, we get
z3 = -8i
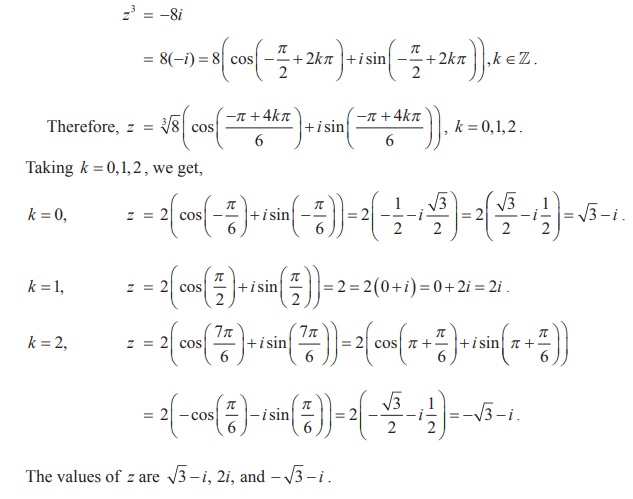
Example 2.35
Find all cube roots of ŌłÜ3 + i
Solution
We have to find (ŌłÜ3 + i)1/3. Let z3 = ŌłÜ3 + i = r (cos╬Ė + i sin╬Ė )
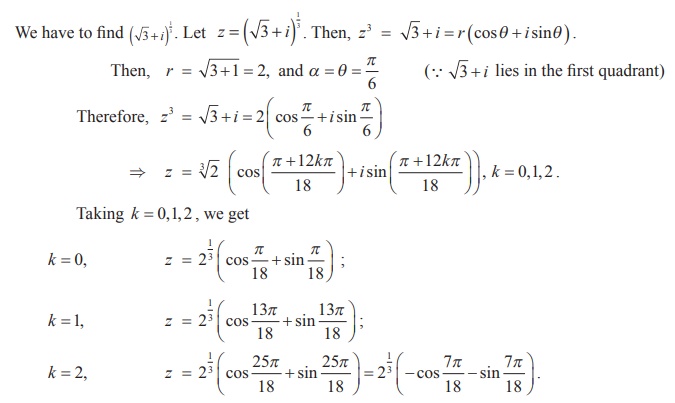
Example 2.36
Suppose z1 , z2, and z3 are the vertices of an equilateral triangle inscribed in the circle |z| = 2. If z1 = 1+ iŌłÜ3 , then find z2 and z3.
Solution
|z| = 2 represents the circle with centre (0, 0) and radius 2.
Let A, B, and C be the vertices of the given triangle. Since the vertices z1 , z2 , and z3 form an equilateral triangle inscribed in the circle |z| = 2 , the sides of this triangle AB, BC, and CA subtend 2ŽĆ/3 radians (120 degree) at the origin (circumcenter of the triangle).
(The complex number z ei╬Ė is a rotation of z by ╬Ė radians in the counter clockwise direction about the origin.)
Therefore, we can obtain z2 and z3 by the rotation of z1 by 2ŽĆ/3 and 4 ŽĆ/3 respectively.
Given that
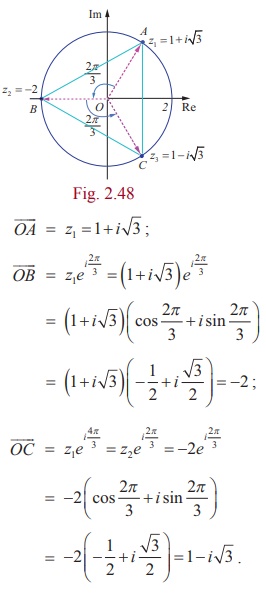
Therefore, z2 = -2, and z3 = 1- iŌłÜ3.
Related Topics