Introduction to Complex Numbers

Introduction to Complex Numbers

Before introducing complex numbers, let us try to answer the question “Whether there exists a real number whose square is negative?” Let’s look at simple examples to get the answer for it. Consider the equations 1 and 2.

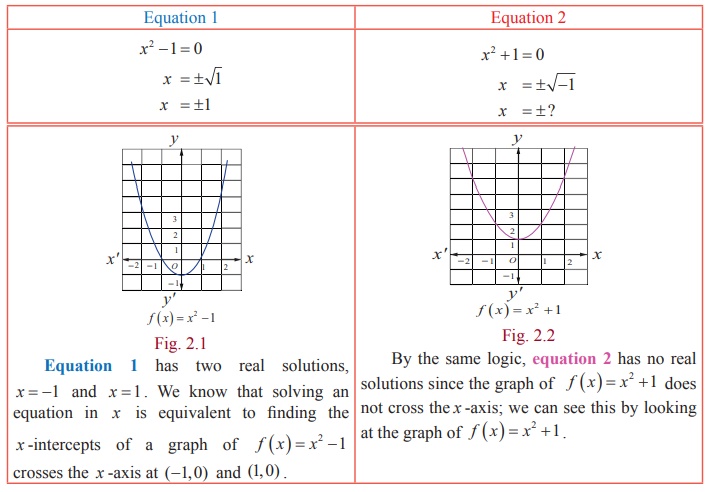
This is because, when we square a real number it is impossible to get a negative real number.

If equation 2 has solutions, then we must create an imaginary number as a square root of −1. This imaginary unit √−1 is denoted by i .The imaginary number i tells us that i² = −1. We can use this fact to find other powers of i . 

Powers of imaginary unit i

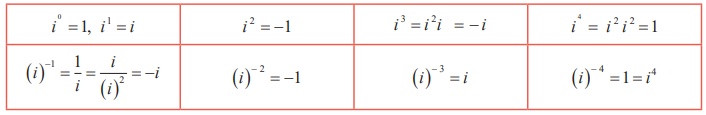
We note that, for any integer n , iⁿ  has only four possible values:  they correspond to values of n when divided by 4 leave the remainders 0, 1, 2, and 3.That is when the integer n ≤ −4  or  n ≥ 4 , using division algorithm, n can be written as n = 4q + k, 0 ≤ k < 4, k and q are integers and we write

 i)ⁿ = (i)^4q+k = (i)^4q(i)^k = ((i)⁴)^q(i)^k =  (i) ^q(i)^k =(i)^k

Example 2.1

Simplify the following 

(i) i⁷ (ii) i¹⁷²⁹ (iii) i ⁻¹⁹²⁴ + i²⁰¹⁸ (iv)  (v) i i² i³ ….. i⁴⁰ 

Solution

(i) (i)⁷ = (i)⁴⁺³ = (i)³ = -i;

(ii) i¹⁷²⁹ = i¹⁷²⁹i¹ = i

(iii) (i)^-1924 + (i)²⁰¹⁸ = (i)^-1924+0 + (i)²⁰¹⁶⁺² = (i)⁰ + (i)² = 1-1 = 0

(iv)  = (i¹ + i² + i³ + i⁴) + (i⁵ + i⁶ + i⁷ + i⁸ ) + … + (i⁹⁷ + i⁹⁸ + i⁹⁹ + i¹⁰⁰ +) + i¹⁰¹ + i¹⁰²

= (i¹ + i² + i³ + i⁴) + (i¹ + i² + i³ + i⁴) + … + (i¹ + i² + i³ + i⁴) + i¹ + i²

= {i+(-1)+(-i)+1} + {i+(-1)+(-i)+1} + …. … + {i+(-1)+(-i)+1} + i + (-1)

= 0 + 0 + … 0 + i -1

= −1 + i (What is this number?)

(v) i i² i^{3 …} i⁴⁰ = i^1+2+3+…+40 = i ^[40x41]/2 = i⁸²⁰ = i⁰ = 1

Note

(i) √[ab] = √a√b valid only if at least one of a, b is non-negative.

For example, 6 = √36 = √[(-4)(-9)] = √(-4) √ (-9) = (2i) (3i) = 6i² = -6, a contradiction.

Since we have taken √[(-4)(-9)] = √(-4) √ (-9), we arrived at a contradiction. 

Therefore √[ab] = √a √b valid only if at least one of a b, is non-negative.

(ii) For y ∈ R , y² ≥ 0

 iy = yi.