Problem Questions with Answer, Solution - Exercise 2.6: Geometry and Locus of Complex Numbers | 12th Mathematics : UNIT 2 : Complex Numbers
Chapter: 12th Mathematics : UNIT 2 : Complex Numbers
Exercise 2.6: Geometry and Locus of Complex Numbers
EXERCISE 2.6
1. If z = x + iy is a complex number such that 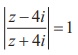
show that the locus of z is real axis.
2. If z = x + iy is a complex number such that Im 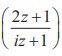 = 0 , show that the locus of z is
= 0 , show that the locus of z is
2x2 + 2 y2 + x - 2 y = 0.
3. Obtain the Cartesian form of the locus of z = x + iy in each of the following cases:
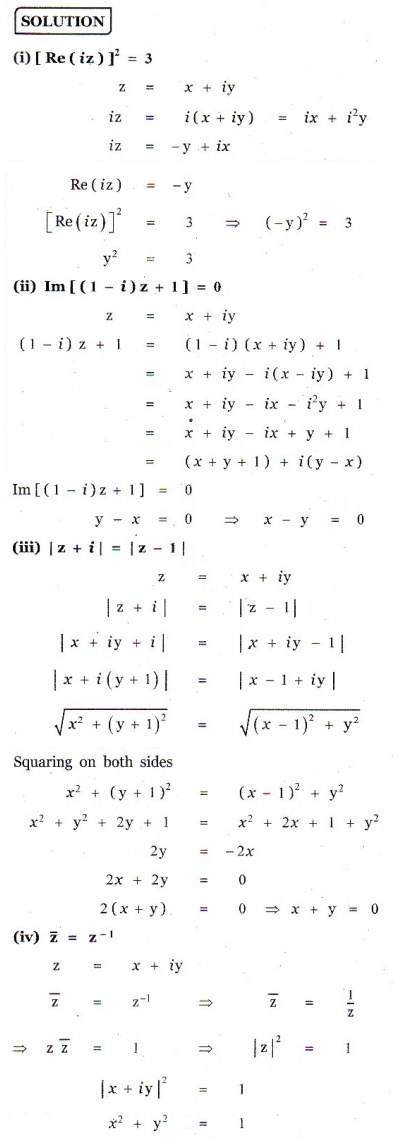
(i) [Re (iz )]2 = 3
(ii) Im[(1- i)z +1] = 0
(iii) |z + i| = |z -1|
(iv) ![]() = z-1.
= z-1.
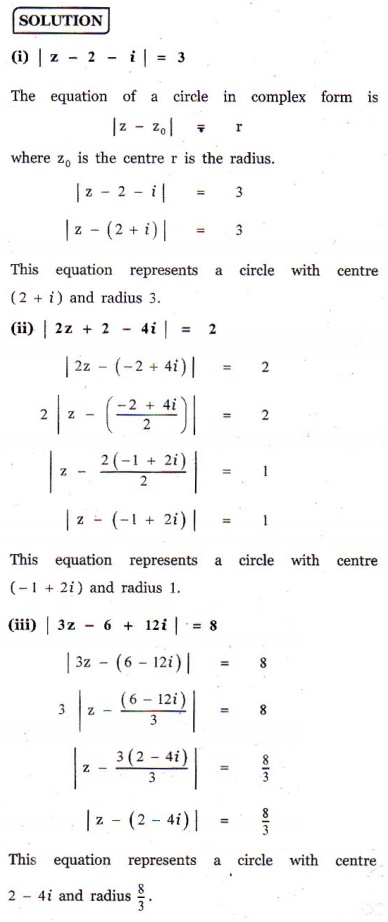
4. Show that the following equations represent a circle, and, find its centre and radius.
(i) |z - 2 – i| = 3
(ii) |2z + 2 - 4i| = 2
(iii) |3z - 6 +12i| = 8
5. Obtain the Cartesian equation for the locus of z = x + iy in each of the following cases:
(i) |z – 4| = 16
(ii) |z – 4|2 - |z -1|2 = 16 .
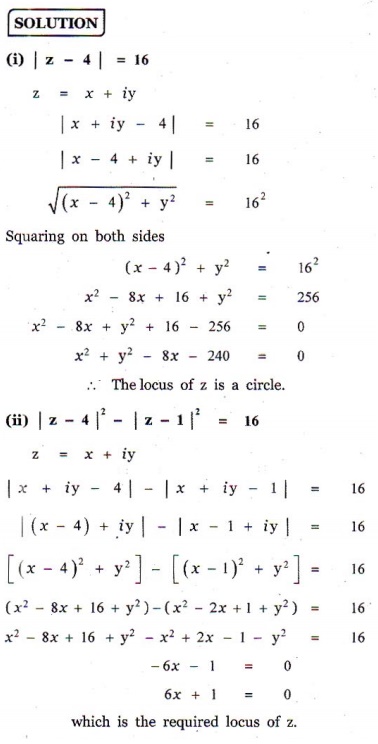
Answers:
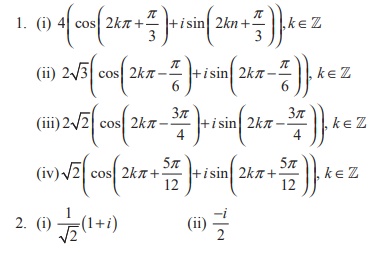
Related Topics