with Answers, Solution - Modulus of a Complex Number: Solved Example Problems | 12th Mathematics : UNIT 2 : Complex Numbers
Chapter: 12th Mathematics : UNIT 2 : Complex Numbers
Modulus of a Complex Number: Solved Example Problems
Example 2.9
If z1 = 3 + 4i, z2 = 5 -12i, and z3 = 6 + 8i, find
|z1| , |z2|, |z3|, |z1 + z2| ,| z2 - z3|, and |z1 + z3|.
Solution
Using the given values for z1, z2 and z3 we get |z1| = |3 + 4i| = ŌłÜ[32 +42] =5
|z2| = |5 -12i| = ŌłÜ[52 + (-12)2] = 13
z3 = |6 + 8i| = ŌłÜ[62 + 82] = 10
|z1 + z2| = |(3 + 4i ) + (5 -12i)| = |8 - 8i| =
ŌłÜ128 = 8ŌłÜ2
|z2 - z3| = |(5 -12i ) - (6 + 8i )| = |-1- 20i| =
ŌłÜ401
|z1 + z3| = |(3 + 4i) + (6 + 8i)| = |9 +12i| =
ŌłÜ225 = 15
Note that the triangle inequality is satisfied in all the cases.
|z1 + z3| = |z1| + |z3|= 15 (why?)
Example 2.10
Find the following
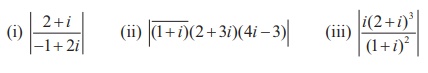
Solution
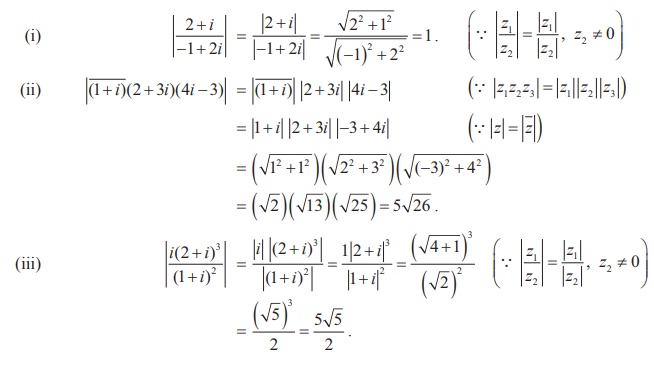
Example 2.11
Which one of the points i, -2 + i , and 3 is
farthest from the origin?
Solution
The distance between origin to z = i, -2 + i,
and 3 are
| z | = | i | = 1
| z | = | -2 + i |= ŌłÜ[(-2)2+(1)2] = ŌłÜ5
= | z | = | 3 | = 3
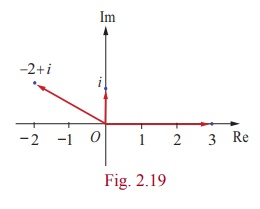
Since 1 < ŌłÜ5 < 3 , the farthest point from the origin is 3
Example 2.12
If z1, z2, and z3 are complex numbers such that |z1| = |z2| =|z3| = |z1 + z2 + z3| = 1, find the value of 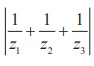 .
.
Solution
Since, |z1| = |z1| = |z1| = 1,
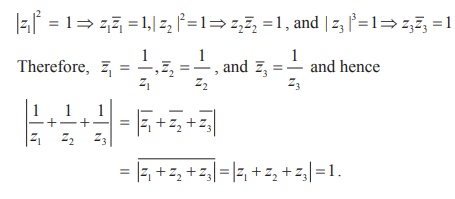
Example 2.13
If |z| = 2 show that 3 Ōēż |z + 3 + 4i| Ōēż 7
Solution
|z + 3 + 4i| Ōēż |z| + |3 + 4i| = 2 + 5 = 7
|z + 3 + 4i| Ōēż 7 (1)
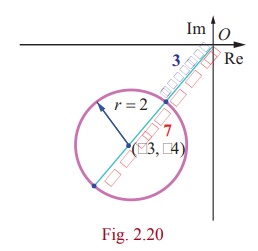
|z + 3 + 4i| Ōēź | |z| - | 3 + 4i| | = |2-5| = 3
|z + 3 + 4i| Ōēź 3
(2)
From (1) and (2), we get 3 Ōēż |z + 3 + 4i| Ōēż 7
Note
To find the lower bound and upper bound use | |z1| - |z2| | Ōēż |z1 + z2 | Ōēż |z1| + |z2|.
Example 2.14
Show that the points 1, 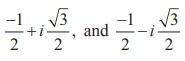 are
the vertices of an equilateral triangle.
are
the vertices of an equilateral triangle.
Solution
It is enough to prove that the sides of the triangle are equal.
Let z = 1,
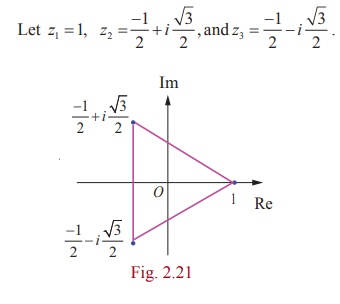
The length of the sides of the triangles are
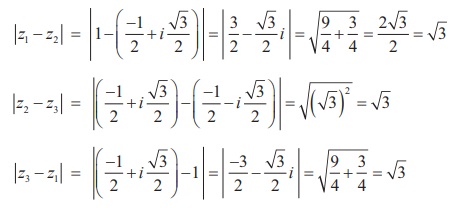
Since the sides are equal, the given points form an equilateral
triangle.
Example 2.15
Let z1 , z2 , and z3 be complex numbers such that |z1| = |z2| =|z3| = r > 0 and z1 + z2 + z3 ŌēĀ 0 .
Prove that 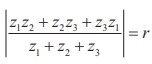 .
.
Solution
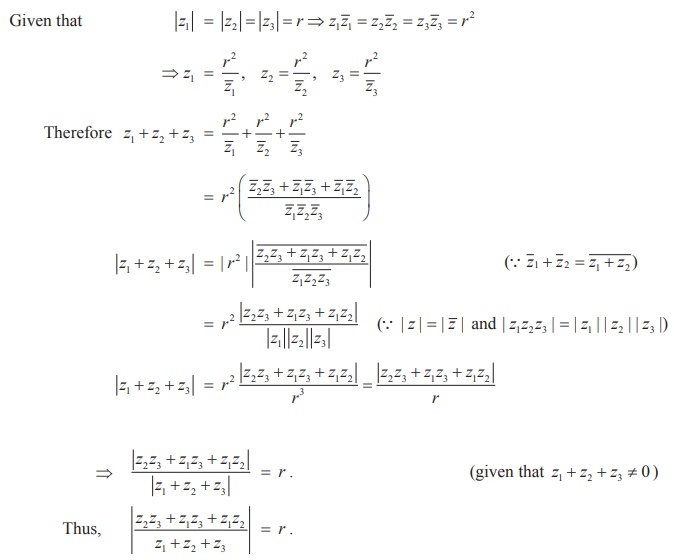
Example 2.16
Show that the equation z2 = ![]() has four solutions.
has four solutions.
Solution
We have,

It has 3 non-zero solutions. Hence including zero solution, there
are four solutions.
Related Topics