Problem Questions with Answer, Solution - Exercise 5.4: Tangents and Normals to Conics | 12th Mathematics : UNIT 5 : Two Dimensional Analytical Geometry II
Chapter: 12th Mathematics : UNIT 5 : Two Dimensional Analytical Geometry II
Exercise 5.4: Tangents and Normals to Conics
EXERCISE 5.4
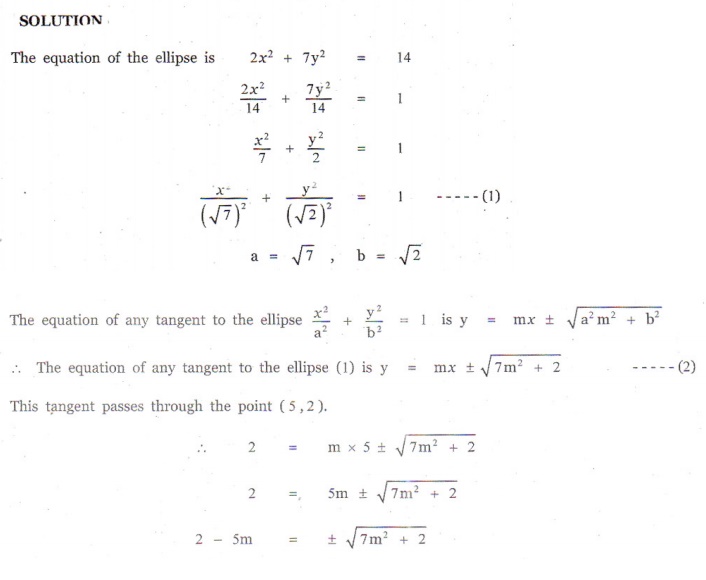
1. Find the equations of the two tangents that can be drawn from (5, 2) to the ellipse 2x2 + 7 y2 = 14 .

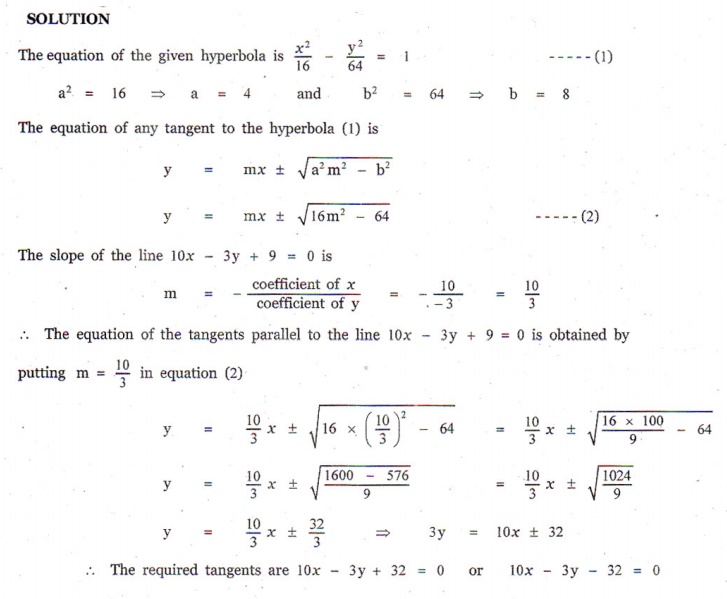
2. Find the equations of tangents to the hyperbola  which are parallel to 10x − 3y + 9 = 0.
which are parallel to 10x − 3y + 9 = 0.
3. Show that the line x − y + 4 = 0 is a tangent to the ellipse x2 + 3y2 = 12 . Also find the coordinates of the point of contact.

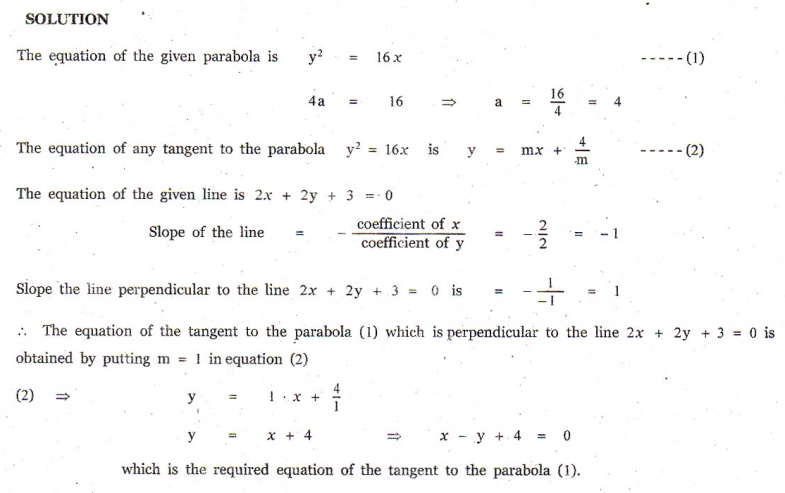
4. Find the equation of the tangent to the parabola y2 = 16x perpendicular to 2x + 2 y + 3 = 0 .
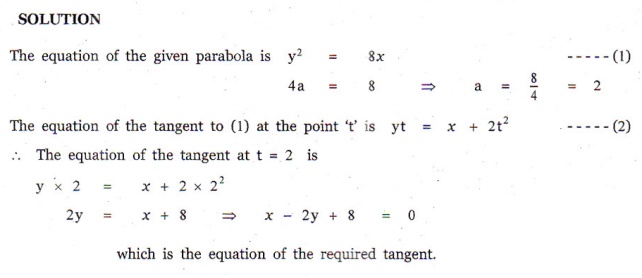
5. Find the equation of the tangent at t = 2 to the parabola y2 = 8x . (Hint: use parametric form)
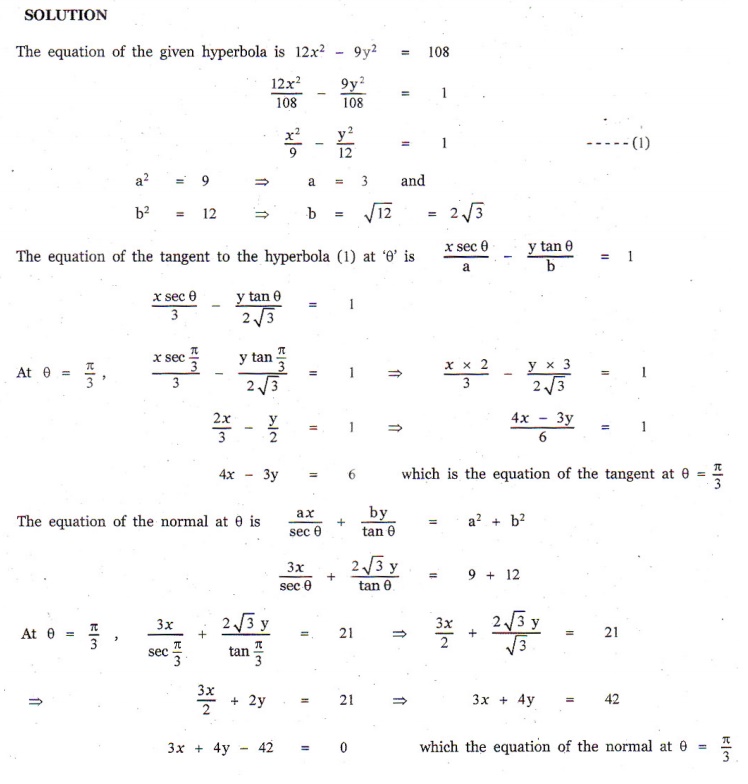
6. Find the equations of the tangent and normal to hyperbola 12x2 − 9 y2 = 108 at θ = π/3. (Hint: use parametric form)
7. Prove that the point of intersection of the tangents at ‘ t1 ’ and ‘ t2 ’on the parabola y2 = 4ax is [at1t2 , a (t1 + t2 )].

8. If the normal at the point ‘ t ’ on the parabola y2 = 4ax meets the parabola again at the point ‘ t ’, then prove that t2= - ( t + 2/t1).

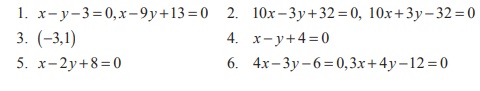
Related Topics