Equation of a plane progressive wave

Progressive wave

A progressive wave is defined as the onward transmission of the vibratory motion of a body in an elastic medium from one particle to the successive particle.

Equation of a plane progressive wave

An equation can be formed to represent generally the displacement of a vibrating particle in a medium through which a wave passes. Thus each particle of a progressive wave executes simple harmonic motion of the same period and amplitude differing in phase from each other.

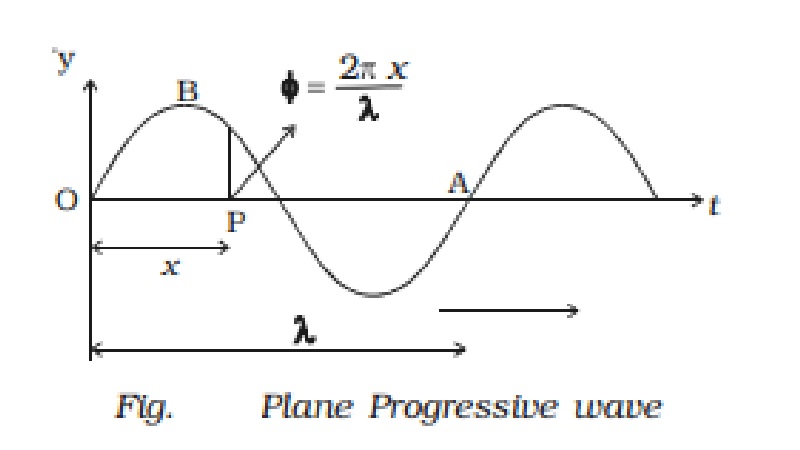
Let us assume that a progressive wave travels from the origin O along the positive direction of X axis, from left to right (Fig. 7.6). The displacement of a particle at a given instant is

y = a sin ωt ... (1)

 where a is the amplitude of the vibration of the particle and ω = 2πn.

The displacement of the particle P at a distance x from O at a given instant is given by,

y = a sin (ωt - φ)  ?.(2)

If the two particles are separated by a distance λ, they will differ by a phase of 2π. Therefore, the phase φ of the particle P at a distance

x is φ = 2π/λ  .  x

y = a sin (ωt - 2πx/ λ)

Since ω = 2πn = 2π v / λ, the equation is given by

y = a sin(2πt/ λ - 2πx/ λ)

y = a sin( 2π/ λ .(vt-x) )   ???..(4)

Since ω = 2π/ T , the eqn. (3) can also be written as

y = a sin 2π (t/T ? x/ λ)

If the wave travels in opposite direction, the equation becomes.

 y = a sin 2π(t/T + x/ λ)   ???(6)

(i) Variation of phase with time

The phase changes continuously with time at a constant distance.

At a given distance x from O let φ_ and φ₂ be the phase of a particle at time t₁ and t₂ respectively.

φ₁ = 2π(t₁/T ? x/ λ)

φ₂ = 2π(t₂/T ? x/ λ)

φ₂ - φ₁ = 2π(t₂/T  - t₁/T)

∆φ = 2π /T ∆t

This is the phase change ∆φ of a particle in time interval ∆t. If ∆t = T, ∆φ = 2π. This shows that after a time period T, the phase of a particle becomes the same.

(ii) Variation of phase with distance

At a given time t phase changes periodically with distance x. Let φ₁ and φ ₂ be the phase of two particles at distance x₁ and x₂ respectively from the origin at a time t.

φ₁ = 2π(t/T ? x₁/ λ)

φ₂ = 2π(t/T ? x₂/ λ)

φ₂ - φ₁ = 2π/ λ  (x₂ - x₁)

∆φ = 2π / λ ∆t

The negative sign indicates that the forward points lag in phase when the wave travels from left to right.

When ∆x = λ, ∆φ = 2π, the phase difference between two particles having a path difference λ is 2π.