Bohr atom model

Bohr atom model

Neils Bohr in 1913, modified Rutherford's atom model in order to explain the stability of the atom and the emission of sharp spectral lines. He proposed the following postulates :

(i) An electron cannot revolve round the nucleus in all possible orbits. The electrons can revolve round the nucleus only in those allowed or permissible orbits for which the angular momentum of the electron is an integral multiple of h/2π (where h is Planck's constant = 6.626 × 10^-34 Js). These orbits are called stationary orbits or non-radiating orbits and an electron revolving in these orbits does not radiate any energy.

If m and v are the mass and velocity of the electron in a permitted orbit of radius r then angular momentum of electron = mvr = nh / 2 π, where n is called principal quantum number and has the integral values 1,2,3

This is called Bohr's quantization condition.

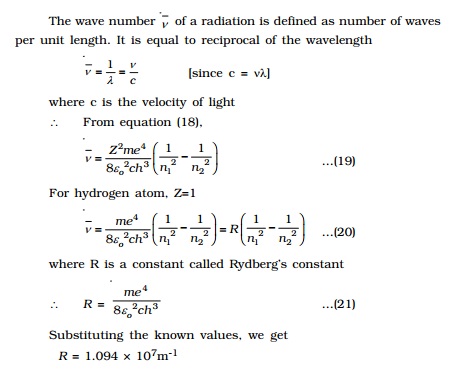
ii) An atom radiates energy, only when an electron jumps from a stationary orbit of higher energy to an orbit of lower energy. If the electron jumps from an orbit of energy E₂ to an orbit of energy E₁, a photon of energy hν = E₂ - E₁ is emitted. This condition is called Bohr's frequency condition.

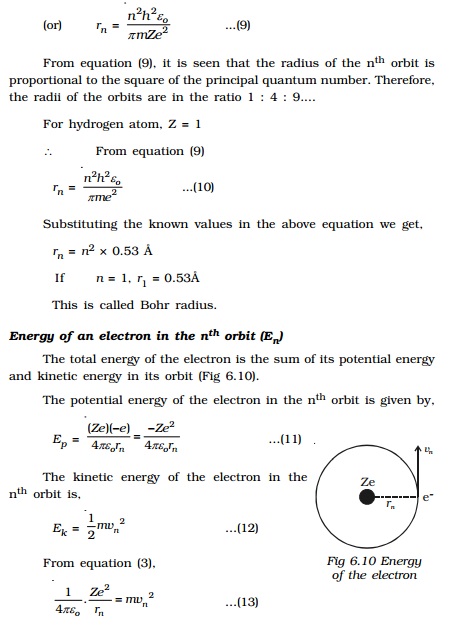
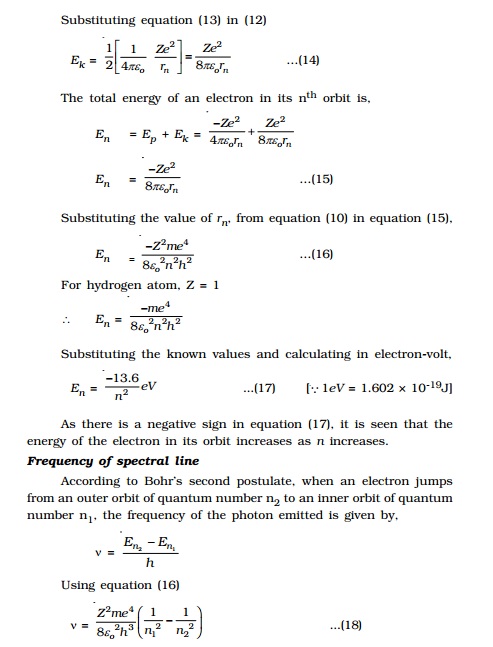
Radius of the n^th orbit (r_n )

Consider an atom whose nucleus has a positive charge Ze, where Z is the atomic number that gives the number of protons in the nucleus and e, the charge of the electron which is numerically equal to that of proton. Let an electron revolve around the nucleus in the n^th orbit of radius r_n.

By Coulomb's law, the electrostatic force of attraction between the nucleus and the electron = ( 1/ 4πε₀ ) .  ( Ze) (e ) / r²_n    ……. (1)

where ε_o is the permittivity of the free space.

Since, the electron revolves in a circular orbit, it experiences a centripetal force, mv²_n   / r_n  = mr_nω_n²  ………… (2)

where m is the mass of the electron, v_n and ω_n are the linear velocity and angular velocity of the electron in the n^th orbit respectively.

The necessary centripetal force is provided by the electrostatic force of attraction.

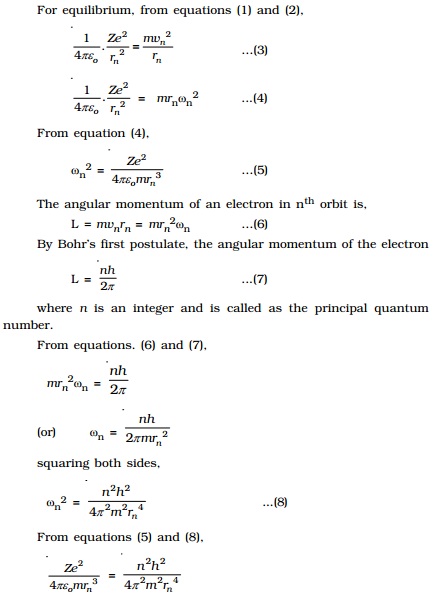
For equilibrium, from equations (1) and (2),

( 1/ 4πε₀ ) .  ( Ze) (e ) / r²_n  = mv²_n   / r_n