Numbers | Chapter 1 | 8th Maths - Basic Arithmetic Operations on Rational Numbers | 8th Maths : Chapter 1 : Numbers
Chapter: 8th Maths : Chapter 1 : Numbers
Basic Arithmetic Operations on Rational Numbers
Basic Arithmetic
Operations on Rational Numbers
All the rules
and principles that govern fractions in the basic operations apply to rational numbers
also.
1. Addition
There can
be four different situations while doing addition.
Type 1
: Adding numbers that have same denominators
This is simply
like adding like fractions and the result is the sum of the numerators divided by
their common denominator.
Example 1.10
Add : 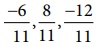
Solution:
Write the
given rational numbers in the standard form and then add them.
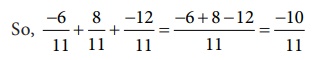
Type 2
: Adding numbers that have different denominators
After writing
the given rational numbers in the standard form, use the LCM of their denominators
to convert the numbers into equivalent rational numbers with a common denominator
so that this reduces to Type1.
Example 1.11
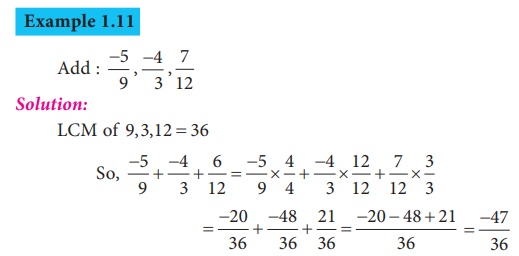
2. Additive
Inverse
The additive
inverse of a rational number is another rational number which when added to the given number, gives zero.
For example,
4/3 and -4/3
are additive inverses of each other, since their sum is zero.
Think
Is zero a rational number? If so, what is its additive inverse?
Solution: Yes zero is a national number. Additive inverse of
zero is zero.
3. Subtraction
Subtraction is simply adding the additive inverse.
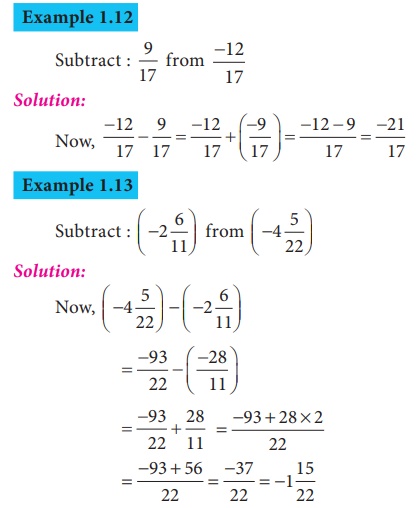
4. Multiplication
Product of two or more rational numbers is found by multiplying the corresponding numerators and denominators of the numbers and then writing them in the standard form.
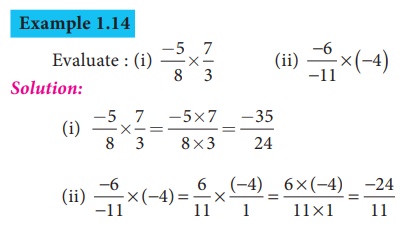
5. Multiplicative
Inverse
If the product
of two rational numbers is 1, then each of them is said to be the reciprocal or
the multiplicative inverse of the other.
Think
What is the multiplicative inverse of 1 and –1?
Solution: Multiplicative
inverse of 1 is 1 and −1 is −1.
For the rational
number a, its reciprocal is 1/a and vice versa since a × 1/a= 1/a × a = 1.
For the rational
number a/b , its multiplicative inverse
is b/a and vice versa since a/b × b/a
=
b/a × a/b
=
1.
6. Division
The idea
of reciprocals of fractions is extended to the division of rational numbers also.
To divide a given rational number by another rational number, we have to multiply
the given rational number by the reciprocal of the second rational number. That
is, division is simply multiplying by the multiplicative inverse of the divisor.


Solution:
(i) −7/3 ÷ 5 = [−7/3] ÷ [5/1] = [−7/3]
× [1/5] = −7/15
(ii) 5 ÷ (−7/3) = [5/1] × [3/−7] = 15/−7
= −2 (1/7)
(iii) −7/3 ÷ 35/62 = [−7/3] × [6/35] = −2/5
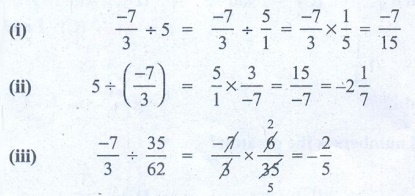
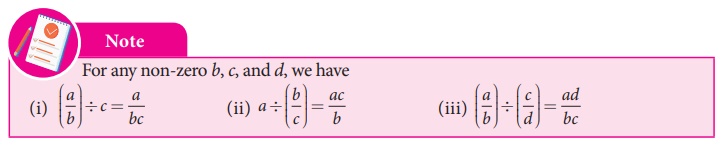
Related Topics