Chapter: 11th Chemistry : UNIT 6 : Gaseous State
Solved Numerical Problems: Gaseous State (Chemistry)
Gaseous State (Chemistry)
Numerical Problems Questions with Answers, Solution
44. A sample of gas at 15 °C at 1 atm. has a volume of 2.58 dm3. When the temperature is raised to 38 °C at 1 atm does the volume of the gas increase? If so, calculate the final volume.
Answer:
T1 = 15°C + 273
T2 = 38 + 273
T1 = 288 K
T2 = 311 K
V1 = 2.58 dm3
V2 = ?
(P = 1 atm constant)
Solution:
V1 / T1 = V2 / T2
V2 = V1/T1 × T2 = [2.58 dm3 / 288 K ] × 311 K
V2 = 2.78 dm3
ie., volume increased from 2.58 dm3 to 2.78 dm3
45. A sample of gas has a volume of 8.5 dm3 at an unknown temperature. When the sample is submerged in ice water at 0 °C, its volume gets reduced to 6.37 dm3. What is its initial temperature?
Answer:
Given
V1 = 8.5 dm3
V2 = 6.37 dm3
T1 = ?
T2 = 0°
C = 273 K
Solution:
V1 / T1 = V2 / T2
V1 × (T2 / V2) = T1
= T1
⇒ 8.5 dm3 × ( 273 K / 6.37 dm3 )
⇒ T1 = 364.28 K
46. Of two samples of nitrogen gas, sample A contains 1.5 moles of nitrogen in a vessel of volume of 37.6 dm3 at 298K, and the sample B is in a vessel of volume 16.5 dm3 at 298K. Calculate the number of moles in sample B.
Answer:
Given
nA = 1.5 mol
nB = ?
VA = 37.6 dm3
VB = 16.5 dm3
(T = 298K constant)
Solution :
VA / nA = VB / nB
nB = (nA / VA) VB
= (1.5 mol / 37.6 dm3 ) × 16.5 dm3 = 0.66 mol
47. Sulphur hexafluoride is a colourless, odourless gas; calculate the pressure exerted by 1.82 moles of the gas in a steel vessel of volume 5.43 dm3 at 69.5 °C, assuming ideal gas behaviour
Answer:
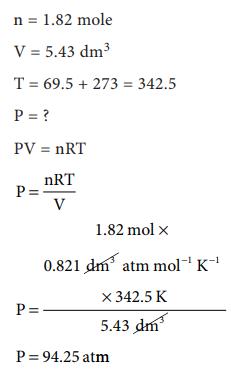
[Alternative Answer] Answers are in doubt
Given:
n = 1.82 mole
V = 5.43 dm3
T = 69.5 + 273 = 342.5
P = ?
PV = nRT
Solution:
P = nRT / V
P = [1.82 × 0.0821 × 342.5] / 5.43
P = 9.425 atm
48. Argon is an inert gas used in light bulbs to retard the vaporization of the tungsten filament. A certain light bulb containing argon at 1.2 atm and 18°C is heated to 85°C at constant volume. Calculate its final pressure in atm.
Answer:
Given:
P1 = 1.2 atm
T1 = 18°C + 273 = 291K
T2 = 85°C + 273 = 358 K
P2 = ?
Solution:
P1 / T1 = P2 / T2
P2 = (P1/T1 ) × T2
= (1.2 atm / 291K) × 358 K
P2 = 1.48 atm
49. A small bubble rises from the bottom of a lake where the temperature and pressure are 6°C and 4 atm. to the water surface, where the temperature is 25°C and pressure is 1 atm. Calculate the final volume in (mL) of the bubble, if its initial volume is 1.5 mL.
Answer:
Solution:
T1 = 6°C + 273 = 279 K
P1 = 4 atm
V1 = 1.5 ml
T2 = 25° C + 273 = 298 K
P2 = 1 atm
V2 = ?
P1V1 / T1 = P2V2 / T2
V2 = [ P1V1 / T1 ] × [T2 / P2]
= [ 4 atm × 1.5 ml × 298 K ] / [ 279 K × 1 atm ]
V2 = 6.41 mol
50. Hydrochloric acid is treated with a metal to produce hydrogen gas. Suppose a student carries out this reaction and collects a volume of 154.4 × 10-3 dm3 of a gas at a pressure of 742 mm of Hg at a temperature of 298 K. What mass of hydrogen gas (in mg) did the student collect?
Answer:
Given:
V = 154.4 × 10−3 dm3,
P = 742 mm of Hg
T = 298 K
m = ?
n = PV / RT
= [ 742 mm Hg × 154.4 × 10−3L ] / [ 62 mm Hg LK−1mol −1 × 298 K ]
= 0.006 mol
n = mass / Molar mass
Mass = n × Molar mass
= 0.006 × 2.016
= 0.0121g = 12.1 mg
51. It takes 192 sec for an unknown gas to diffuse through a porous wall and 84 sec for N2 gas to effuse at the same temperature and pressure. What is the molar mass of the unknown gas?
Answer:
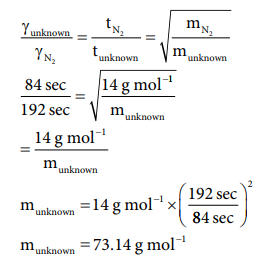
[Answers are in doubt ]
(84 / 192)2 = 28 g mol−1 / munknown
munknown = 28g mol−1 × (192 sec / 84 sec )2
munknown = 146.28 mol−1
52. A tank contains a mixture of 52.5 g of oxygen and 65.1 g of CO2 at 300 K the total pressure in the tanks is 9.21 atm. Calculate the partial pressure (in atm.) of each gas in the mixture.
Answer:
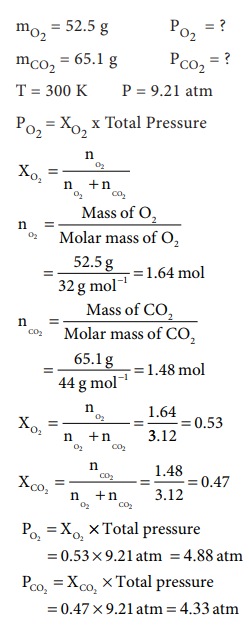
54. A combustible gas is stored in a metal tank at a pressure of 2.98 atm at 25 °C. The tank can withstand a maximum pressure of 12 atm after which it will explode. The building in which the tank has been stored catches fire. Now predict whether the tank will blow up first or start melting? (Melting point of the metal = 1100 K).
Answer:
Pressure of the gas in the tank at its melting point
T1 = 298K;
P1 = 2.98 atm ;
T2 = 1100 K;
P2 = ?
Solution:
P1/T1 = P2/T2
⇒ P2 = P1 × (T2 / T1)
= (2.98 atm × 1100 K ) / 298 K = 11 atm
At 1100 K the pressure of the gas inside the tank will become 11 atm. Given that tank can withstand a maximum pressure of 12 atm, the tank will start melting first.
Related Topics