Ionic Product Of Water : The pH of solutions

IONIC PRODUCT OF WATER

Water is a weak electrolyte. The dissociation equilibrium of water can be considered as,

2H₂O < --- -- > H₃O⁺ + OH^-

According to law of mass action,

K_eq = [H₃0⁺][OH^-]   /  [H₂0]²

Since water as a solvent is always in excess and change in concentration due its dissociation is negligible. Hence water concentration is assumed to be constant.

K_eq [H₂O]² = [H₃O⁺] [OH^- ] = K_w

The constant K_w is called as the ionic product of water and its value is given by the product of concentrations of hydronium (H₃O⁺) and hydroxide (OH^- ) ions. At 298 K, K_w = 1 ´ 10^-14 mol².dm^-6.

The pH of solutions

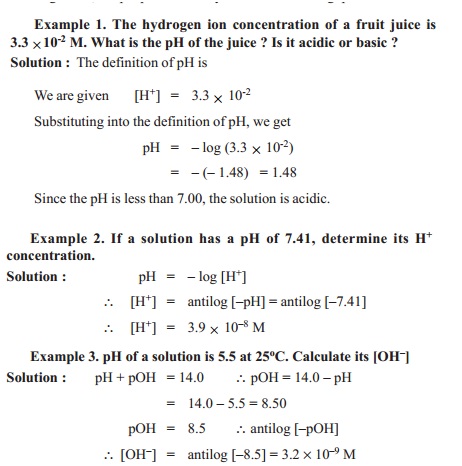
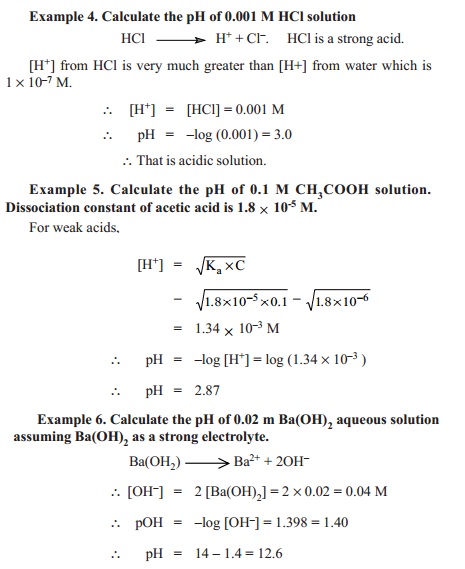
A knowledge of the concentration of hydrogen ions (more specifically hydronium ions) is of the greatest importance in chemistry. Hydrogen ion concentrations are typically quite small numbers. Therefore, chemists report the hydrogen ion concentration of a solution in terms of pH. It is defined as the negative of the base-10 logarithm (log) of the H⁺  concentration.

Mathematically it may be expressed as

pH = - log ₁₀ [H⁺]

where [H⁺] is the concentration of hydrogen ions in moles per litre Alternative and more useful forms of pH definition are :

pH = - log ₁₀ ( 1/[H⁺])

[H⁺] = 10^-pH

The pH concept is very convenient for expressing hydrogen ion concentration. It was introduced by Sorensen in 1909. It is now used as a general way of expressing other quantities also, for example.

(a) Concentration of OH^-  ions in aqueous solution of a base is expressed as

p [OH^-] = - log₁₀ [OH^-]

(b) Equilibrium constant for water is written as

pK_w       = - log ₁₀ [K_w]

For any quantity X, we can write

pX = - log X

The 'p' in these expressions means ''-log of the quantity''.

The pH of a given solution can be measured with the help of an apparatus called pH meter.

Knowing the pH of the solution its hydrogen ion concentration can be calculated.

pH Scale

In order to express the hydrogen ion concentration or acidity of a solution, a pH scale was evolved. The pH is defined as

pH = - log [H ⁺]        or      [H⁺] = 10^-pH

The hydrogen ion concentrations of different acidic solutions were determined experimentally. These were converted to pH values using the above relations. Then these pH values were computed on a scale taking water as the reference substance. The scale on which pH values are

computed is called the pH scale.

Water dissociates to H⁺ and OH^- ions to a very small degree so that we have the equilibrium.

H₂O  < --- -- > H⁺ + OH^-

Since      K_w = 1 ´ 10^-14 mol².dm^-6.

[H₃O⁺] = [H⁺] = [OH^- ] = sq.rt(1x10^-14) = 1x 10^-7mol.dm^-3

Thus the H⁺ ion and OH- ion concentrations in pure water are both 10^-7 mol.dm^-3 at 25^oC and it is said to be neutral. In acidic solution, however, the concentration of H⁺ ions must be greater than 10^-7 mol. L^-1. Similarly in a basic solution, the concentration of OH^- ions must be greater than 10^-7 mol L^-1. Thus we can state :

neutral solution [H⁺] = [OH^- ]

acidic solution [H⁺] > [OH^- ]

basic solution [H⁺] < [OH^- ]

Expressing the [H⁺] in terms of pH for the different solutions cited above, we get what we call the pH scale. On this scale the values range from 0 to 14. Since pH is defined as -log [H ⁺] and the hydrogen ion concentration of water is 10^-7, the pH of water is 7. All solutions having pH less than 7 are acidic and those with pH greater than 7 are basic.

pH=  1 < -- --- ACID ---- >  pH= 7  < -- BASE --- >  pH= 14

As shown by the pH scale, pH decreases with the increase of [H⁺]. The lower the pH, higher is the [H⁺] or acidity.

To calculate [H⁺] and [OH^- ] from K_w. In any aqueous solution, the product of [H⁺] and [OH^- ] always equal to Kw. This is so irrespective of the solute and relative concentrations of H⁺ and OH^- ions. However, the value of Kw depends on temperature. At 25^oC it is 1.0 ´ 10^-14. Thus,

[H⁺] [OH^- ] = 1.0 ´ 10^-14

Each of [H⁺] and [OH^- ] in pure water at 25^oC is 10^-7. The concentrations of [H⁺] and OH^- ions are expressed in gram moles per litre.

The concentrations [H⁺] and [OH^- ] ions can be calculated from the expressions :

[H⁺]  = K_{w / [OH}^-_]

[OH^- ]  = K_w  / [ H⁺ ]

Relation between pH and pOH

pH concept can be used to express small quantities as [OH^- ] and K_w. Thus

pOH = - log₁₀[OH^- ]

pK_w =   - log ₁₀ K_w

Let us consider the log form of the expression

K = [H⁺] [OH^- ]

That is log K = log [H⁺] + log [OH^- ]

- log K _w =   -log [H ⁺] - log [OH ^- ]

Thus pK_w = pH + pOH

Since K_w = 1.0 ´ 10^-14

pK_{w =} -log (1.0x10^-14 ) = 14.00

Hence, for any aqueous solution at 25^oC, pH and pOH add up to 14.00. That is,

pH + pOH = 14.00