Differentiability and Methods of Differentiation | Differential Calculus | Mathematics - Exercise 10.5: Choose the correct answer | 11th Mathematics : UNIT 10 : Differential Calculus: Differentiability and Methods of Differentiation
Chapter: 11th Mathematics : UNIT 10 : Differential Calculus: Differentiability and Methods of Differentiation
Exercise 10.5: Choose the correct answer
Differentiability and Methods of Differentiation - Differential Calculus (Mathematics)
Choose the correct or
the most suitable answer from the given four alternatives.
(1) d/ dx
(2/π sin x° ) or 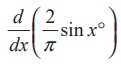 is
is
(1) π/180 . cos x°
(2) 1/90 . cos x°
(3) π/90 . cos x°
(4) 2/ π . cos x°
Ans: (2)
Solution
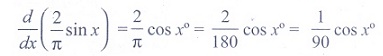
(2) If y = f (x2
+ 2) and f '(3) = 5, then dy/dx at x = 1 is
(1) 5
(2) 25
(3) 15
(4) 10
Ans: (4)
Solution
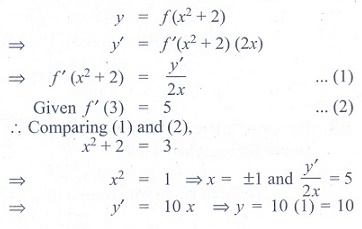
(3) If y = 1/4 u4
, u = 2/3 x3 + 5, then dy/dx is
(1) 1/ 27 x2
(2x3 +15)3
(2) 2/27 x (2x3
+ 5)3
(3) 2/ 27 x2
(2x3 +15)3
(4) 2/27 x (2x3
+ 5)3
Ans: (3)
Solution

(4) If f (x)
= x2 − 3x , then the points at which f (x) = f
'(x) are
(1) both positive
integers
(2) both negative
integers
(3) both
irrational
(4) one rational and
another irrational
Ans: (3)
Solution
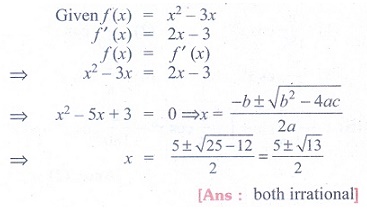
(5) If y = 1/ a − z ,
then dz/ dy is
(1) (a − z)2
(2) −( z − a)2
(3) (z + a)2
(4) − ( z + a)2
Ans: (1)
Solution
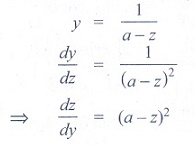
(6) If y = cos(sin
x2), then dy/dx at x = √[π/2] is
(1) - 2
(2) 2
(3) −2 √[π/2]
(4) 0
Ans: (4)
Solution

(7) If y = mx
+ c and f (0) = f '(0) = 1, then f (2) is
(1) 1
(2) 2
(3) 3
(4) - 3
Ans: (3)
Solution
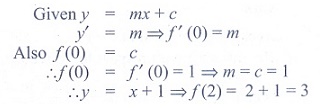
(8) If f (x)
= x tan−1x , then f '(1) is
(1) 1+ π/4
(2) 1/2 + π/4
(3) 1/2 – π/4
(4) 2
Ans: (2)
Solution
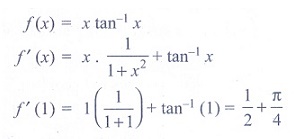
(9) d/
dx (ex +5logx) is
(1) ex
.x4 (x + 5)
(2) ex .x (x
+ 5)
(3) ex + 5/x
(4) ex
- 5/x
Ans: (1)
Solution
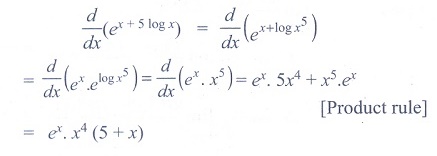
(10) If the derivative
of (ax − 5)e3x at x = 0 is -13, then the value of a is
(1) 8
(2) - 2
(3) 5
(4) 2
Ans: (4)
Solution
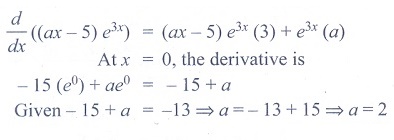
(11) 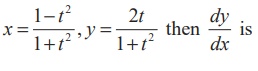
(1) −y /x
(2) y/x
(3) – x/y
(4) x/y
Ans: (3)
Solution
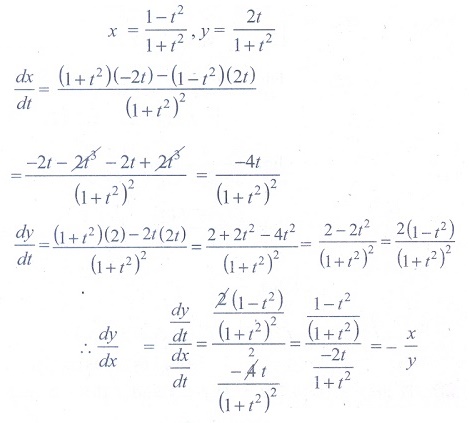
(12) If x = asinθ
and y = bcosθ , then d2y/ d2x
is
(1) a/ b2 sec2θ
(2) – b/a sec2θ
(3) –b/a2 sec3θ
(4) −b2/a2
sec3θ
Ans: (3)
Solution

(13) The differential
coefficient of log10x with respect to logx10
is
(1) 1
(2) −(log10x)2
(3) (logx10)2
(4) x2
/100
Ans: (2)
Solution
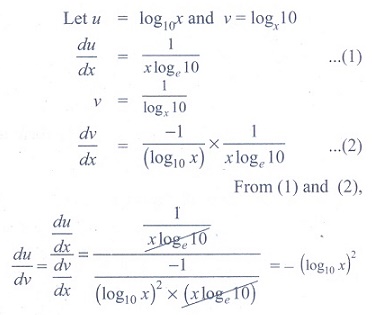
(14) If f (x)
= x + 2, then f '( f (x)) at x = 4 is
(1) 8
(2) 1
(3) 4
(4) 5
Ans: (2)
Solution
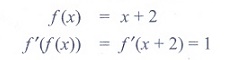
(15) If y = (1− x)2/
x2 , then dy/ dx is
(1) 2/x2
+ 2/x3
(2) −2/x2
+ 2/x3
(3) −2/x2
− 2/x3
(4) −2/x2
+ 2/x3
Ans: (4)
Solution

(16) If pv = 81,
then dp/dv at v = 9 is
(1) 1
(2) - 1
(3) 2
(4) -2
Ans: (2)
Solution
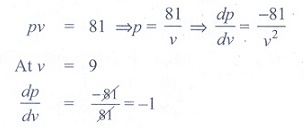
(17) If f (x
) = 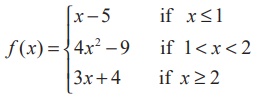 , then the right hand derivative of f(x) at x = 2
is
, then the right hand derivative of f(x) at x = 2
is
(1) 0
(2) 2
(3) 3
(4) 4
Ans: (3)
Solution
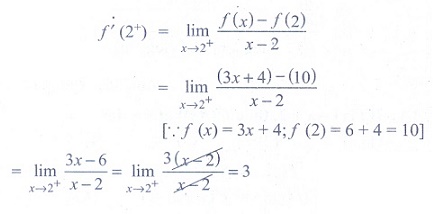
(18) It is given that f
'(a) exists, then lim x →a [xf (a ) − af (x)]/[
x − a] is
(1) f (a ) − af
'(a)
(2) f '(a)
(3) − f '(a)
(4) f (a ) + af '(a)
Ans: (1)
Solution
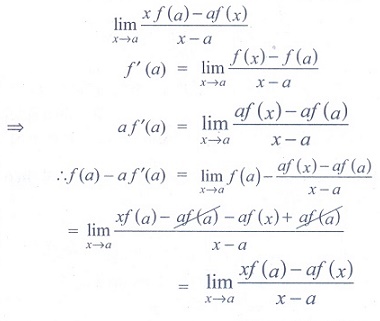
(19) If 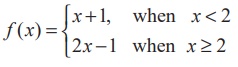 , then f '(2) is
, then f '(2) is
(1) 0
(2) 1
(3) 2
(4) does not exist
Ans: (4)
Solution
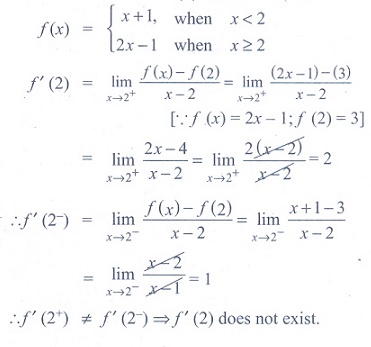
(20) If g (x)
= (x2 + 2x + 3) f (x) and f (0) = 5 and 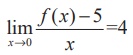 , then g '(0) is
, then g '(0) is
(1) 20
(2) 14
(3) 18
(4) 12
Ans: (2)
(21) If 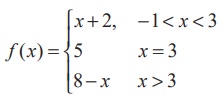 , then at x = 3, f '(x) is
, then at x = 3, f '(x) is
(1) 1
(2) - 1
(3) 0
(4) does not exist
Ans: (4)
Solution

(22) The derivative of f
(x) = x | x | at x = −3 is
(1) 6
(2) - 6
(3) does not exist
(4) 0
Ans: (1)
Solution

(23) If f (x) = 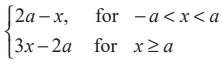 , then which one of the following is true?
, then which one of the following is true?
(1) f(x) is not
differentiable at x = a
(2) f(x) is
discontinuous at x = a
(3) f(x) is
continuous for all x in R
(4) f(x) is
differentiable for all x ≥ a
Ans: (1)
Solution
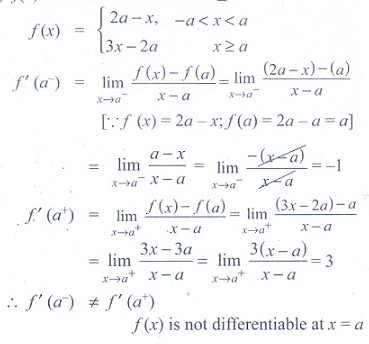
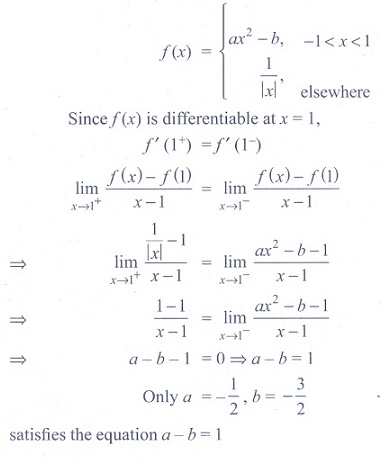
(24) If f (x) = 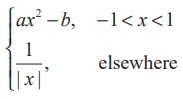 is differentiable at x = 1, then
is differentiable at x = 1, then
(1) a = 1/2 , b =
−3/2
(2) a = −1/2 , b =
3/2
(3) a = − 1/2 , b = −
3/2
(4) a = 1/2 , b =
3/2
Ans: (3)
Solution
(25) The number of
points in R in which the function f (x) =| x − 1| + | x −
3| + sin x is not differentiable, is
(1) 3
(2) 2
(3) 1
(4) 4
Ans: (2)
Solution
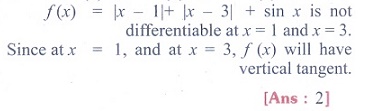
Related Topics