Chapter: Biochemistry: The Behavior of Proteins: Enzymes
Enzyme Inhibition
Enzyme Inhibition
An inhibitor, as the name implies, is a
substance that interferes with the action of an enzyme and slows the rate of a
reaction. A good deal of information about enzymatic reactions can be obtained
by observing the changes in the reaction caused by the presence of inhibitors.
Inhibitors can affect an enzymatic reaction in two ways. A reversible inhibitor
can bind to the enzyme and subsequently be released, leaving the enzyme in its
original condition. An irreversible inhibitor reacts with the enzyme to produce
a protein that is not enzymatically active and from which the original enzyme
cannot be regenerated.
Two
major classes of reversible inhibitors can be distinguished on the basis of the
sites on the enzyme to which they bind. One class consists of compounds very
similar in structure to the substrate. In this case, the inhibitor can bind to
the active site and block the substrateÕs access to it. This mode of action is
called competitive inhibition
because the inhibitor competes with the substrate for the active site on the
enzyme. Another major class of reversible inhibitors includes any inhibitor
that binds to the enzyme at a site other than the active site and, as a result
of binding, causes a change in the structure of the enzyme, especially around
the active site. The substrate is still able to bind to the active site, but
the enzyme cannot catalyze the reaction when the inhibitor is bound to it. This
mode of action is called noncompetitive
inhibition (Figure 6.11).
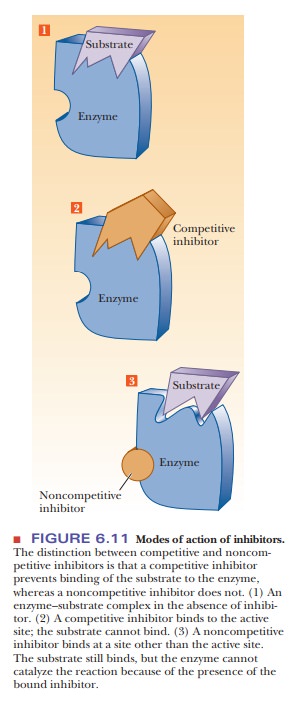
The two
kinds of inhibition can be distinguished from one another in the laboratory.
The reaction is carried out in the presence of inhibitor at several substrate
concentrations, and the rates obtained are compared with those of the
uninhibited reaction. The differences in the Lineweaver-Burk plots for the
inhibited and uninhibited reactions provide the basis for the comparison.
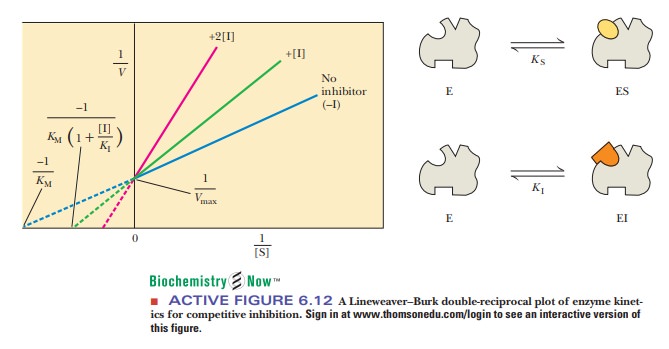
How can we identify a competitive inhibitor?
In the presence of a competitive inhibitor, the
slope of the Lineweaver-Burk plot changes, but the y intercept does not. (The x
intercept also changes.) Vmax
is unchanged, but KM
increases. More substrate is needed to get to a given rate in the presence of
inhibitor than in its absence. This point speciĂžcally applies to the speciĂžc
value Vmax/2 (recall that
at Vmax/2, the substrate
concentration, [S], equals KM)
(Figure 6.12). Competitive inhibition can be overcome by a sufĂžciently high
substrate concentration.
In the presence of a competitive inhibitor, the
equation for an enzymatic reaction becomes
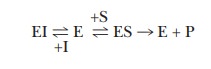
where EI is the enzyme-inhibitor complex. The
dissociation constant for the enzyme-inhibitor complex can be written
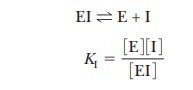
It can be shown algebraically (although we
shall not do so here) that, in the presence of inhibitor the value of KM increases by the factor
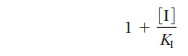
If we substitute KM (1 + [I]/KI)
for KM in Equation 6.17,
we obtain
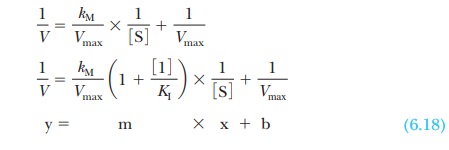
Here the term 1/V takes the place of the y
coordinate, and the term 1/[S] takes the place of the x coordinate, as was the case in Equation 6.17. The intercept 1/Vmax, the b term in the equation for a straight
line, has not changed from the earlier equation, but the slope KM/Vmax in Equation 6.17 has increased by the fac-tor (1 +
[I]/KI). The slope, the m term in the equation for a straight
line, is now
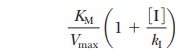
accounting for the changes in the slope of the
Lineweaver-Burk plot. Note that the y
intercept does not change. This algebraic treatment of competitive inhibition
agrees with experimental results, validating the model, just as experimental
results validate the underlying Michaelis-Menten model for enzyme action. It is
important to remember that the most distinguishing characteristic of a
competitive inhibitor is that substrate or inhibitor can bind the enzyme, but
not both. Because both are vying for the same location, sufĂžciently high
substrate will ÒoutcompeteÓ the inhibitor. This is why Vmax does not change; it is a measure of the velocity at
inĂžnite [substrate].
How can we identify a noncompetitive inhibitor?
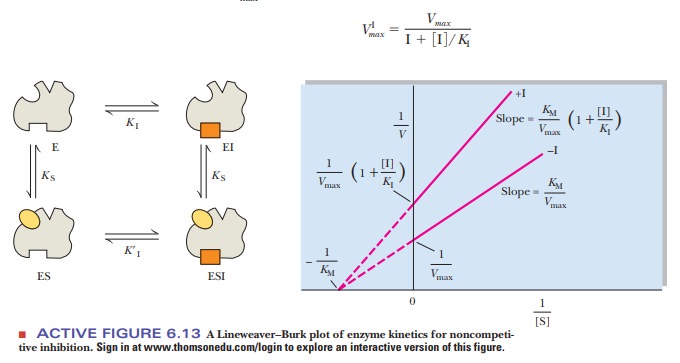
The kinetic results of noncompetitive
inhibition differ from those of competitive inhibition. The Lineweaver-Burk
plots for a reaction in the presence and absence of a noncompetitive inhibitor
show that both the slope and the y
intercept change for the inhibited reaction (Figure 6.13), without changing the
x intercept. The value of Vmax decreases, but that of KM remains the same; the
inhibitor does not interfere with the binding of substrate to the active site.
Increasing the substrate concentration cannot overcome noncompetitive
inhibition because the inhibitor and substrate are not competing for the same
site.
The reaction pathway has become considerably
more complicated, and sev-eral equilibria must be considered.

In the presence of a noncompetitive inhibitor,
I, the maximum velocity of the reaction, VImax,
has the form (we shall not do the derivation here)
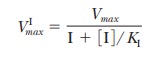
where KI
is again the dissociation constant for the enzyme-inhibitor complex, EI. Recall
that the maximum rate, Vmax,
appears in the expressions for both the slope and the intercept in the equation
for the Lineweaver-Burk plot (Equation 6.17):
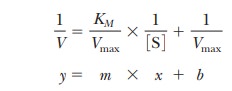
In noncompetitive inhibition, we replace the
term Vmax with the
expression for V Imax,
to obtain
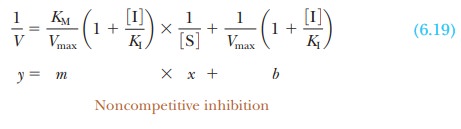
The expressions for both the slope and the
intercept in the equation for a Lineweaver-Burk plot of an uninhibited reaction
have been replaced by more complicated expressions in the equation that
describes noncompetitive inhibition. This interpretation is borne out by the
observed results. With a pure, noncompetitive inhibitor, the binding of
substrate does not affect the binding of inhibitor, and vice versa. Because the
KM is a measure of the
afĂžnity of the enzyme and substrate, and because the inhibitor does not affect
the binding, the KM does
not change with noncompetitive inhibition.
The two types of inhibition presented here are the two extreme cases. There are many other types of inhibition. Uncompetitive inhibition is seen when an inhibitor can bind to the ES complex but not to free E. A Lineweaver-Burk plot of an uncompetitive inhibitor shows parallel lines. The Vmax decreases and the apparent KM decreases as well. Noncompetitive inhibition is actually a limiting case of a more general inhibition type called mixed inhibition. With a mixed inhibitor, the same binding diagram is seen as in the preceding equilibrium equations but, in this case, the binding of inhibitor does affect the binding of substrate and vice versa. A Lineweaver-Burk plot of an enzyme plus mixed inhibitor gives lines that intersect in the left-hand quadrant of the graph. The KMincreases, and the Vmaxdecreases.
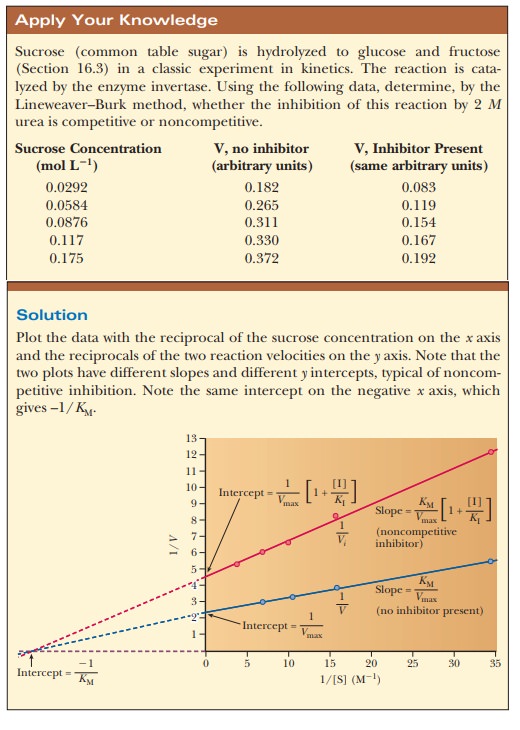
Summary
Inhibitors are compounds that bind to enzymes
and reduce the rate of catalysis.
Two principal types of inhibitors are
competitive and noncompetitive.
Competitive inhibitors bind to the active site
of an enzyme and prevent the simultaneous binding of substrate.
Noncompetitive inhibitors
bind to enzymes at a site other than the active site, but they alter the active
site in such a way to reduce the catalytic effi-ciency of the enzyme.
The type
of inhibition can be determined by using a Lineweaver-Burk plot.
Related Topics