Types of Angles, Transversal

Geometry Basics –Recall

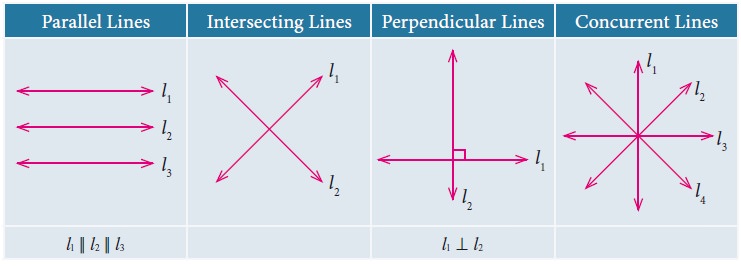
Draw two lines on a plane. They can be either parallel or intersecting.

Parallel lines        Two or more lines lying in the same plane that never meet.

Intersecting lines  Two lines which meet at a common point.

Perpendicular lines Two lines which intersect each other at right angle.

Concurrent lines  Three or more lines passing through the same point.

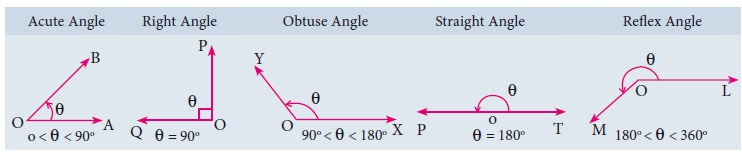
1. Types of Angles

Plumbers measure the angle between connecting pipes to make a good fitting. Wood workers adjust their saw blades to cut wood at the correct angle. Air Traffic Controllers (ATC) use angles to direct planes. Carom and billiards players must know their angles to plan their shots. An angle is formed by two rays that share a common end point provided that the two rays are non-collinear.

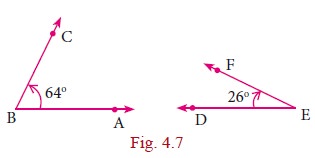
Complementary Angles

Two angles are Complementary if their sum is 90°. For example, if ∠ABC=64° and ∠DEF=26°, then angles ∠ABC and ∠DEF are complementary to each other because ∠ABC + ∠DEF = 90°

Supplementary Angles

Two angles are Supplementary if their sum is 180°.

For example if ∠ABC=110° and ∠XYZ=70°

Here ∠ABC + ∠XYZ = 180°

∴∠ABC and ∠XYZ are supplementary to each other

Adjacent Angles

Two angles are called adjacent angles if

i. They have a common vertex

ii. They have a common arm.

iii. The common arm lies between the two non-common arms.

Linear Pair of Angles

If a ray stands on a straight line then the sum of two adjacent angle is 180°. We then say that the angles so formed is a linear pair.

∠AOC + ∠BOC=180°

∴∠AOC and ∠BOC form a linear pair

∠XOZ + ∠YOZ = 180°

∠XOZ and ∠YOZ form a linear pair

Vertically Opposite Angles

If two lines intersect each other, then vertically opposite angles are equal.

In this figure ∠POQ = ∠SOR

 ∠POS = ∠QOR

2. Transversal

A line which intersects two or more lines at a distinct points is called a transversal of those lines.

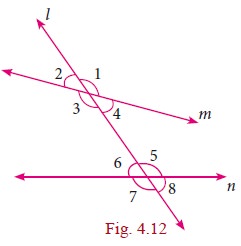
Case (i) When a transversal intersect two lines, we get eight angles.

In the figure the line l is the transversal for the lines m and n

(i) Corresponding Angles: ∠1 and ∠5, ∠2 and ∠6, ∠3 and ∠7, ∠4 and ∠8

(ii) Alternate Interior Angles:  ∠4 and ∠6, ∠3 and ∠5

(iii) Alternate Exterior Angles: ∠1 and ∠7, ∠2 and ∠8

(iv) ∠4 and ∠5,∠3 and ∠6 are interior angles on the same side of the transversal.

(v) ∠1 and ∠8, ∠2 and ∠7 are exterior angles on the same side of the transversal.

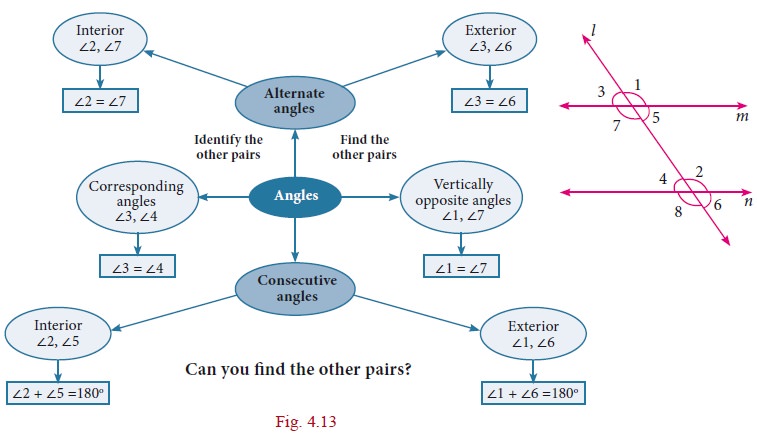
Case (ii) If a transversal intersects two parallel lines. The transversal forms different pairs of angles.

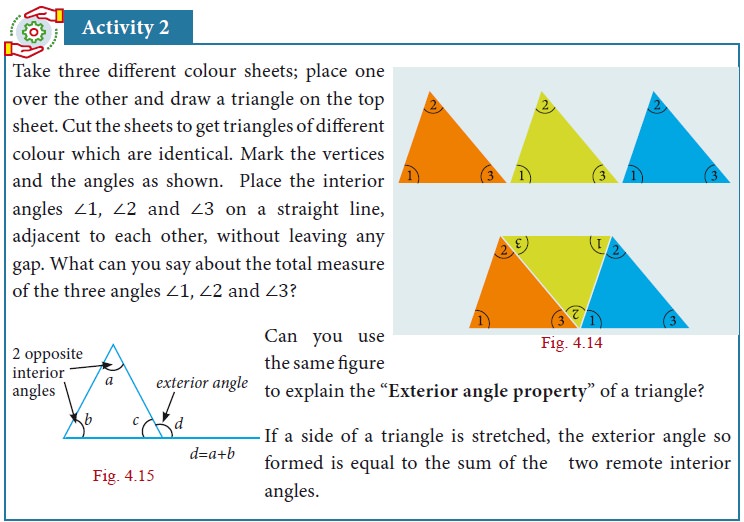
3. Triangles

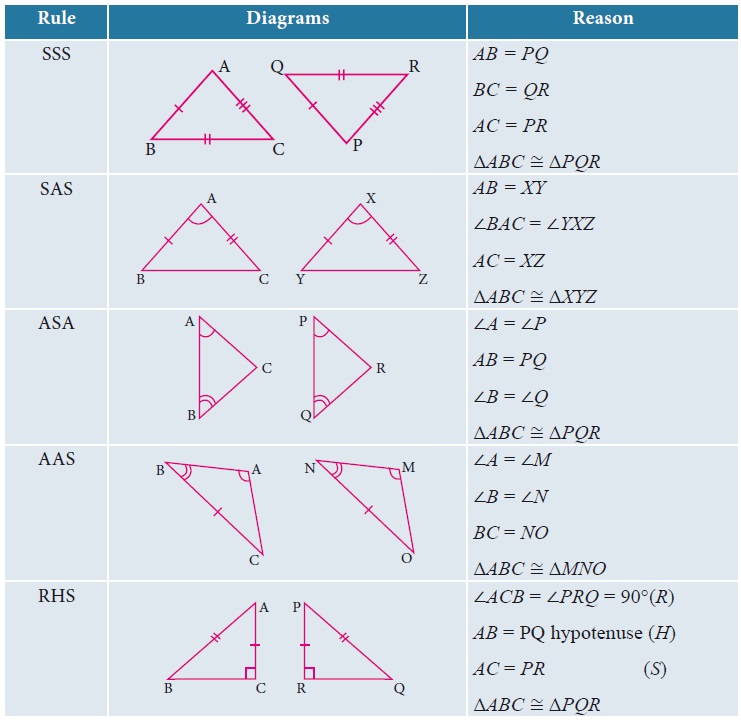
4. Congruent Triangles

Two triangles are congruent if the sides and angles of one triangle are equal to the corresponding sides and angles of another triangle.