Chapter: 11th Chemistry : UNIT 8 : Physical and Chemical Equilibrium
Solved Numerical Example Problems: Physical and Chemical Equilibrium
Physical and Chemical Equilibrium | Chemistry
Numerical Problems Questions with Answers, Solution
Example 1
The value of Kc for the following reaction at 717 K is 48.
H2(g) + I2(g) ⇌ 2HI(g)
At a particular instant, the concentration of H2, I2and HI are found to be 0.2 mol L-1, 0.2 mol L-1 and 0.6 mol L-1 respectively. From the above information we can predict the direction of reaction as follows.
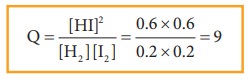
Since Q< Kc, the reaction will proceed in the forward direction.
Example 2
The value of Kc for the reaction
N2O4 (g) ⇌ 2NO2(g)
Kc = 0.21 at 373 K. The concentrations N2O4 and NO2 are found to be 0.125 mol dm-3 and 0.5 mol dm-3 respectively at a given time. From the above information we can predict the direction of reaction as follows.
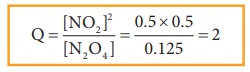
The Q value is greater than Kc. Hence, the reaction will proceed in the reverse direction until the Q value reaches 0.21
28. What the relation between KP and KC. Give one example for which KP is equal to KC.
The relation between equilibrium constants KP and Kc are given by
KP= Kc (RT)Δng
Δng = 2 – 2 = 0
H2(g) + I2(g) → 2HI(g)
KP = KC
29. For a gaseous homogeneous reaction at equilibrium, number of moles of products are greater than the number of moles of reactants. Is KC is larger or smaller than KP.
For a gaseous homogenious equilibrium reaction at equilibrium, number of moles of products are greater than the number of moles of reactants.
For example PCl5(g) ⇌ PCl3(g) + Cl2(g)
Δng = 2 − 1 = 1 positive
The relation between KP & KC for above reaction is KP > KC. Hence KC is smaller than KP.
30. When the numerical value of the reaction quotient (Q) is greater than the equilibrium constant (K), in which direction does the reaction proceed to reach equilibrium ?
When the numerical value of reaction quotient Q is greater then the equilibrium constant KC. Q > KC. The equilibrium shift to the left and produce more reactants.
31. For the reaction,
A2(g) + B2(g) ⇌ 2AB(g) ; ΔH is –ve.
the following molecular scenes represent different reaction mixture (A – green, B – blue)
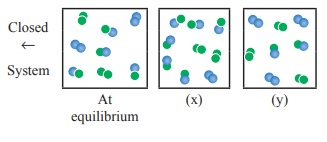
i) Calculate the equilibrium constant KP and (KC).
ii) For the reaction mixture represented by scene (x), (y) the reaction proceed in which directions ?
iii) What is the effect of increase in pressure for the mixture at equilibrium.
Solution:
i) KC= [AB]2 / [A2] [B2]
A – Green
B – blue
Given that 'V' is constant (closed system)
At equilibrium
∴KC = [ (4/V)2 ] / [ (2/V) (2/V) ] = 16 / 4 = 4
KP = KC (RT)Δng
KP = 4 (RT)o = 4
ii) At Stage 'x'
Q = ( [AB]2 ) / ( [A2] [B2] ) = (6/V)2 / ( (2/V) (1/V) ) = 36/2 = 18
Q > KC ie., reverse reaction is favoured
iii) At stage 'y'
Q = (3/V)2 / ( (3/V)(3/V) ) = 9 / (3× 3) = 1
KC > Q ie., forward reaction is favoured.
33. Consider the following reactions,
a) H2(g) + I2(g) ⇌ 2 HI
b) CaCO3 (s) ⇌ CaO (s) + CO2(g)
c) S(s) + 3F2 (g) ⇌ SF6 (g)
In each of the above reaction find out whether you have to increase (or) decrease the volume to increase the yield of the product.
Ans: Δng = 2−2 = 0
a) The number of moles of products is equal to the number of moles of reactants, so change in pressure has no effect on equilibrium.
b) Δng = 1− 0 = 1
Δng = +ve
(ie) To increase the yield of product pressure should be decrease and hence volume increases
c) Δng = 1−3 = −2
Δng = -ve
(ie) pncrease in pressure favours formation of product hence volume should be decreased
36. Derive a general expression for the equilibrium constant KP and KC for the reaction
3H2(g) + N2(g) ⇌ 2NH3(g)
Synthesis of ammonia:
Let us consider the formation of ammonia in which 'a' moles nitrogen and 'b' moles hydrogen gas are allowed to react in a container of volume V. Let 'x' moles of nitrogen react with 3x moles of hydrogen to give 2x moles off ammonia.
N2(g) + 3H2(g) ⇌ 2NH3(g)
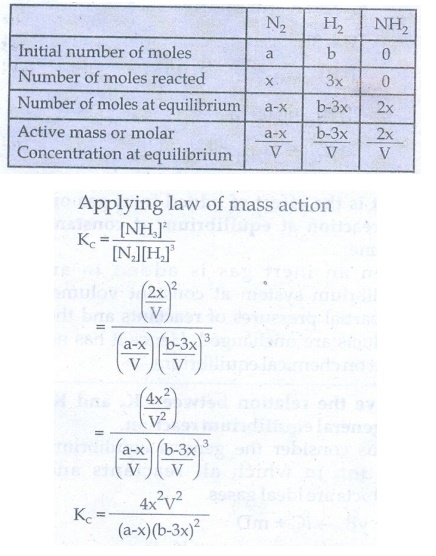
The equilibrium constant Kp can be also be calculated as follows:

37. Write a balanced chemical equation for a equilibrium reaction for which the equilibrium constant is given by expression
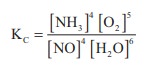
Solution:
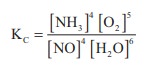
KC = [NH3]4[O2]5 / [NO]4[H2O]6
Chemical equation is
4NO + 6H2O ⇌ 4NH3 +5O2
38. What is the effect of added inert gas on the reaction at equilibrium
When an inert gas is added to an equilibrium system at constant volume, the partial pressures of reactants and the products are unchanged. Hence it has no effect on chemical equilibrium.
39. Derive the relation between KP and KC
Let us consider the general equilibrium reaction in which all reactants and products are ideal gases.
xA + y B → lC + mD
The equilibrium constant KC is
KC = [C]l [D]m / [A]x[B]y …………….(1)
The equilibrium constant KP is
KP = [ (PC)l.(PD)m ] / [ (PA)x.(PB)y ] …………….(2)
From the ideal gas equation
PV = nRT
P = n/V RT
Active mass = molar concentration = n / V
P = active mass × (RT)
PAx = [A]x . [RT] x
PCl = [C] l . [RT] l
PBy = [B]y . [RT]y
PDm = [D]m . [RT]m
on subsituting equation 2
kP = { [C]l.[RT]l [D]m.[RT]m } / { [A]x.[RT] x [B]y.[RT]y } ………. (3)
kP = { [C]l[D]m[RT]l+m } / { [A]x [B]y[RT]x+y }
kP = { [C]l[D]m / [A]x[B]y } [RT]( l + m) - ( x + y) …….(4)
By comparing equation (1) & (4) we get
kP = KC (RT) (Δng) ΔS ……….(5)
Where Δng, is the difference between the sum of number of moles of gaseous products and the sum of the number of moles of gaseous reactants.
40. One mole of PCl5 is heated in one litre closed container. If 0.6 mole of chlorine is found at equilibrium, calculate the value of equilibrium constant.
Solution:
Given that [PCl5]initital = 1 mole / 1 dm3
[Cl2]eq = 0.6 mole dm−3
PCl5 → PCl3 + Cl2
[PCl3]eq = 0.6 mole dm−3
[PCl5]eq = 0.4 mole dm−3
KC = ( [PC13][C12] ) / [PCl5]
= (0.6 × 0.6) / 0.4 = 0.9
KC = 0.9
41. For the reaction
SrCO3 (s) ⇌ SrO (s) + CO2(g),
the value of equilibrium constant KP = 2.2 × 10–4 at 1002 K. Calculate KC for the reaction.
Solution:
For the reaction,
SrCO3(s) ⇌ SrO (s) + CO2(g)
Δng = 1 − 0 = 1
KP = KC (RT)
2.2 × 10-4 = KC (0.0821) (1002)
KC = ( 2.2× 10-4 ) / ( 0.0821× 1002 )
= 0.0267 × 10-4
= 2.67 × 10-6
42. To study the decomposition of hydrogen iodide, a student fills an evacuated 3 litre flask with 0.3 mol of HI gas and allows the reaction to proceed at 500 0C. At equilibrium he found the concentration of HI which is equal to 0.05 M. Calculate KC and KP.
Solution:
V = 3L
[HI]initital = 0.3 / 3 = 0.1M
[HI]eq = 0.05 M
2HI(g) ⇌ H2(g) + I2(g)

KC = [H2] [I2] / [HI]2
= (0.025 ×0.025) / (0.05)2
KP = KC (RT)Δng
Δng = 2−2 = 0
KP = 0.25 (RT)°
∴ KP = 0.25
43. Oxidation of nitrogen monoxide was studied at 200 0C with initial pressures of 1 atm NO and 1 atm of O2. At equilibrium partial pressure of oxygen is found to be 0.52 atm calculate KP value.
Solution:
2NO(g) + O2(g) ⇌ 2NO2(g)
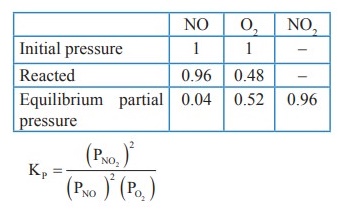
KP = [PNO2]2/ ( [PNO]2[PO2]) = (0.96 × 0.96) / (0.04 × 0.04 × 0.52)
= 1.107 × 103 (atm)−1
44. 1 mol of CH4, 1 mole of CS2 and 2 mol of H2S are 2 mol of H2 are mixed in a 500 ml flask. The equilibrium constant for the reaction KC = 4 × 10–2 mol2 lit–2. In which direction will the reaction proceed to reach equilibrium ?
Solution:
CH4(g) + 2H2S(g) ⇌ CS2(g) + 4H2(g)
KC = 4 ×10−2 mol2lit−2
V = 500 ml = 1/2 L
[CH4]in = 1 mol / (½)L = 2 mol L−1 ;
[CS2]in = 1 mol / (½)L = 2 mol L−1
[H2S]in = 2 mol / (½)L = 4 mol L−1
[H2]in = 2 mol / (½)L = 4 mol L−1
Q = ( [CS2] [H2]4 ) / ( [CH4] [H2S]2 ) = [ 2 × (4)4 ] / [ 2 × (4)2 ] = 16
Q > KC
∴ The reaction will proceed in the reverse direction to reach the equilibrium.
45. At particular temperature KC = 4 × 10–2 for the reaction
H2S(g) ⇌ H2(g) + ½ S2(g)
Calculate KC for each of the following reaction
i) 2H2S (g) ⇌ 2H2 (g) + S2 (g)
ii) 3H2S (g) ⇌ 3H2 (g) + 3/2 S2(g)
Solution:
i) 2H2S(g) ⇌ 2H2(g) + S2(g)
ii) 3H2S(g) ⇌ 3H2(g) + 3/2 S2(g)
KC = 4 × 10−2 for the reaction,
H2S(g) ⇌ H2(g) + ½ S2(g).
KC = ( [H2] [S2]½ ) / [H2S]
⇒ 4 × 10−2 = [H2][S2] ½ / [H2S]
For the reaction,
2H2S(g) ⇌ 2H2(g) + S2(g)
KC = ([H2]2[S2]) / [H2S]2 = (4 × 10−2)2
= 16 × 10-4
For the reaction,
3H2S(g) ⇌ 3H2(g) + 3/2 S2(g)
KC = ([H2]3[S2] 3/2) / [H2S]3 = (4 × 10−2)3
= 64 × 10-6
46. 28 g of Nitrogen and 6 g of hydrogen were mixed in a 1 litre closed container. At equilibrium 17g NH3 was produced. Calculate the weight of nitrogen, hydrogen at equilibrium.
Solution:
Given,
mN2 = 28g; mH2 = 6g;
mNH3 = 17g ; V = 1 L
(nN2)initiaI = 28 / 28 = 1 mol
(nH2)initial = 6 / 2 = 3 mol
N2(g) + 3H2(g) ⇌ 2NH3(g)
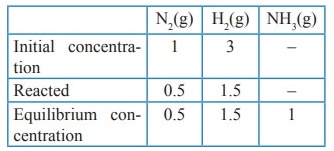
[NH3] = (17 / 17 ) = l mol
Weight of N2 = (no.of moles of N2) × molar mass of N2
= 0.5 × 28 =14g
Weight of H2 = (no.of moles of H2) × molar mass of H2
= 1.5 × 2 = 3g
47. The equilibrium for the dissociation of XY2 is given as,
2XY2 (g) ⇌ 2XY (g) + Y2(g)
if the degree of dissociation x is so small compared to one. Show that 2 KP = PX3 where P is the total pressure and KP is the dissociation equilibrium constant of XY2.
Solution:

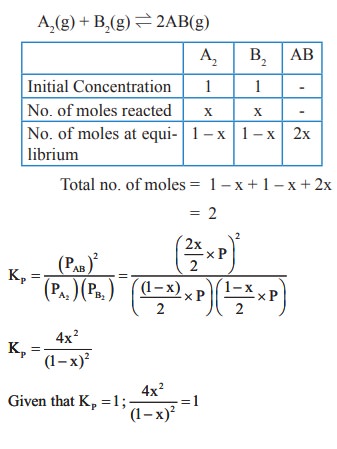
48. A sealed container was filled with 1 mol of A2 (g), 1 mol B2 (g) at 800 K and total pressure 1.00 bar. Calculate the amounts of the components in the mixture at equilibrium given that K = 1 for the reaction
A2 (g) + B2 (g) ⇌ 2AB (g)
Solution:

49. Deduce the Vant Hoff equation.
50. The equilibrium constant KP for the reaction
N2(g) + 3H2(g) ⇌ 2NH3(g) is 8.19 × 102 at 298 K and 4.6 × 10–1 at 498 K. Calculate ΔH0 for the reaction.
Solution:

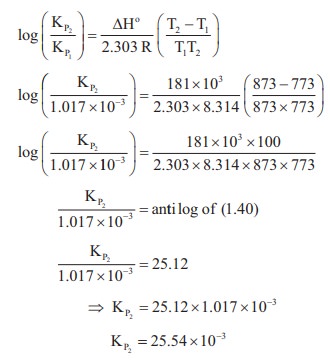
51. The partial pressure of carbon dioxide in the reaction CaCO3 (s) ⇌ CaO (s) + CO2(g) is 1.017 × 10–3 atm at 5000 C. Calculate KP at 6000c C for the reaction. ΔH for the reaction is 181 KJ mol–1 and does not change in the given range of temperature.
Solution:
PCO2 = 1.017x10-3 atm T = 500º C
Kp = PCO2
KP1 = 1.017x10-3 T=500+273=773K
KP2 = ? T=600+273=873K
∆Hº = 181KJmol-1
Related Topics