Particulate Gravimetry: Theory and Practice

Theory and Practice

Two approaches have been used to separate the analyte from its matrix in particu- late gravimetry. The most common approach is filtration, in which solid particu- lates are separated from their gas, liquid, or solid matrix. A second approach uses a liquid-phase or solid-phase extraction.

Filtration 

Liquid samples are filtered by pulling the liquid through an appropriate filtering medium, either by gravity or by applying suction from a vacuum pump or aspirator. The choice of filtering medium is dictated primarily by the size of the solid particles and the sample’s matrix. Filters are constructed from a variety of ma- terials, including cellulose fibers, glass fibers, cellulose nitrate, and polytetrafluo- roethylene (PTFE). Particle retention depends on the size of the filter’s pores. Cellu- lose fiber filters, commonly referred to as filter paper, range in pore size from 30 μm to 2–3 μm. Glass fiber filters, constructed from chemically inert borosilicate glass, range in pore size from 2.5 μm to 0.3 μm. Membrane filters, which are made from a variety of materials, including cellulose nitrate and PTFE, are available with pore sizes from 5.0 μm to 0.1 μm.

Solid aerosol particulates in gas samples are filtered using either a single or multiple stage. In a single-stage system the gas is passed through a single filter, re- taining particles larger than the filter’s pore size. When sampling a gas line, the filter is placed directly in line. Atmospheric gases are sampled with a high-volume sam- pler that uses a vacuum pump to pull air through the filter at a rate of approxi- mately 75 m3/h. In either case, the filtering medium used for liquid samples also can be used for gas samples. In a multiple-stage system, a series of filtering units is used to separate the particles by size.

Solid samples are separated by particle size using one or more sieves. By select- ing several sieves of different mesh size, particulates with a narrow size range can be isolated from the solid matrix. Sieves are available in a variety of mesh sizes, ranging from approximately 25 mm to 40 μm.

Extraction 

Filtering limits particulate gravimetry to solid particulate analytes that are easily separated from their matrix. Particulate gravimetry can be extended to the analysis of gas-phase analytes, solutes, and poorly filterable solids if the analyte can be extracted from its matrix with a suitable solvent. After extraction, the solvent can be evaporated and the mass of the extracted analyte determined. Alternatively, the analyte can be determined indirectly by measuring the change in a sample’s mass after extracting the analyte.

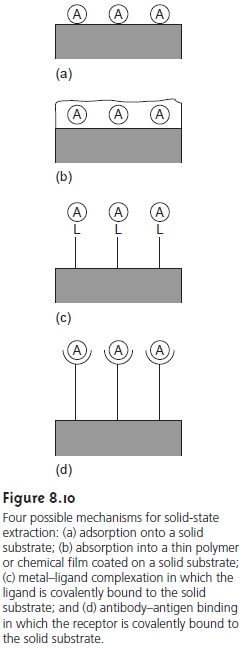
More recently, methods for particulate gravimetry have been developed in which the analyte is separated by adsorption onto a metal surface, by absorption into a thin polymer or chemical film coated on a solid support, or by chemically binding to a suitable receptor covalently bound to a solid support (Figure 8.10). Ad- sorption, absorption, and binding occur at the interface between the metal surface, the thin film, or the receptor, and the solution containing the analyte. Conse- quently, the amount of analyte extracted is minuscule, and the resulting change in mass is too small to detect with a conventional balance. This problem is overcome by using a quartz crystal microbalance as a support.

The measurement of mass using a quartz crystal microbalance is based on the piezoelectric effect.10 When a piezoelectric material, such as a quartz crystal, experi- ences a mechanical stress, it generates an electrical potential whose magnitude is proportional to the applied stress. Conversely, when an alternating electrical field is applied across a quartz crystal, an oscillatory vibrational motion is induced in the crystal. Every quartz crystal vibrates at a characteristic resonant frequency that is a function of the crystal’s properties, including the mass per unit area of any material coated on the crystal’s surface. The change in mass following adsorption, absorption, or binding of the analyte, therefore, can be determined by monitoring the change in the quartz crystal’s characteristic resonant frequency. The exact relationship between the change in frequency and mass is determined by a calibration curve.