Mathematics - First Order Linear Differential Equations | 12th Maths : UNIT 10 : Ordinary Differential Equations
Chapter: 12th Maths : UNIT 10 : Ordinary Differential Equations
First Order Linear Differential Equations
First Order Linear
Differential Equations
A first order
differential equation of the form
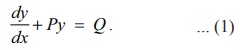
where P and Q are functions of x
only. Here no product of dy/dx and
its derivative dy/dx occur and the dependent
variable y and its derivative with
respect to independent variable x
occurs only in the first degree.
To
integrate (1), let us consider the homogeneous equation dy/dx + Py
=
0 . ….(2)
The
equation (2) can be integrated as follows:
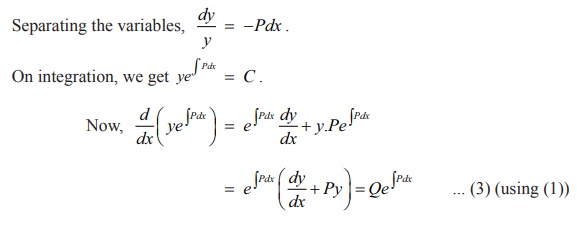
Integrating
both sides of (3) with respect to x,
we get the solution of the given differential equation as
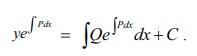
Here e∫Pdx is known as the integrating
factor (I.F.) of (1).
Remarks
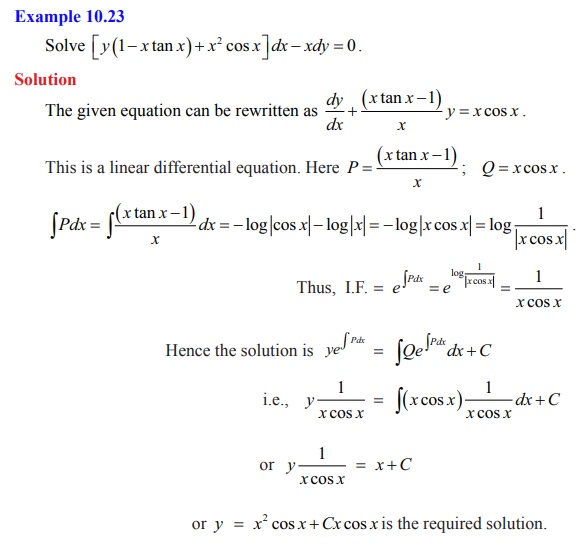
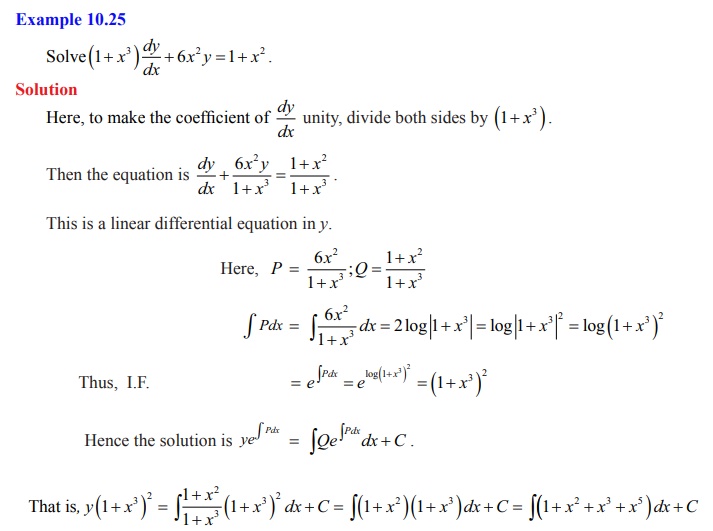
1. The
solution of linear differential equation is
y × (I.F )
= ∫Q(I .F
)dx + C , where C is an arbitrary constant.
2. In
the integrating factor e∫
Pdx , P is the coefficient of y
in the differential equation provided the coefficient of dy/dx is unity.
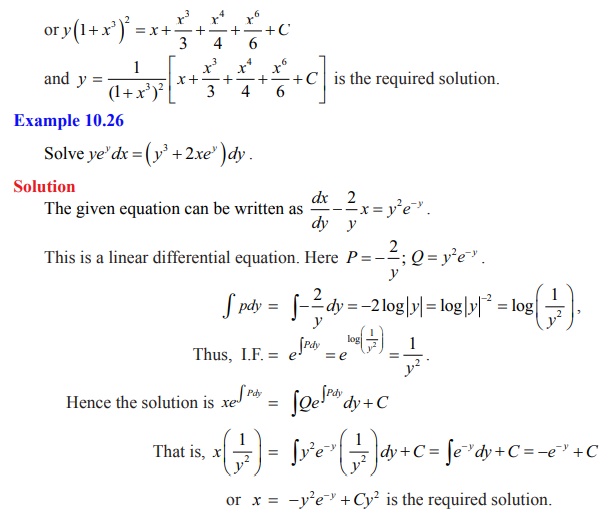
3. A
first order differential equation of the form dx/dy + Px
=
Q , where P and Q are functions of y only. Here no product of x and its derivative dx/dy occur and the dependent variable x and its derivative with respect to
independent variable y occurs only in
the first degree.
In this
case, the solution is given by xe∫
Pdy =
∫Qe∫
Pdy dy + C
.
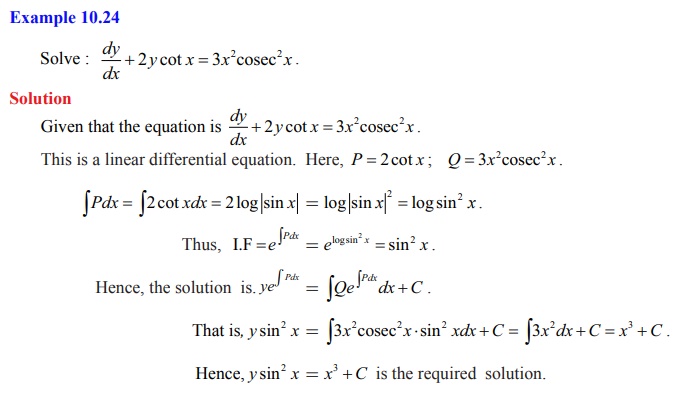
Related Topics