Chapter: 11th 12th std standard Class Organic Inorganic Physical Chemistry Higher secondary school College Notes
Calculation Of Atomic Radius (Covalent Radius)
CALCULATION OF ATOMIC RADIUS (COVALENT RADIUS)
Atomic radius is the distance from the centre of the
nucleus to the point where the electron density is effectively zero.
a. Homonuclear
diatomic molecules
In case of homonuclear diatomic molecules of A2 type (e.g. F2, Cl2, Br2, I2 ... etc.) the bond
length, d(A-A) is given by
d(A - A) = r(A) +
r(A)
d(A - A) = 2 × r(A)
r(A) = d(A-A) / 2
The above equation shows that in the case of homonuclear
diatomic molecule of A2 type, the covalent
radius of an atom A, r(A) is equal to one half of the inter- nuclear
distance, d(A-A). Therefore, the covalent radius of an atom in a homonuclear diatomic molecule can be obtained by
dividing the internuclear distance by
two.
Example
1. Cl2 molecule
The value of Cl-Cl bond distance as found experimentally is 1.98Å. Thus
r(Cl)= d(Cl- Cl) / 2 = 1.98/2 = 0.99Å
2. Diamond
The value of d(C-C) distance as found experimentally in a variety of
saturated hydrocarbons is 1.54Å.
r(C) = d(C - C) / 2 = 1.54 / 2 = 0.77 Å
b. Heteronuclear diatomic molecule
In case of heteronuclear diatomic molecule of AB type,
bond length
d(A - B) is given by
d(A - B) = r(A) + r(B)
r(A) and r(B) are the covalent radii of A and B atoms.
Example
i) CCl4 molecule
The experimental value of d(C - Cl) is 1.76 Å
Thus d(C-Cl) =
r(C) + r(Cl)
r(C) = d(C - Cl)
- r(Cl)
= 1.76 - r(Cl)
Thus the covalent radius of carbon atom can be
calculated by subtracting the covalent radius of Cl atom
from d(C-Cl) bond length. The covalent radius of Cl atom can
also be obtained, provided that covalent radius of C atom is known.
ii) Sic
The experimental value of d(Si-C) is 1.93 Å. Thus,
d(Si - C) = r(Si) +
r(C)
r(Si) = d(Si - C) - r(C) = 1.93 - r(C)
= 1.93 - 0.77 = 1.16 Å
The experimental values of covalent bond length for some common
homonuclear diatomic molecules are given below.
Molecule : Bond
: Bond length (Å)
H2 : H-H : 0.74
F2 : F-F : 1.44
Cl2 : Cl-Cl : 1.98
Br2 : Br-Br : 2.28
H3C-CH3 : C-C : 1.54
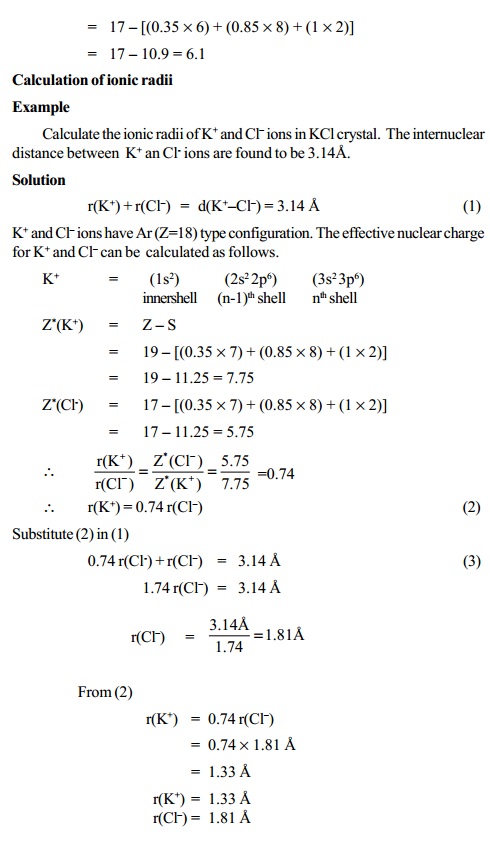
Calculation of ionic radii
Pauling's Method
Pauling has calculated the radii of the ions on the
basis of the observed internuclear distances in four crystals namely NaF, KCl,
RbBr and CsI. In each ionic crystal the cations and anions are isoelectronic
with inert gas configuration.
NaF crystal : Na+ - 2, 8 F- - 2, 8 Ne type configuration
KCl crystal : K+ - 2, 8, 8 Cl- - 2, 8, 8 Ar type configuration
Further the following two
assumptions are made to assign the ionic radii.
i) The cations and anions of an ionic crystal are assumed to be in
contact
with each other and hence the sum of their radii will be equal to the
inter nuclear distance between them.
r(C+) + r(A-) = d (C+-A-)
where
r(C+)
- radius of
cation, C+ r(A-)
-
radius of anion, A-
d(C+-A-)
- internuclear distance between C+ and A- ions in C+A- ionic crystal
ii) For a given noble gas configuration, the radius of
an ion is inversely proportional to its effective nuclear charge. i.e.
r(C+ ) á = 1/ Z (C+ )
r(A- ) á = 1/ Z (A- )
where,
Z*(C+)
& Z*(A-) are the
effective nuclear charges of cation (C+) and anion (A-)
respectively. On combining
r(C+ ) / r(A- ) = Z*(A- ) / Z*(C+ )
Hence the above two equations (1) & (4) can be used
to evaluate the
values of r(C+) and r(A-) provided that the values of d(C+-A-), Z*(C+) and Z*(A-) are known.
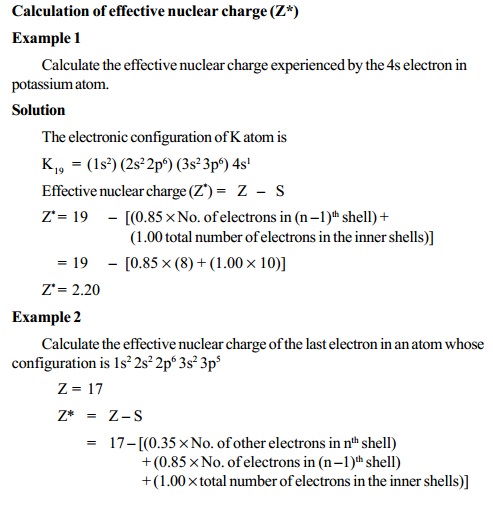
Slater rules
The value of screening constant (S) and effective
nuclear charge (Z*)
can
be calculated by using Slater's rules. According to these rules the
value of "S" for a given electron is estimated as follows.
i) Write down the complete electronic configuration of
the element and divide the electrons into the following orbital groups
starting from the inside of the atom.
(1s) : (2s, 2p) : (3s, 3p) : (3d) : (4s, 4p) :
(4d) : (4f) : (5s, 5p) : (6s, 6p) .......etc.
ii) Select the electron for which the value of S is to be
calculated. For this calculation add up the contributions to S for the other
electrons according to the following rules.
Contribution to S for each electron of this
type
Type of electron
1.
All electrons in groups outside the electron chosen - 0
2.
All other electrons in the same group as the chosen one (n) - 0.35 (or 0.30 for 1s electron)
3.
All electrons in shell immediately inside (n-1) - 0.85
4.
All electrons further inside - 1.00
Ionisation potential
Ionisation energy of an element is defined as the amount of energy
required to remove the most loosely bound electron from isolated neutral
gaseous atom in its lowest energy state. The process is represented as
M(g) + Energy
supplied - I1-- > M+(g) + e-
Ionisation energy is measured in electron volts per atom (eV/atom), kilo
calories
per mole (kcal/mole) or kilo joules per mole (kJ/mole).
Successive ionisation potentials
In addition to first ionisation potential (I1) defined
above, second, third. etc.
ionisation potentials are also known. Second ionisation potential (I2) is the
energy required to remove one more electron from the gaseous
cation, M+(g) to get the
doubly positively charged gaseous cation, M2+(g), i.e.,
M+(g) + I2 --- > M2+(g)
+ e-
Similarly, third ionisation potential (I3) is the
energy required to remove still one more electron
from M2+(g) cation to get M3+(g) cation, i.e.
M2+(g) + I3 - -- > M3+(g)
+ e-
Similarly ionisation potentials of higher and higher grades are also
known.
Each successive ionization potential or energy is greater than the
previous one, since the electron must be removed against the net
positive charge on the ion.
Related Topics