Wave nature of particles: Example Solved Numerical Problems

Wave nature of particles

EXAMPLE 7.6

Calculate the momentum and the de Broglie wavelength in the following cases:

i) an electron with kinetic energy 2 eV.

ii) a bullet of 50 g fired from rifle with a speed of 200 m/s

iii) a 4000 kg car moving along the highways at 50 m/s

Hence show that the wave nature of matter is important at the atomic level but is not really relevant at macroscopic level.

Solution:

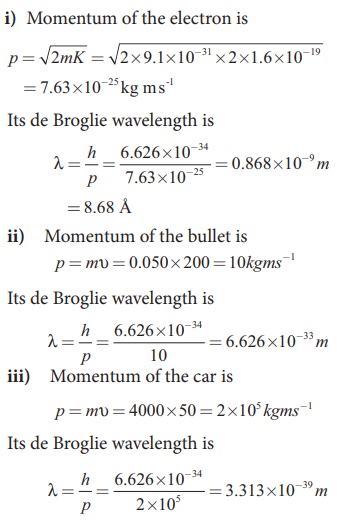
From these calculations, we notice that electron has significant value of de Broglie wavelength (≈10^–9m which can be measured from difraction studies) but bullet and car have negligibly small de Broglie wavelengths associated with them (≈10^–33m and 10^–39m respectively, which are not measurable by any experiment). This implies that the wave nature of matter is important at the atomic level but it is not really relevant at the macroscopic level.

EXAMPLE 7.7

Find the de Broglie wavelength associated with an alpha particle which is accelerated through a potential difference of 400 V. Given that the mass of the proton is 1.67 × 10^–27 kg.

Solution

An alpha particle contains 2 protons and 2 neutrons. Therefore, the mass M of the alpha particle is 4 times that of a proton (m_p) (or a neutron) and its charge q is twice that of a proton (+e).

The de Broglie wavelength associated with it is

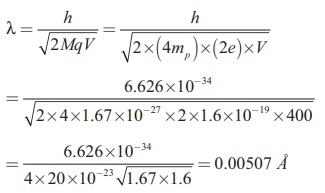
EXAMPLE 7.8

A proton and an electron have same de Broglie wavelength. Which of them moves faster and which possesses more kinetic energy?

Solution

We know that λ = h/ √(2mK)

Since proton and electron have same de Broglie wavelength, we get

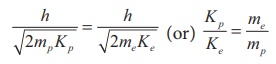
Since m_e < m_p , K_p < K_e , the electron has more kinetic energy than the proton.

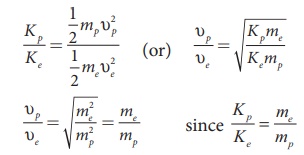
Since m_e < m_p , Ï…_p < Ï…_e , the electron moves faster than the proton.